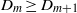The inviscid growth of a range of vorticity moments is compared using Euler calculations of anti-parallel vortices with a new initial condition. The primary goal is to understand the role of nonlinearity in the generation of a new hierarchy of rescaled vorticity moments in Navier–Stokes calculations where the rescaled moments obey ${D}_{m} \geq {D}_{m+ 1} $, the reverse of the usual ${\Omega }_{m+ 1} \geq {\Omega }_{m} $ Hölder ordering of the original moments. Two temporal phases have been identified for the Euler calculations. In the first phase the 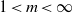$1\lt m\lt \infty $ vorticity moments are ordered in a manner consistent with the new Navier–Stokes hierarchy and grow in a manner that skirts the lower edge of possible singular growth with 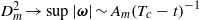${ D}_{m}^{2} \rightarrow \sup \vert \boldsymbol{\omega} \vert \sim A_{m}{({T}_{c} - t)}^{- 1} $ where the 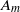${A}_{m} $ are nearly independent of 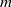$m$. In the second phase, the new ${D}_{m} $ ordering breaks down as the ${\Omega }_{m} $ converge towards the same super-exponential growth for all $m$. The transition is identified using new inequalities for the upper bounds for the $- \mathrm{d} { D}_{m}^{- 2} / \mathrm{d} t$ that are based solely upon the ratios 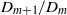${D}_{m+ 1} / {D}_{m} $, and the convergent super-exponential growth is shown by plotting 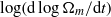$\log (\mathrm{d} \log {\Omega }_{m} / \mathrm{d} t)$. Three-dimensional graphics show significant divergence of the vortex lines during the second phase, which could be what inhibits the initial power-law growth.