Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-01-24T21:51:03.979Z Has data issue: false hasContentIssue false
  • English
  • Français

Boundary-layer-separation-driven vortex shedding beneath internal solitary waves of depression

Published online by Cambridge University Press:  24 November 2011

Payam Aghsaee ,
Leon Boegman ,
Peter J. Diamessis  and
Kevin G. Lamb
Payam Aghsaee
Affiliation:
Environmental Fluid Dynamics Laboratory, Department of Civil Engineering, Queen’s University, Kingston, Ontario, K7L 3N6, Canada
Leon Boegman*
Affiliation:
Environmental Fluid Dynamics Laboratory, Department of Civil Engineering, Queen’s University, Kingston, Ontario, K7L 3N6, Canada
Peter J. Diamessis
Affiliation:
School of Civil and Environmental Engineering, Cornell University, Ithaca, New York, USA
Kevin G. Lamb
Affiliation:
Department of Applied Mathematics, University of Waterloo, Ontario, N2L 3G1, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate global instability and vortex shedding in the separated laminar boundary layer beneath internal solitary waves (ISWs) of depression in a two-layer stratified fluid by performing high-resolution two-dimensional direct numerical simulations. The simulations were conducted with waves propagating over a flat bottom and shoaling over relatively mild and steep 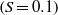slopes. Over a flat bottom, the potential for vortex shedding is shown to be directly dependent on wave amplitude, for a particular stratification, owing to increase of the adverse pressure gradient ( 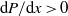for leftward propagating waves) beneath the trailing edge of larger amplitude waves. The generated eddies can ascend from the bottom boundary to as high as 33 % of the total depth in two-dimensional simulations. Over sloping boundaries, global instability occurs beneath all waves as they steepen. For the slopes considered, vortex shedding begins before wave breaking and the vortices, shed from the bottom boundary, can reach the pycnocline, modifying the wave breaking mechanism. Combining the results over flat and sloping boundaries, a unified criterion for vortex shedding in arbitrary two-layer continuous stratifications is proposed, which depends on the momentum-thickness Reynolds number and the non-dimensionalized ISW-induced pressure gradient at the point of separation. The criterion is generalized to a form that may be readily computed from field data and compared to published laboratory experiments and field observations. During vortex shedding events, the bed shear stress, vertical velocity and near-bed Reynolds stress were elevated, in agreement with laboratory observations during re-suspension events, indicating that boundary layer instability is an important mechanism leading to sediment re-suspension.

JFM classification

Boundary Layers: Boundary layer stability Geophysical and Geological Flows: Internal waves Geophysical and Geological Flows: Ocean processes
Type
Papers
Information
Journal of Fluid Mechanics , Volume 690 , 10 January 2012 , pp. 321 - 344
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Aghsaee, P., Boegman, L. & Lamb, K. G. 2010 Breaking of shoaling internal solitary waves. J. Fluid Mech. 659, 289–317.CrossRefGoogle Scholar
2. Apel, J. R. 2002 Oceanic internal waves and solitons. In An Atlas of Oceanic Internal Solitary Waves. Global Ocean Associates.Google Scholar
3. Barad, M. F. & Fringer, O. B. 2010 Simulations of shear instabilities in interfacial gravity waves. J. Fluid Mech. 644, 61–95.CrossRefGoogle Scholar
4. Boegman, L., Imberger, J., Ivey, G. N. & Antenucci, J. P. 2003 High-frequency internal waves in large stratified lakes. Limnol. Oceanogr. 48, 895–919.CrossRefGoogle Scholar
5. Boegman, L. & Ivey, G. N. 2009 Flow separation and resuspension beneath shoaling nonlinear internal waves. J. Geophys. Res. 114, C02018.Google Scholar
6. Bogucki, D. J., Dickey, T. & Redekopp, L. G. 1997 Sediment resuspension and mixing by resonantly generated internal solitary waves. J. Phys. Oceanogr. 27, 1181–1196.2.0.CO;2>CrossRefGoogle Scholar
7. Bogucki, D. J. & Redekopp, L. G. 1999 Global instability of separated flows: a paradigm for sediment resuspension by internal waves. Geophys. Res. Lett. 26, 1317–1320.CrossRefGoogle Scholar
8. Bogucki, D. J., Redekopp, L. G. & Barth, J. 2005 Internal solitary waves in the coastal mixing and optics 1996 experiment: multimodal structure and re-suspension. J. Geophys. Res. 110, C02024.Google Scholar
9. Bonnin, J., Van Haren, H., Hosegood, Ph. & Brummer, G. A. 2006 Burst resuspension of seabed material at the foot of the continental slope in the Rockall Channel. Mar. Geol. 226, 167–184.CrossRefGoogle Scholar
10. Bourassa, C. & Thomas, F. O. 2009 An experimental investigation of a highly accelerated turbulent boundary layer. J. Fluid Mech. 634, 359–404.CrossRefGoogle Scholar
11. Bourgault, D. & Kelley, D. E. 2003 Wave-induced mixing in a partially mixed estuary. J. Mar. Res. 226, 553–576.CrossRefGoogle Scholar
12. Butman, B., Alexander, P. S., Scotti, A., Beardsley, R. C. & Anderson, S. P. 2006 Large internal waves in Massachusetts Bay transport sediments offshore. Cont. Shelf Res. 26, 2029–2049.CrossRefGoogle Scholar
13. Carr, M. & Davies, P. A. 2006 The motion of an internal solitary wave of depression over a fixed bottom boundary in a shallow, two layer fluid. Phys. Fluids 18, 016601.CrossRefGoogle Scholar
14. Carr, M., Davies, P. A. & Shivaram, P. 2008 Experimental evidence of internal solitary wave-induced global instability in shallow water benthic boundary layers. Phys. Fluids 20, 066603.CrossRefGoogle Scholar
15. Carr, M., Stastna, M. & Davies, P. A. 2010 Internal solitary wave-induced flow over a corrugated bed. Ocean Dyn. 60, 1007–1025.CrossRefGoogle Scholar
16. Diamessis, P. & Redekopp, L. G. 2006 Numerical investigation of solitary internal wave-induced global instability in shallow water benthic boundary layers. J. Phys. Oceanogr. 36, 784–812.CrossRefGoogle Scholar
17. Drake, D. E. & Cacchione, D. A. 1986 Field observations of bed shear stress and sediment resuspension on continental shelves, Alaska and California. Cont. Shelf Res. 6, 3, 415–429.CrossRefGoogle Scholar
18. Gaster, M. 1969 The structure and behaviour of laminar separation bubbles. AGARD CP 4, 813–854.Google Scholar
19. Helfrich, K. R. & Melville, W. K. 1986 On long nonlinear internal waves over slope-shelf topography. J. Fluid Mech. 167, 285–308.CrossRefGoogle Scholar
20. Helfrich, K. R. & Melville, W. K. 2006 Long nonlinear internal waves. Annu. Rev. Fluid Mech. 38, 395–425.CrossRefGoogle Scholar
21. Holloway, P. E. 1987 Internal hydraulic jumps and solitons at a shelf break region on the Australian North West Shelf. J. Geophys. Res. 92, 5405–5416.Google Scholar
22. Hosegood, H. & van Haren, 2004 Near-bed Solibores over the continental slope in the Faeroe–Shetland Channel. Deep-Sea Res. II 51, 2943–2971.Google Scholar
23. Huerre, P. 2000 Open shear flow instabilities. In Perspectives in Fluid Dynamics (ed. Batchelor, G. K., Moffatt, H. K. & Worster, M. G. ), pp. 159–229. Cambridge University Press.Google Scholar
24. Jackson, C. 2007 Internal wave detection using the Moderate Resolution Imaging Spectroradiometer. J. Geophys. Res. 112, C11012.Google Scholar
25. Jones, L. E., Sandberg, R. D. & Sandham, N. D. 2008 Direct numerical simulations of forced and unforced separation bubbles on an aerofoil at incidence. J. Fluid Mech. 602, 175–207.CrossRefGoogle Scholar
26. Klymak, J. M. & Moum, J. N. 2003 Internal solitary waves of elevation advancing on a shoaling shelf. Geophys. Res. Lett. 30 (20), 2045.CrossRefGoogle Scholar
27. Koop, C. G. & Butler, G. 1981 An investigation of internal solitary waves in a two-fluid system. J. Fluid Mech. 112, 225–251.CrossRefGoogle Scholar
28. Lamb, K. G. 2002 A numerical investigation of solitary internal waves with trapped cores formed via shoaling. J. Fluid Mech. 451, 109–144.CrossRefGoogle Scholar
29. Lamb, K. G., Boegman, L. & Ivey, G. N. 2005 Numerical simulations of shoaling internal solitary waves in tilting tank experiments. In Proc. 9th European Workshop on Physical Processes in Natural Waters. Lancaster University, pp. 31–38.Google Scholar
30. Lamb, K. G. & Nguyen, V. T. 2009 Calculating energy flux in internal solitary waves with an application to reflectance. J. Phys. Oceanogr. 39, 559–580.CrossRefGoogle Scholar
31. Lamb, K. G. & Wan, B. 1998 Conjugate flows and flat solitary waves for a continuously stratified fluid. Phys. Fluids 10, 2061–2079.CrossRefGoogle Scholar
32. Lemckert, C., Antenucci, J., Saggio, A. & Imberger, J 2004 Physical properties of turbulent benthic boundary layers generated by internal waves. J. Hydraul. Engng ASCE 130, 58–69.CrossRefGoogle Scholar
33. Liu, A. K., Chang, Y. S., Hsu, M.-K. & Liang, N. K. 1998 Evolution of nonlinear internal waves in the East and South China seas: advances in Oceanography and Sea Ice Research: using ERS observations. J. Geophys. Res. 103, 7995–8008.CrossRefGoogle Scholar
34. Michallet, H. & Ivey, G. N. 1999 Experiments on mixing due to internal solitary waves breaking on uniform slopes. J. Geophys. Res. 104, 13467–13477.CrossRefGoogle Scholar
35. Moum, J. N., Farmer, D. M., Smyth, W. D., Armi, L. & Vagle, S. 2003 Structure and generation of turbulence at interfaces strained by internal solitary waves propagating shoreward over the continental shelf. J. Phys. Oceanogr. 33, 2093–2112.2.0.CO;2>CrossRefGoogle Scholar
36. Nimmo Smith, W. A. M., Katz, J. & Osborn, T. R. 2005 On the structure of turbulence in the bottom boundary layer of the coastal ocean. J. Phys. Oceanogr. 35, 72–93.CrossRefGoogle Scholar
37. Orr, M. H. & Mignerey, P. C. 2003 Nonlinear internal waves in the South China Sea: observation of the conversion of depression internal waves to elevation internal waves. J. Geophys. Res. 108 (C3), 3064.Google Scholar
38. Ostrovsky, L. A. & Stepanyants, Y. A. 2005 Internal solitons in laboratory experiments: comparison with theoretical models. Chaos 15, 037111:1–037111:28.CrossRefGoogle ScholarPubMed
39. Pauley, L. L., Moin, P. & Reynolds, W. C. 1990 The structure of two-dimensional separation. J. Fluid Mech. 220, 397–411.CrossRefGoogle Scholar
40. Quaresma, L. S., Vitorino, J., Oliveira, A. & da Silva, J. 2007 Evidence of sediment resuspension by nonlinear internal waves on the western Portuguese mid-shelf. Mar. Geol. 246, 123–143.CrossRefGoogle Scholar
41. Reeder, D. B., Ma, B. B. & Yang, Y. J. 2011 Very large subaqueous sand dunes on the upper continental slope in the South China Sea generated by episodic, shoaling deep-water internal solitary waves. Mar. Geol. 279, 12–18.CrossRefGoogle Scholar
42. Schlichting, H. 1979 Boundary-layer Theory, 7th edn. McGraw-Hill.Google Scholar
43. Shroyer, E. L., Moum, J. N. & Nash, J. D. 2009 Observations of polarity reversal in shoaling nonlinear internal waves. J. Phys. Oceanogr. 39, 691–701.CrossRefGoogle Scholar
44. Stastna, M. & Lamb, K. G. 2002 Vortex shedding and sediment resuspension associated with the interaction of an internal solitary wave and the bottom boundary layer. Geophys. Res. Lett. 29 (0) doi:10.1029/2001GL014070.CrossRefGoogle Scholar
45. Stastna, M. & Lamb, K. G. 2008 Sediment resuspension mechanisms associated with internal waves in coastal waters. J. Geophys. Res. 113, C10016.Google Scholar
46. Stefanakis, Themistoklis 2010 Bottom boundary layer instabilities induced by nonlinear internal waves. MSc thesis. School of Civil and Environmental Engineering, Cornell University.Google Scholar
47. Sveen, J. K., Guo, Y., Davies, P. A. & Grue, J. 2002 On the breaking of internal solitary waves at a ridge. J. Fluid Mech. 469, 161–188.CrossRefGoogle Scholar
48. Vlasenko, V., Brandt, P. & Rubino, A. 2000 Structure of large-amplitude internal solitary waves. J. Phys. Oceanogr. 30, 2172–2185.2.0.CO;2>CrossRefGoogle Scholar
49. Wang, B. J., Bogucki, D. J. & Redekopp, L. G. 2001 Internal solitary waves in a structured thermocline with implications for resuspension and the formation of thin particle-laden layers. J. Geophys. Res. 106, 9565–9585.CrossRefGoogle Scholar
50. Warn-Varnas, A. C., Chin-Bing, S. A., King, D. B., Hawkins, J. A., Lamb, K. G. & Lynch, J. F. 2007 Winter PRIMER ocean-acoustic solitary wave modelling studies. IEEE J. Ocean. Engng 32, 436–452.CrossRefGoogle Scholar