Hostname: page-component-848d4c4894-xm8r8 Total loading time: 0 Render date: 2024-07-03T06:58:37.173Z Has data issue: false hasContentIssue false
  • English
  • Français

Boundary layer transition mechanisms behind a micro-ramp

Published online by Cambridge University Press:  14 March 2016

Qingqing Ye ,
Ferry F. J. Schrijer  and
Fulvio Scarano
Qingqing Ye*
Affiliation:
Faculty of Aerospace Engineering, Delft University of Technology, Kluyverweg 1, 2629HS, Delft, The Netherlands
Ferry F. J. Schrijer
Affiliation:
Faculty of Aerospace Engineering, Delft University of Technology, Kluyverweg 1, 2629HS, Delft, The Netherlands
Fulvio Scarano
Affiliation:
Faculty of Aerospace Engineering, Delft University of Technology, Kluyverweg 1, 2629HS, Delft, The Netherlands
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The early stage of three-dimensional laminar-to-turbulent transition behind a micro-ramp is studied in the incompressible regime using tomographic particle image velocimetry. Experiments are conducted at supercritical micro-ramp height $h$ based Reynolds number 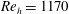$Re_{h}=1170$. The measurement domain encompasses 6 ramp widths spanwise and 73 ramp heights streamwise. The mean flow topology reveals the underlying vortex structure of the wake flow with multiple pairs of streamwise counter-rotating vortices visualized by streamwise vorticity. The primary pair generates a vigorous upwash motion in the symmetry plane with a pronounced momentum deficit. A secondary vortex pair is induced closer to the wall. The tertiary and even further vortices maintain a streamwise orientation, but are produced progressively outwards of the secondary pair and follow a wedge-type pattern. The instantaneous flow pattern reveals that the earliest unstable mode of the wake features arc-like Kelvin–Helmholtz (K–H) vortices in the separated shear layer. Under the influence of the K–H vortices, the wake exhibits a high level of fluctuations with a pulsatile mode for the streamwise momentum deficit. The K–H vortices are lifted up due to the upwash induced by the quasi-streamwise vortex pair, while they appear to undergo pairing, distortion and finally breakdown. Immediately downstream, a streamwise interval of relatively low vortical activity separates the end of the K–H region from the formation of new hairpin vortices close to the wall. The latter vortex structures originate from the region of maximum wall shear, induced by the secondary vortex pair causing strong ejection events which transport low-speed flow upwards. The whole pattern features a cascade of hairpin vortices along a turbulent/non-turbulent interface. The wedge-shaped cascade signifies the formation of a turbulent wedge. The turbulent properties of the wake are inspected with the spatial distribution of the velocity fluctuations and turbulence production in the developing boundary layer. Inside the wedge region, the velocity fluctuations approach quasi-spanwise homogeneity, indicating the development towards a turbulent boundary layer. The wedge interface is characterized by a localized higher level of velocity fluctuations and turbulence production, associated to the deflection of the shear layer close to the wall and the onset of coherent hairpin vortices inducing localized large-scale ejections.

JFM classification

Boundary Layers: Boundary Layers Instability: Instability Instability: Transition to turbulence
Type
Papers
Information
Journal of Fluid Mechanics , Volume 793 , 25 April 2016 , pp. 132 - 161
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Acarlar, M. S. & Smith, C. R. 1987 A study of hairpin vortices in a laminar boundary layer. Part 1. Hairpin vortices generated by a hemispherical protuberance. J. Fluid Mech. 175, 1–41.Google Scholar
Adrian, R. J. & Liu, Z. C. 2002 Observation of vortex packets in direct numerical simulation of fully turbulent channel flow. J. Vis. 5, 9–19.Google Scholar
Adrian, R. J., Meinhart, C. D. & Tomkins, C. D. 2000 Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 422, 1–54.Google Scholar
Anderson, B. H., Tinapple, J. & Surber, L. 2006 Optimal control of shock wave turbulent boundary layer interactions using micro-array actuation. AIAA Paper 2006-3197.Google Scholar
Andersson, P., Berggren, M. & Henningson, D. S. 1999 Optimal disturbances and bypass transition in boundary layers. Phys. Fluids 11, 134–150.Google Scholar
Atkinson, C. & Soria, J. 2009 An efficient simultaneous reconstruction technique for tomographic particle image velocimetry. Exp. Fluids 47, 553–568.Google Scholar
Babinsky, H., Li, Y. & PittFord, C. W. 2009 Microramp control of supersonic oblique shock-wave/boundary-layer interactions. AIAA J. 47, 668–675.Google Scholar
Bernardini, M., Pirozzoli, S. & Orlandi, P. 2012 Compressibility effects on roughness-induced boundary layer transition. Intl J. Heat Fluid Flow 35, 45–51.Google Scholar
Blinde, P. L., Humble, R. A., van Oudheusden, B. W. & Scarano, F. 2009 Effects of micro-ramps on a shock wave/turbulent boundary layer interaction. Shock Waves 19, 507–520.Google Scholar
Brinkerhoff, J. R. & Yaras, M. I. 2014 Numerical investigation of the generation and growth of coherent flow structures in a triggered turbulent spot. J. Fluid Mech. 759, 257–294.Google Scholar
Choudhari, M., Li, F. & Edwards, J. 2009 Stability analysis of roughness array wake in a high-speed boundary layer. AIAA Paper 2009-0170.Google Scholar
Choudhari, M., Li, F., Wu, M., Chang, C.-L., Edwards, J., Kegerise, M. & King, R. 2010 Laminar-turbulent transition behind discrete roughness elements in a high-speed boundary layer. AIAA Paper 2010-1575.Google Scholar
Citro, V., Giannetti, F., Luchini, P. & Auteri, F. 2015 Global stability and sensitivity analysis of boundary-layer flows past a hemispherical roughness element. Phys. Fluids 27, 1–15.Google Scholar
Crow, S. C. 1970 Stability theory for a pair of trailing vortices. AIAA J. 8, 2172–2179.Google Scholar
von Doenhoff, A. E. & Braslow, A. L. 1961 The effect of distributed surface roughness on laminar flow. In Boundary Layer and Flow Control (ed. Lachmann, G. V.), pp. 657–681. Pergamon.Google Scholar
Dolling, D. S. 2001 Fifty years of shock-wave/boundary-layer interaction research: what next? AIAA J. 39, 1517–1531.Google Scholar
van Driest, E. R. & McCauley, W. D. 1960 The effect of controlled three-dimensional roughness on boundary-layer transition at supersonic speeds. J. Aerosp. Sci. 27, 261–271.Google Scholar
Dryden, H. L. 1953 Review of published data on the effect of roughness on transition from laminar to turbulent flow. J. Aeronaut. Sci. 20, 477–482.Google Scholar
Durbin, P. & Wu, X. 2007 Transition beneath vortical disturbances. Annu. Rev. Fluid Mech. 39, 107–128.Google Scholar
Elsinga, G. E., Scarano, F., Wieneke, B. & van Oudheusden, B. W. 2006 Tomographic particle image velocimetry. Exp. Fluids 41, 933–947.Google Scholar
Elsinga, G. E. & Westerweel, J. 2012 Tomographic-piv measurement of the flow around a zigzag boundary layer trip. Exp. Fluids 52, 865–876.Google Scholar
Ergin, F. G. & White, E. B. 2006 Unsteady and transitional flows behind roughness elements. AIAA J. 44, 2504–2514.Google Scholar
Fransson, J. H. M., Brandt, L., Talamelli, A. & Cossu, C. 2004 Experimental and theoretical investigation of the nonmodal growth of steady streaks in a at plate boundary layer. Phys. Fluids 16, 3627–3638.Google Scholar
Fukuda, M. K., Hingst, W. G. & Reshotko, E.1975 Control of shock wave-boundary layer interactions by bleed in supersonic mixed compression inlets. NASA Tech. Rep. 2595, CR.Google Scholar
Ghosh, S., Choi, J.-I. & Edwards, J. R. 2010 Numerical simulations of effects of micro vortex generators using immersed-boundary methods. AIAA J. 48, 92–103.Google Scholar
Giepman, R. H. M., Schrijer, F. F. J. & van Oudheusden, B. W. 2014 Flow control of an oblique shock wave reflection with micro-ramp vortex generators: effects of location and size. Phys. Fluids 26, 1–16.Google Scholar
Guezennec, Y. G., Piomelli, U. & Kim, J. 1989 On the shape and dynamics of wall structures in turbulent channel flow. Phys. Fluids A 1, 764–766.Google Scholar
Herges, T., Kroeker, E., Elliott, G. & Dutton, C. 2010 Microramp flow control of normal shock/boundary-layer interactions. AIAA J. 48, 2529–2542.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Kachanov, Y. S. 1994 Physical mechanisms of laminar-boundary-layer transition. Annu. Rev. Fluid Mech. 26, 411–482.Google Scholar
Kaehler, C. J., Scharnowski, S. & Cierpka, C. 2012 On the resolution limit of digital particle image velocimetry. Exp. Fluids 52, 1629–1639.Google Scholar
Kegerise, M., King, R., Owens, L., Choudhari, M., Norris, A., Li, F. & Chang, C.-L.2012 An experimental and numerical study of roughness-induced instabilities in a Mach 3.5 boundary layer. Tech. Rep. NATO RTO AVT.Google Scholar
Klebanoff, P. S.1955 Characteristics of turbulence in a boundary layer with zero pressure gradient. NACA Tech. Rep. 1247.Google Scholar
Klebanoff, P. S., Cleveland, W. G. & Tidstrom, K. D. 1992 On the evolution of a turbulent boundary layer induced by a three-dimensional roughness element. J. Fluid Mech. 237, 101–187.Google Scholar
Klebanoff, P. S., Schubauerand, G. B. & Tidstrom, K. D. 1955 Measurements of the effect of two-dimensional and three-dimensional roughness elements on boundary-layer transition. J. Aeronaut. Sci. 22, 803–804.Google Scholar
Kline, S. J., Coles, D. E. & Hirst, E. A. 1969 Computation of turbulent boundary layers-1968 AFOSR-IFP-Stanford Conference: proceedings. In Conference on Computation of Turbulent Boundary Layers, Stanford University.Google Scholar
Kundu, P. K. & Cohen, I. M. 2002 Fluid Mechanics, 2nd edn. Academic.Google Scholar
Lee, S., Goettke, M. K., Loth, E., Tinapple, J. & Benek, J. 2010 Microramps upstreams of an oblique-shock/boundary-layer interaction. AIAA J. 48, 104–118.Google Scholar
Li, Q. & Liu, C. 2010 Les for supersonic ramp control flow using mvg at $m=2.5$ and $Re_{{\it\theta}}=1440$ . AIAA Paper 2010-592.Google Scholar
Li, Q. & Liu, C. 2011 Implicit les for supersonic microramp vortex generator: new discoveries and new mechanisms. Model. Simul. Engng 2011, 1–15.Google Scholar
Lin, J. C. 2002 Review of research on low-profile vortex generators to control boundary-layer separation. Prog. Aerosp. Sci. 38, 389–420.Google Scholar
Lin, N., Reed, H. L. & Saric, W. S. 1992 Effect of leading-edge geometry on boundary-layer receptivity to freestream sound. In Instability, Transition and Turbulence (ed. Hussaini, M. Y., Kumar, A. & Streett, C. L.), pp. 421–440. Springer.Google Scholar
Loiseau, J. C., Robinet, J. C., Cherubini, S. & Emmanuel, L. 2014 Investigation of the roughness-induced transition: global stability analyses and direct numerical simulations. J. Fluid Mech. 760, 175–211.CrossRefGoogle Scholar
Lu, F. K., Pierce, A. J. & Shih, Y. 2010 Experimental study of near wake of micro vortex generators in supersonic flow. AIAA Paper 2010-4623.Google Scholar
Lynch, K. P. & Scarano, F. 2015 An efficient and accurate approach to mte-mart for time-resolved tomographic piv. Exp. Fluids 56:66, 1–16.Google Scholar
Ovchinnikov, V., Choudhari, M. M. & Piomelli, U. 2008 Numerical simulation of boundary-layer bypass transition due to high-amplitude free-stream turbulence. J. Fluid Mech. 613, 135–169.Google Scholar
Prasad, A. K. & Jensen, K. 1995 Scheimpflug stereocamera for particle image velocimetry in liquid flows. Appl. Opt. 34, 7092–7099.Google Scholar
Redford, J. A., Sandham, N. D. & Roberts, G. T. 2010 Compressibility effects on boundary-layer transition induced by an isolated roughness element. AIAA J. 48, 2818–2830.Google Scholar
Rizzetta, D. P. & Visbal, M. R. 2007 Direct numerical simulations of flow past an array of distributed roughness elements. AIAA J. 45, 1967–1976.Google Scholar
Robinson, S. K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23, 601–639.Google Scholar
van Rooij, R. P. J. O. M. & Timmer, W. A. 2003 Roughness sensitivity considerations for thick rotor blade airfoils. Trans. ASME: J. Sol. Energy 125, 468–478.Google Scholar
Scarano, F. 2013 Tomographic piv: principles and practice. Meas. Sci. Technol. 24, 1–28.Google Scholar
Schubauer, G. B. & Klebanoff, P. S.1956 Contributions on the mechanics of boundary-layer transition. NACA Tech. Rep. 1289.Google Scholar
Singer, B. A. 1996 Characteristics of a young turbulent spot. Phys. Fluids 8, 509–521.Google Scholar
Smith, A. & Clutter, D. W. 1959 The smallest height of roughness capable of affecting boundary-layer transition. J. Aerosp. Sci. 26, 229–245.Google Scholar
Spalart, P. R. 1988 Direct simulation of a turbulent boundary layer up to $Re_{{\it\theta}}=1410$ . J. Fluid Mech. 187, 61–98.Google Scholar
Sun, Z., Scarano, F., van Oudheusden, B. W., Schrijer, F. F. J., Yan, Y. & Liu, C. 2014a Numerical and experimental investigations of the supersonic microramp wake. AIAA J. 52, 1518–1527.Google Scholar
Sun, Z., Schrijer, F. F. J., Scarano, F. & van Oudheusden, B. W. 2012 The three-dimensional flow organization past a micro-ramp in a supersonic boundary layer. Phys. Fluids 24, 1–22.Google Scholar
Sun, Z., Schrijer, F. F. J., Scarano, F. & van Oudheusden, B. W. 2014b Decay of the supersonic turbulent wakes from micro-ramps. Phys. Fluids 26, 1–21.Google Scholar
Tani, I. 1969 Boundary-layer transition. Annu. Rev. Fluid Mech. 1, 169–196.Google Scholar
Tirtey, S. C., Chazot, O. & Walpot, L. 2011 Characterization of hypersonic roughness-induced boundary-layer transition. Exp. Fluids 50, 407–418.Google Scholar
de Tullio, N.2013 Receptivity and transition to turbulence of supersonic boundary layers with surface roughness. PhD thesis, University of Southampton.Google Scholar
Wang, B., Liu, W., Zhao, Y., Fan, X. & Wang, C. 2012 Experimental investigation of the micro-ramp based shock wave and turbulent boundary layer interaction control. Phys. Fluids 24, 1–14.Google Scholar
Westerweel, J. & Scarano, F. 2005 Universal outlier detection for piv data. Exp. Fluids 39, 1096–1100.Google Scholar
White, F. M. 1991 Viscous Fluid Flow, 2nd edn, pp. 233–240. McGraw-Hill.Google Scholar
Wieneke, B. 2008 Volume self-calibration for 3d particle image velocimetry. Exp. Fluids 45, 549–556.Google Scholar
Wu, X. & Moin, P. 2009 Direct numerical simulation of turbulence in a nominally zero-pressure-gradient at-plate boundary layer. J. Fluid Mech. 630, 5–41.Google Scholar
Yan, Y., Chen, C., Wang, X. & Liu, C. 2014 Les and analyses on the vortex structure behind supersonic mvg with turbulent inflow. Appl. Math. Model. 38, 196–211.Google Scholar
Zhou, J., Adrian, R. J., Balachandar, S. & Kendall, T. M. 1999 Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 387, 353–396.Google Scholar

Ye et al. supplemnetary movie

The instantaneous flow pattern detected by _λ2 criterion, color coded by u/u∞, perspective view.

Download Ye et al. supplemnetary movie(Video)
Video 2.5 MB

Ye et al. supplementary movie

The instantaneous flow pattern detected by _λ2criterion, color coded by u/u∞, side view.

Download Ye et al. supplementary movie(Video)
Video 2.6 MB

Ye et al. supplementary movie

Instantaneous flow pattern at the wedge region. Vortices visualized by iso-surfaces of λ2, color coded by u/u∞; low-speed regions (light blue u’ = -0.13u∞) and high-speed regions (dark blue u’ = 0.1u∞).

Download Ye et al. supplementary movie(Video)
Video 2.9 MB