Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-27T10:56:22.040Z Has data issue: false hasContentIssue false
  • English
  • Français

Bifurcation scenario for a two-dimensional static airfoil exhibiting trailing edge stall

Published online by Cambridge University Press:  04 October 2021

Denis Busquet Open the ORCID record for Denis Busquet [Opens in a new window] ,
Olivier Marquet Open the ORCID record for Olivier Marquet [Opens in a new window] ,
François Richez Open the ORCID record for François Richez [Opens in a new window] ,
Matthew Juniper Open the ORCID record for Matthew Juniper [Opens in a new window]  and
Denis Sipp Open the ORCID record for Denis Sipp [Opens in a new window]
Denis Busquet*
Affiliation:
DAAA, ONERA, Université Paris Saclay, F-92190 Meudon, France Engineering Department, University of Cambridge, Trumpington Street, Cambridge CB2 1PZ, UK
Olivier Marquet
Affiliation:
DAAA, ONERA, Université Paris Saclay, F-92190 Meudon, France
François Richez
Affiliation:
DAAA, ONERA, Université Paris Saclay, F-92190 Meudon, France
Matthew Juniper
Affiliation:
Engineering Department, University of Cambridge, Trumpington Street, Cambridge CB2 1PZ, UK
Denis Sipp
Affiliation:
DAAA, ONERA, Université Paris Saclay, F-92190 Meudon, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

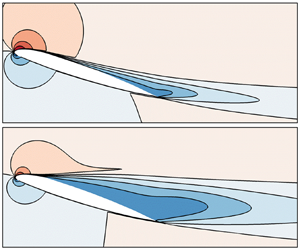
We numerically investigate stalling flow around a static airfoil at high Reynolds numbers using the Reynolds-averaged Navier–Stokes equations (RANS) closed with the Spalart–Allmaras turbulence model. An arclength continuation method allows to identify three branches of steady solutions, which form a characteristic inverted S-shaped curve as the angle of attack is varied. Global stability analyses of these steady solutions reveal the existence of two unstable modes: a low-frequency mode, which is unstable for angles of attack in the stall region, and a high-frequency vortex shedding mode, which is unstable at larger angles of attack. The low-frequency stall mode bifurcates several times along the three steady solutions: there are two Hopf bifurcations, two solutions with a two-fold degenerate eigenvalue and two saddle-node bifurcations. This low-frequency mode induces a cyclic flow separation and reattachment along the airfoil. Unsteady simulations of the RANS equations confirm the existence of large-amplitude low-frequency periodic solutions that oscillate around the three steady solutions in phase space. An analysis of the periodic solutions in the phase space shows that, when decreasing the angle of attack, the low-frequency periodic solution collides with the unstable steady middle-branch solution and thus disappears via a homoclinic bifurcation of periodic orbits. Finally, a one-equation nonlinear stall model is introduced to reveal that the disappearance of the limit cycle, when increasing the angle of attack, is due to a saddle-node bifurcation of periodic orbits.

JFM classification

Instability: Nonlinear instability Nonlinear Dynamical Systems: Bifurcation Turbulent Flows: Turbulent boundary layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 928 , 10 December 2021 , A3
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Akervik, E., Brandt, L., Henningson, D.S., Hoepffner, J., Marxen, O. & Schlatter, P. 2006 Steady solutions of the Navier–Stokes equations by selective frequency damping. Phys. Fluids 18, 068102.CrossRefGoogle Scholar
Alferez, N., Mary, I. & Lamballais, E. 2013 Study of stall development around an airfoil by means of high fidelity large eddy simulation. Flow Turbul. Combust. 91 (3), 623–641.CrossRefGoogle Scholar
Almutairi, J. & AlQadi, I. 2013 Large-eddy simulation of natural low-frequency oscillations of separating–reattaching flow near stall conditions. AIAA J. 51 (4), 981–991.CrossRefGoogle Scholar
Almutairi, J., AlQadi, I. & ElJack, E.M. 2015 Large eddy simulation of a NACA-0012 airfoil near stall. In Direct and Large-Eddy Simulation IX, ERCOFTAC Series (ed. J. Fröhlich, H. Kuerten, B. Geurts & V. Armenio), vol. 20. Springer.CrossRefGoogle Scholar
AlMutairi, J., ElJack, E. & AlQadi, I. 2017 Dynamics of laminar separation bubble over NACA-0012 airfoil near stall conditions. Aerosp. Sci. Technol. 68, 193–203.CrossRefGoogle Scholar
Almutairi, J., Jones, L.E. & Sandham, N.D. 2010 Intermittent bursting of a laminar separation bubble on an airfoil. AIAA J. 48 (2), 414–426.CrossRefGoogle Scholar
Amestoy, P., Duff, I., L'Excellent, J.-Y. & Koster, J. 2001 A fully asynchronous multifrontal solver using distributed dynamic scheduling. SIAM J. Matrix Anal. Applics. 23 (1), 15–41.CrossRefGoogle Scholar
An, H.B., Wen, J. & Feng, T. 2011 On finite difference approximation of a matrix-vector product in the Jacobian-free Newton-Krylov method. J. Comput. Appl. Maths 236, 1399–1409.CrossRefGoogle Scholar
Ansell, P.J. & Bragg, M.B. 2016 Unsteady modes in flowfield about airfoil with horn-ice shape. J. Aircraft 53 (2), 475–486.CrossRefGoogle Scholar
Beneddine, S. 2014 Global stability analysis of underexpanded screeching jets. Eur. J. Mech. (B/Fluids) 49, 392–399.CrossRefGoogle Scholar
Beneddine, S. 2017 Characterization of unsteady flow behavior by linear stability analysis. PhD thesis, Ecole Polytechnique.Google Scholar
Bernardos, L., Richez, F., Gleize, V. & Gerolymos, G.A. 2019 Algebraic nonlocal transition modeling of laminar separation bubbles using $k-\omega$ turbulence models. AIAA J. 57 (2), 553–565.CrossRefGoogle Scholar
Bertagnolio, F, Sørensen, N.N & Rasmussen, F 2005 New insight into the flow around a wind turbine airfoil section. Trans. ASME: J. Sol. Energy Engng 127 (2), 214–222.Google Scholar
Bonne, N. 2018 Stabilité de l'interaction onde de choc/couche limite laminaire. PhD thesis, Université Paris-Saclay – Ecole Polytechnique.Google Scholar
Bragg, M.B. & Zaman, K.B.M.Q. 1994 Flow oscillation over airfoils near stall. Intl Council Aeronaut. Sci. 4 (5), 1639–1648.Google Scholar
Broeren, A.P. & Bragg, M.B. 1998 Low frequency flowfield unsteadiness during airfoil stall and the influence of stall type. AIAA Paper 98-2517.CrossRefGoogle Scholar
Broeren, A.P. & Bragg, M.B. 2001 Spanwise variation in the unsteady stalling flowfields of two-dimensional airfoil models. AIAA J. 37 (1), 130–132.CrossRefGoogle Scholar
Busquet, D. 2020 Study of a high Reynolds number flow around a two dimensional airfoil at stall: an approach coupling a rans framework and bifurcation theory. PhD thesis, Institut Polytechnique de Paris.Google Scholar
Cambier, L., Heib, S. & Plot, S. 2013 The ONERA elsA CFD Software: input from research and feedback from industry. Mech. Indust. 14 (3), 159–174.CrossRefGoogle Scholar
Cliquet, J., Houdeville, R. & Arnal, D. 2008 Application of laminar-turbulent transition criteria in Navier–Stokes computations. AIAA J. 46 (5), 1182–1190.CrossRefGoogle Scholar
Costes, M., Richez, F., Le Pape, A. & Gavériaux, R. 2015 Numerical investigation of three-dimensional effects during dynamic stall. Aerosp. Sci. Technol. 47, 216–237.CrossRefGoogle Scholar
Crouch, J.D., Garbaruk, A., Magidov, D. & Travin, A. 2009 Origin of transonic buffer on aerofoils. J. Fluid Mech. 628, 357–369.CrossRefGoogle Scholar
Deb, K. & Agarwal, R.B. 1995 Simulated binary crossover for continuous search space. Complex Syst. 9, 115–148.Google Scholar
Deb, K., Pratap, A., Agarwal, S. & Meyarivan, T. 2002 A fast and elitist multiobjective genetic algorithm: NSGA-II. IEEE Trans. Evol. Comput. 6 (2), 182–197.CrossRefGoogle Scholar
Dell'Orso, H. & Amitay, M. 2018 Parametric investigation of stall cell formation on a NACA 0015 airfoil. AIAA J. 56 (8), 3216–3228.CrossRefGoogle Scholar
Dhooge, A., Govaerts, W., Kuznetsov, Y.A., Meijer, H.G.E. & Sautois, B. 2008 New features of the software MatCont for bifurcation analysis of dynamical systems. Math. Comput. Model. Dyn. Syst. 14 (2), 147–175.CrossRefGoogle Scholar
Dijkstra, H.A., et al. 2014 Numerical bifurcation methods and their application to fluid dynamics: analysis beyond simulation. Commun. Comput. Phys. 15 (1), 1–45.CrossRefGoogle Scholar
Edwards, J.R. & Liou, M.-S. 1998 Low-diffusion flux splitting methods for flows at all speeds. AIAA J. 36, 1610–1617.CrossRefGoogle Scholar
Ekaterinaris, J.A. & Menter, F.R. 1994 Computation of oscillating airfoil flows with one- and two-equation turbulence models. AIAA J. 32 (12), 2359–2365.CrossRefGoogle Scholar
ElJack, E. & Soria, J. 2018 Bursting and reformation cycle of the laminar separation bubble over a NACA-0012 aerofoil: the underlying mechanism. arXiv:1807.09199.Google Scholar
ElJack, E. & Soria, J. 2020 Investigation of the low-frequency oscillations in the flowfield about an airfoil. AIAA J. 58 (10), 4271–4286.CrossRefGoogle Scholar
Fabre, D., Auguste, F. & Magnaudet, J. 2008 Bifurcations and symmetry breaking in the wake of axisymmetric bodies. Phys. Fluids 20 (051702), 1–4.CrossRefGoogle Scholar
Gatski, T.B. & Bonnet, J.-P. 2009 Compressibility, Turbulence and High Speed Flow. Elsevier.Google Scholar
Gear, R.W. 1971 Numerical Initial Value Problems in Ordinary Differential Equations. Prentice-Hall.Google Scholar
Gregory, N., Quincey, V.G., O'Reilly, C.L. & Hall, D.J. 1970 Progress report on observations of three-dimensional flow patterns obtained during stall development on aerofoils, and on the problem of measuring two-dimensional characteristics. Tech. Rep. CP 1146. Aeronautical Research Council.Google Scholar
Hristov, G. & Ansell, P.J. 2018 Post-stall hysteresis and flow field unsteadiness on a NACA0012 airfoil. AIAA J. 56 (7), 2528–2539.CrossRefGoogle Scholar
Iorio, M.C., Gonzalez, L.M. & Martinez-Cava, A. 2016 Global stability analysis of a compressible turbulent flow around a high-lift configuration. AIAA J. 54 (2), 373–385.CrossRefGoogle Scholar
Jain, R., Le Pape, A., Grubb, A., Costes, M., Richez, F. & Smith, M. 2018 High-resolution computational fluid dynamics predictions for the static and dynamic stall of a finite-span OA209 wing. J. Fluids Struct. 78, 126–145.CrossRefGoogle Scholar
Jones, B.M. 1933 An experimental study of the stalling of wings. Tech. Rep. R&M 1588. Aeronautical Research Council.Google Scholar
Keller, H.B. 1986 Lectures on Numerical Methods in Bifurcation Problems. Springer-Verlag.Google Scholar
Knoll, D.A. & Keyes, D.E. 2004 Jacobian-free Newton-Krylov methods: a survey of approaches and applications. J. Comput. Phys. 193, 357–397.CrossRefGoogle Scholar
Lehoucq, R.B., Sorensen, D.C. & Yang, C. 1998 ARPACK Users Guide: Solution of Large Scale Eigenvalue Problems by Implicitly Restarted Arnoldi Methods. SIAM.CrossRefGoogle Scholar
Mamun, C.K. & Tuckerman, L.S. 1995 Asymmetry and Hopf bifurcation in spherical Couette flow. Phys. Fluids 7, 80–91.CrossRefGoogle Scholar
Manni, L., Nishino, T. & Delafin, P.-L. 2016 Numerical study of airfoil stall cells using a very wide computational domain. Comput. Fluids 140, 260–269.CrossRefGoogle Scholar
Marchman, J.F., Sumantran, V. & Schaefer, C.G. 1987 Acoustic and turbulence influences on stall hysteresis. AIAA J. 25 (1), 50–51.CrossRefGoogle Scholar
Marquet, O., Sipp, D., Chomaz, J.-M. & Jacquin, L. 2008 Amplifier and resonator dynamics of a low-Reynolds-number recirculation bubble in a global framework. J. Fluid Mech. 605, 429–443.CrossRefGoogle Scholar
Mary, I. & Sagaut, P. 2002 Large eddy simulation of flow around an airfoil near stall. AIAA J. 40 (6), 1139–1145.CrossRefGoogle Scholar
McCullough, G.B. & Gault, D.E. 1951 Examples of three representative types of airfoil section stall at low speed. NACA Tech. Note 2502.Google Scholar
Meliga, P., Pujals, G. & Serre, E. 2012 Sensitivity of 2-d turbulent flow past a d-shaped cylinder using global stability. Phys. Fluids 24 (6), 061701.CrossRefGoogle Scholar
Menter, F.R. 1993 Zonal two equation $k-\omega$ turbulence models for aerodynamic flows. AIAA Paper 93-2906.CrossRefGoogle Scholar
Menter, F.R., Langtry, R.B., Likki, S.R., Suzen, Y.B., Huang, P.G. & Völker, S 2006 A correlation-based transition model using local variables. Part I: model formulation. Trans. ASME J. Turbomach. 128 (3), 413–422.CrossRefGoogle Scholar
Menter, F.R., Smirnov, P.E., Liu, T. & Avancha, R. 2015 A one-equation local correlation-based transition model. Flow Turbul. Combust. 95 (4), 583–619.CrossRefGoogle Scholar
Mettot, C., Renac, F. & Sipp, D. 2014 Computation of eigenvalue sensitivity to base flow modification in a discrete framework: application to open-loop control. J. Comput. Phys. 269, 234–258.CrossRefGoogle Scholar
Millikan, C.B. & Klein, A.L. 1933 The effect of turbulence. Aircraft Engng 5 (8), 169–174.CrossRefGoogle Scholar
Mittal, S. & Saxena, P. 2001 Hysteresis in flow past a NACA 0012 airfoil. Comput. Meth. Appl. Mech. Engng 191, 2179–2189.Google Scholar
Moss, G.F. & Murdin, P.M. 1971 Two dimensional low-speed tunnel tests on the NACA 0012 section including measurements made during pitching oscillations at the stall. Tech. Rep. CP 1145. Aeronautical Research Council.Google Scholar
Mueller, T.J., Pohlen, L.J., Conigliaro, P.E. & Jansen, B.J. 1983 The influence of free-stream disturbances on low Reynolds number airfoil experiments. Exp. Fluids 1, 3–14.CrossRefGoogle Scholar
Ohtake, T., Nakae, Y. & Motohashi, T. 2007 Nonlinearity of the aerodynamic characteristics of NACA0012 aerofoil at low Reynolds numbers. J. Japan Soc. Aeronaut. Space Sci. 55 (644), 439–445.Google Scholar
Pailhas, G., Houdeville, R., Barricau, P., Le Pape, A., Faubert, A., Loiret, P. & David, F. 2005 Experimental investigation of dynamic stall. In Proceedings of the 31st European Rotorcraft Forum. AIDAA.Google Scholar
Paladini, E., Marquet, O., Sipp, D., Robinet, J.-C. & Dandois, J. 2019 Various approaches to determine active regions in an unstable global mode: application to transonic buffet. J. Fluid Mech. 881, 617–647.CrossRefGoogle Scholar
Plante, F., Dandois, J., Beneddine, S., Laurendeau, É. & Sipp, D. 2021 Link between subsonic stall and transonic buffet on swept and unswept wings: from global stability analysis to nonlinear dynamics. J. Fluid Mech. 908, A16.CrossRefGoogle Scholar
Plante, F., Dandois, J. & Laurendeau, É. 2020 Similarities between cellular patterns occurring in transonic buffet and subsonic stall. AIAA J. 58 (1), 71–84.CrossRefGoogle Scholar
Pohlen, L.J. & Mueller, T.J. 1984 Boundary layer characteristics of the Miley airfoil at low Reynolds numbers. J. Aircraft 21 (9), 658–664.CrossRefGoogle Scholar
Richez, F., Le Pape, A. & Costes, M. 2015 Zonal detached-eddy simulation of separated flow around a finite-span wing. AIAA J. 53 (11), 3157–3166.CrossRefGoogle Scholar
Richez, F., Leguille, M. & Marquet, O. 2016 Selective frequency damping method for steady RANS solutions of turbulent separated flows around an airfoil at stall. Comput. Fluids 132, 51–61.CrossRefGoogle Scholar
Rinoie, K & Takemura, N 2004 Oscillating behaviour of laminar separation bubble formed on an aerofoil near stall. Aeronaut. J. 108 (1081), 153–163.CrossRefGoogle Scholar
Roe, P.L. 1981 Approximate Riemann solvers, parameter vectors and difference schemes. J. Comput. Phys. 43 (2), 357–372.CrossRefGoogle Scholar
Roshko, A. 1954 On the drag and shedding frequency of two dimensional bluff bodies. NACA Tech. Note 3169.Google Scholar
Saad, Y. 1992 Numerical Methods for Large Eigenvalue Problems. SIAM.Google Scholar
Sartor, F., Mettot, C., Bur, R. & Sipp, D. 2015 a Unsteadiness in transonic shock-wave/boundary-layer interactions: experimental investigation and global stability analysis. J. Fluid Mech. 781, 550–577.CrossRefGoogle Scholar
Sartor, F., Mettot, C. & Sipp, D. 2015 b Stability, receptivity, and sensitivity analyses of buffeting transonic flow over a profile. AIAA J. 53 (7), 1980–1993.CrossRefGoogle Scholar
Schewe, Günter 2001 Reynolds-number effects in flow around more-or-less bluff bodies. J. Wind Engng Ind. Aerodyn. 89 (14–15), 1267–1289.CrossRefGoogle Scholar
Schmitz, F.W. 1967 Aerodynamics of the model airplane. Part I. Airfoil measurements. NASA Tech. Memo. 60976.Google Scholar
Spalart, P.R. & Allmaras, S.R. 1994 A one-equation turbulence model for aerodynamic flows. La Rech. Aérosp. 1, 5–21.Google Scholar
Spalart, P.R. & Rumsey, C.L. 2007 Effective inflow conditions for turbulence models in aerodynamic calculations. AIAA J. 45 (10), 2544–2553.CrossRefGoogle Scholar
Szydlowski, J. & Costes, M. 2004 Simulation of flow around a static and oscillating in pitch NACA0015 airfoil using URANS and DES. In Proceedings of the ASME 2004 Heat Transfer/Fluids Engineering Summer Conference, Volume 2, Parts A and B, pp. 891–908. Springer.CrossRefGoogle Scholar
Tuckerman, L.S. & Barkley, D. 2000 Bifurcation analysis for timesteppers. In Numerical Methods for Bifurcation Problems and Large-Scale Dynamical Systems, IMA Volumes in Mathematics and its Applications, vol. 119, pp. 453–466. Springer.CrossRefGoogle Scholar
Wales, C., Gaitonde, A.L., Jones, D.P., Avitabile, D. & Champneys, A.R. 2012 Numerical continuation of high Reynolds number external flows. Intl J. Numer. Meth. Fluids 68, 135–159.CrossRefGoogle Scholar
Wang, R. & Xiao, Z. 2020 Transition effects on flow characteristics around a static two-dimensional airfoil. Phys. Fluids 32 (3), 035113.Google Scholar
Winkelman, A.E & Barlow, J.B 1980 Flowfield model for a rectangular planform wing beyond stall. AIAA J. 18 (8), 1006–1008.CrossRefGoogle Scholar
Yon, S.A & Katz, J. 1998 Study of the unsteady flow features on a stalled wing. AIAA J. 36 (3), 305–312.CrossRefGoogle Scholar
Zaman, K.B.M.Q., Bar-Sever, A. & Mangalam, S.M. 1987 Effect of acoustic excitation over airfoils near stall. J. Fluid Mech. 182, 127–148.CrossRefGoogle Scholar
Zaman, K.B.M.Q., McKinzie, D.J. & Rumsey, C.L. 1989 A natural low-frequency oscillation of the flow over an airfoil near stalling conditions. J. Fluid Mech. 54, 403–442.CrossRefGoogle Scholar