Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-17T01:16:46.605Z Has data issue: false hasContentIssue false
  • English
  • Français

Assessing and improving the accuracy of synthetic turbulence generation

Published online by Cambridge University Press:  13 November 2020

J. W. Patterson ,
R. Balin  and
K. E. Jansen Open the ORCID record for K. E. Jansen [Opens in a new window]
J. W. Patterson
Affiliation:
Department of Aerospace Engineering, University of Colorado, Boulder, CO80309, USA
R. Balin
Affiliation:
Department of Aerospace Engineering, University of Colorado, Boulder, CO80309, USA
K. E. Jansen*
Affiliation:
Department of Aerospace Engineering, University of Colorado, Boulder, CO80309, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

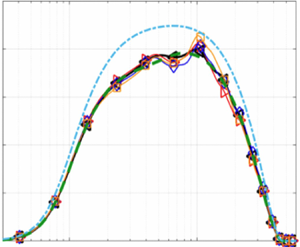
With the growing interest in scale-resolving simulations of spatially evolving boundary layers, synthetic turbulence generation (STG) has become a valuable tool for providing unsteady turbulent boundary conditions through a sum over a finite number of spatio-temporal Fourier modes with amplitude, direction and phase determined by a random number set. Recent developments of STG methods are designed to match target profiles for anisotropic and inhomogeneous Reynolds stresses. In this paper, it is shown that, for practical values of the number of modes, a given set of random numbers may produce Reynolds stress profiles that are 30 % off their target. To remedy this situation, the error in the STG stress prediction is decomposed into a steady-state bias and a purely unsteady part affecting the time convergence. Direct relationships between the random number vectors and both types of error are developed, allowing large collections of random number sets to be rapidly scanned and the best performers selected for a much improved agreement with the target. The process is verified for the inflow to a direct numerical simulation of a flat plate at $Re_\theta = 1000$. This paper demonstrates sufficient time convergence over a few flow-through times as well as a correction of the method's biases.

JFM classification

Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent boundary layers
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 906 , 10 January 2021 , R1
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Adamian, D. Y., Strelets, M. K. & Travin, A. K. 2011 An efficient method of synthetic turbulence generation at LES inflow in zonal RANS-LES approaches to computation of turbulent flows. Math. Mod. 23, 3–19.Google Scholar
Balin, R., Jansen, K. E. & Spalart, P. R. 2020 Wall-modeled LES of flow over a Gaussian bump with strong pressure gradients and separation. In AIAA AVIATION Forum, 3012.Google Scholar
Batten, P., Goldberg, U. & Chakravarthy, S. 2004 Interfacing statistical turbulence closures with large-eddy simulation. AIAA J. 42, 485–482.CrossRefGoogle Scholar
Béchara, W. 1994 Stochastic approach to noise modeling for free turbulent flows. AIAA J. 32, 455–463.CrossRefGoogle Scholar
Coleman, G. N., Rumsey, C. L. & Spalart, P. R. 2018 Numerical study of turbulent separation bubbles with varying pressure gradient and Reynolds number. J. Fluid Mech. 847, 28–70.CrossRefGoogle ScholarPubMed
Coles, D. E. 1962 The turbulent boundary layer in a compressible fluid. Tech. Rep. R-403-PR. The Rand Corporation.Google Scholar
Graham, J., Kanov, K., Yang, X. I. A., Lee, M. K., Malaya, N., Lalescu, C. C., Burns, R., Eyink, G., Szalay, A., Moser, R. D. et al. 2016 A web services-accessible database of turbulent channel flow and its use for testing a new integral wall model for LES. J. Turbul. 17 (2), 181–215.CrossRefGoogle Scholar
Huang, S. H., Li, Q. S. & Wu, J. R. 2010 A general inflow turbulence generator for large eddy simulation. J. Wind Engng Ind. Aerodyn. 98, 600–617.CrossRefGoogle Scholar
Jimenez, J., Hoyas, S., Simens, M. P. & Mizuno, Y. 2010 Turbulent boundary layers and channels at moderate Reynolds numbers. J. Fluid Mech. 657, 335–360.CrossRefGoogle Scholar
Karweit, M., Blanc-Benon, Ph., Juvé, B. & Comte-Bellot, G. 1991 Simulation of the propagation of an acoustic wave through a turbulent velocity field: a study of phase variance. J. Acoust. Soc. Am. 89, 52–62.CrossRefGoogle Scholar
Kraichnan, R. H. 1970 Diffusion by a random velocity field. Phys. Fluids 12, 22–31.CrossRefGoogle Scholar
Lund, T. S., Wu, X. & Squires, K. D. 1998 Generation of turbulent inflow data for spatially-developing boundary layer simulations. J. Comput. Phys. 140, 233–258.CrossRefGoogle Scholar
Shur, M. L., Spalart, P. R., Strelets, M. K. & Travin, A. K. 2008 A hybrid RANS-LES approach with delayed-DES and wall-modelled LES capabilities. Intl J. Heat Fluid Flow 29, 1638–1649.CrossRefGoogle Scholar
Shur, M. L., Spalart, P. R., Strelets, M. K. & Travin, A. K. 2014 Synthetic turbulence generators for RANS-LES interfaces in zonal simulations of aerodynamic and aeroacoustic problems. Flow Turbul. Combust. 93, 63–92.CrossRefGoogle Scholar
Smirnov, A., Shi, S. & Celik, I. 2001 Random flow generation technique for large eddy simulations and particle-dynamics modeling. Trans. ASME: J. Fluids Engng 123, 359–371.Google Scholar
Smits, A. J., Matheson, N. & Joubert, P. N. 1983 Low-Reynolds-number turbulent boundary layers in zero and favorable pressure gradients. J. Ship Res. 27, 147–157.Google Scholar
Spalart, P. R. & Allmaras, S. R. 1994 A one-equation turbulence model for aerodynamic flows. Recherche Aerospatiale 1, 5–21.Google Scholar
Spalart, P. R., Belyaev, K. V., Garbaruk, A. V., Shur, M. L., Strelets, M. K. & Travin, A. K. 2017 Large-eddy and direct numerical simulations of the Bachalo-Johnson flow with shock-induced separation. Flow Turbul. Combust. 99, 865–885.CrossRefGoogle Scholar
Spille-Kohoff, A. & Kaltenbach, H. J. 2001 Generation of turbulent inflow data with a prescribed shear-stress profile. In Proceedings of the Third AFOSR International Conference on DNS/LES (ed. Liu, C., Sakell, L. & Beutner, T.). Texas University at Arlington.Google Scholar
Wu, X. 2017 Inflow turbulence generation methods. Annu. Rev. Fluid Mech. 49, 23–49.CrossRefGoogle Scholar
Xie, Z. T. & Castro, I. P. 2008 Efficient generation of inflow conditions for large eddy simulation of street-scale flows. Flow Turbul. Combust. 81, 449–470.CrossRefGoogle Scholar