Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-17T17:05:48.307Z Has data issue: false hasContentIssue false
  • English
  • Français

Analytical shock solutions at large and small Prandtl number

Published online by Cambridge University Press:  14 June 2013

B. M. Johnson
B. M. Johnson*
Affiliation:
Lawrence Livermore National Laboratory, Livermore, CA 94550, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

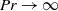Exact one-dimensional solutions to the equations of fluid dynamics are derived in the $\mathit{Pr}\rightarrow \infty $ and 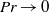$\mathit{Pr}\rightarrow 0$ limits (where 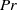$\mathit{Pr}$ is the Prandtl number). The solutions are analogous to the 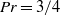$\mathit{Pr}= 3/ 4$ solution discovered by Becker and analytically capture the profile of shock fronts in ideal gases. The large-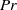$\mathit{Pr}$ solution is very similar to Becker’s solution, differing only by a scale factor. The small-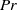$\mathit{Pr}$ solution is qualitatively different, with an embedded isothermal shock occurring above a critical Mach number. Solutions are derived for constant viscosity and conductivity as well as for the case in which conduction is provided by a radiation field. For a completely general density- and temperature-dependent viscosity and conductivity, the system of equations in all three limits can be reduced to quadrature. The maximum error in the analytical solutions when compared to a numerical integration of the finite-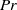$\mathit{Pr}$ equations is 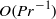$\mathit{O}({\mathit{Pr}}^{- 1} )$ as 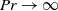$\mathit{Pr}\rightarrow \infty $ and 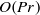$\mathit{O}(\mathit{Pr})$ as 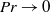$\mathit{Pr}\rightarrow 0$.

JFM classification

Compressible Flows: Compressible Flows Compressible Flows: Shock waves
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 726 , 10 July 2013 , R4
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Becker, R. 1922 Stosswelle und detonation. Z. Phys. 8, 321–362.Google Scholar
Bejan, A. 2004 Convection Heat Transfer. Wiley.Google Scholar
Bell, K. R. & Lin, D. N. C. 1994 Using FU Orionis outbursts to constrain self-regulated protostellar disk models. Astrophys. J. 427, 987–1004.CrossRefGoogle Scholar
Chapman, S. & Cowling, T. G. 1939 Mathematical Theory of Non-Uniform Gases. Cambridge University Press.Google Scholar
Clay, J. P. 1973 Turbulent mixing of temperature in water, air and mercury. PhD thesis, University of California at San Diego.Google Scholar
Hayes, W. D. 1960 Gasdynamic Discontinuities. Princeton University Press.Google Scholar
Hogg, J. D. 2012 Turbulent Rayleigh–Bénard convection of low-Prandtl-number gases. PhD thesis, University of California at Santa Barbara.Google Scholar
Iannelli, J. 2013 An exact nonlinear Navier–Stokes compressible-flow solution for CFD code verification. Intl J. Numer. Meth. Fluids 72, 157–176.Google Scholar
Jukes, J. D. 1957 The structure of a shock wave in a fully ionized gas. J. Fluid Mech. 3, 275–285.Google Scholar
Kaminski, E. & Jaupart, C. 2003 Laminar starting plumes in high-Prandtl-number fluids. J. Fluid Mech. 478, 287–298.Google Scholar
Khidr, M. A. & Mahmoud, M. A. A. 1985 The shock-wave structure for arbitrary Prandtl numbers and high Mach numbers. Astrophys. Space Sci. 113, 289–301.Google Scholar
Landau, L. D. & Lifshitz, E. M. 1987 Fluid Mechanics. Butterworth–Heinemann.Google Scholar
Lowrie, R. B. & Edwards, J. D. 2008 Radiative shock solutions with grey nonequilibrium diffusion. Shock Waves 18, 129–143.CrossRefGoogle Scholar
Lowrie, R. B. & Rauenzahn, R. M. 2007 Radiative shock solutions in the equilibrium diffusion limit. Shock Waves 16, 445–453.Google Scholar
Mihalas, D. & Mihalas, B. W. 1984 Foundations of Radiation Hydrodynamics. Oxford University Press.Google Scholar
Morduchow, M. & Libby, P. A. 1949 On a complete solution of the one-dimensional flow equations of a viscous, heat conducting, compressible gas. J. Aeronaut. Sci. 16, 674–684.Google Scholar
Mott-Smith, H. M. 1951 Phys. Rev. 82, 885–892.Google Scholar
Mozaffari, F. & Eslami, H. 2013 Equation of state for mercury: revisited. Phys. Chem. Liq. 51, 517–523.CrossRefGoogle Scholar
Ohtani, E. 2009 Melting relations and the equation of state of magmas at high pressure: application to geodynamics. Chem. Geol. 265, 279–288.CrossRefGoogle Scholar
Spitzer, L. 1956 Physics of Fully Ionized Gases. Interscience.Google Scholar
Thomas, L. H. 1944 Note on Becker’s theory of the shock front. J. Chem. Phys. 12, 449–452.CrossRefGoogle Scholar
White, F. M. 1991 Viscous Fluid Flow. McGraw–Hill.Google Scholar
Zel’dovich, Ya. B. & Raizer, Yu. P. 2002 Physics of Shock Waves and High-Temperature Hydrodynamic Phenomena. Dover.Google Scholar