Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-26T18:36:01.434Z Has data issue: false hasContentIssue false
  • English
  • Français

Analysis of turbulent coagulation in a jet with discretised population balance and DNS

Published online by Cambridge University Press:  28 February 2022

Malamas Tsagkaridis Open the ORCID record for Malamas Tsagkaridis [Opens in a new window] ,
Stelios Rigopoulos Open the ORCID record for Stelios Rigopoulos [Opens in a new window]  and
George Papadakis Open the ORCID record for George Papadakis [Opens in a new window]
Malamas Tsagkaridis
Affiliation:
Department of Mechanical Engineering, Imperial College London, Exhibition Road, London SW7 2AZ, UK
Stelios Rigopoulos*
Affiliation:
Department of Mechanical Engineering, Imperial College London, Exhibition Road, London SW7 2AZ, UK
George Papadakis
Affiliation:
Department of Aeronautics, Imperial College London, Exhibition Road, London SW7 2AZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

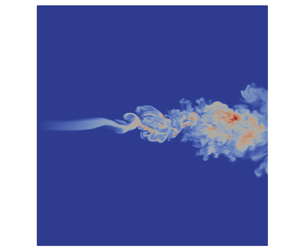
The objective of the present study is to investigate turbulence–coagulation interaction via direct numerical simulation (DNS) coupled with the population balance equation (PBE). Coagulation is an important process in several environmental and engineering applications involving turbulent flow, including soot formation, gas-phase synthesis of nanoparticles and atmospheric processes, but its interaction with turbulence is not yet fully understood. Particle dynamics can be described by the PBE, whose Reynolds decomposition leads to unclosed terms involving correlations of number density fluctuations. In this work, we employ a discretisation (sectional) method for the solution of the PBE, which is free of a priori assumptions regarding the particle size distribution (PSD), and couple it with a DNS for the flow field in order to study the behaviour and significance of the unknown correlations. At present, it is not feasible to resolve the Batchelor scales that result from diffusion at high Schmidt number, hence a unity Schmidt number is employed. The investigation is conducted on a three-dimensional planar jet laden with monodisperse nanoparticles, and coagulation in the free-molecule regime is considered. The correlations due to turbulent fluctuations of the particle number density are calculated at several points in the domain and found to be positive in most cases, except close to the jet break-up. The transport equation for the moments of the PSD is also studied, and it is found that the correlations make a considerable contribution to the time-averaged coagulation source term, up to $20\,\%$ on the jet centreline and $40\,\%$ close to the edges.

JFM classification

Reacting Flows: Turbulent reacting flows Drops and Bubbles: Aerosols/atomization Drops and Bubbles: Breakup/coalescence
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 937 , 25 April 2022 , A25
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alves Portela, F., Papadakis, G. & Vassilicos, J.C. 2020 The role of coherent structures and inhomogeneity in near-field interscale turbulent energy transfers. J. Fluid Mech. 896, A16.CrossRefGoogle Scholar
Balay, S., et al. 2021 PETSc users manual. Tech. Rep. ANL-95/11 – Revision 3.15. Argonne National Laboratory.Google Scholar
Brock, J.R., Kuhn, P.J. & Zehavi, D. 1986 Condensation aerosol formation and growth in a laminar coaxial jet: experimental. J. Aerosol Sci. 17 (1), 11–22.CrossRefGoogle Scholar
Browne, L.W.B., Antonia, R.A., Rajagopalan, S. & Chambers, A.J. 1983 Interaction region of a two-dimensional turbulent plane jet in still air. In Structure of Complex Turbulent Shear Flow, pp. 411–419. Springer.CrossRefGoogle Scholar
Buesser, B. & Pratsinis, S.E. 2012 Design of nanomaterial synthesis by aerosol processes. Annu. Rev. Chem. Biomol. Engng 3, 103–127.CrossRefGoogle ScholarPubMed
Cifuentes, L., Sellmann, J., Wlokas, I. & Kempf, A. 2020 Direct numerical simulations of nanoparticle formation in premixed and non-premixed flame-vortex interactions. Phys. Fluids 32 (9), 093605.CrossRefGoogle Scholar
Das, S. & Garrick, S.C. 2010 The effects of turbulence on nanoparticle growth in turbulent reacting jets. Phys. Fluids 22 (10), 103303.CrossRefGoogle Scholar
Davidson, P.A. 2004 Turbulence An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
Davies, A.E., Keffer, J.F. & Baines, W.D. 1975 Spread of a heated plane turbulent jet. Phys. Fluids 18 (7), 770–775.CrossRefGoogle Scholar
Delichatsios, M.A. & Probstein, R.F. 1975 Coagulation in turbulent flow: theory and experiment. J. Colloid Interface Sci. 51 (3), 394–405.CrossRefGoogle Scholar
Deo, R.C., Mi, J. & Nathan, G.J. 2008 The influence of Reynolds number on a plane jet. Phys. Fluids 20 (7), 075108.CrossRefGoogle Scholar
Di Veroli, G. & Rigopoulos, S. 2009 A study of turbulence-chemistry interaction in reactive precipitation via a population balance-transported PDF method. In Turbulence, Heat and Mass Transfer 6. Proceedings of the Sixth International Symposium on Turbulence, Heat and Mass Transfer, Rome, Italy (ed. K. Hanjalić, Y. Nagano & S. Jakirlić). Begell House.CrossRefGoogle Scholar
Di Veroli, G. & Rigopoulos, S. 2010 Modeling of turbulent precipitation: a transported population balance-PDF method. AIChE J. 56 (4), 878–892.Google Scholar
Di Veroli, G.Y. & Rigopoulos, S. 2011 Modeling of aerosol formation in a turbulent jet with the transported population balance equation-probability density function approach. Phys. Fluids 23 (4), 043305.CrossRefGoogle Scholar
Drake, R.L. 1972 A general mathematical survey of the coagulation equation. In Topics in Current Aerosol Research, Part 2 (ed. Hidy, G.M.), International Reviews in Aerosol Physics and Chemistry, vol. 3, pp. 204–376. Pergamon Press.Google Scholar
Drossinos, Y. & Housiadas, C. 2006 Aerosol flows. In Multiphase Flow Handbook (ed. C.T. Crowe), pp. 6-1–6-58. CRC Press.CrossRefGoogle Scholar
Elghobashi, S. 2006 An updated classification map of particle-laden turbulent flows. Fluid Mech. Appl. 81, 3–10.Google Scholar
Friedlander, S.K. 2000 Smoke, Dust, and Haze: Fundamentals of Aerosol Dynamics, 2nd edn. Oxford University Press.Google Scholar
Garrick, S.C. 2011 Effects of turbulent fluctuations on nanoparticle coagulation in shear flows. Aerosol Sci. Technol. 45 (10), 1272–1285.CrossRefGoogle Scholar
Garrick, S.C. & Khakpour, M. 2004 The effects of differential diffusion on nanoparticle coagulation in temporal mixing layers. Aerosol Sci. Technol. 38 (8), 851–860.CrossRefGoogle Scholar
Garrick, S.C., Lehtinen, K.E.J. & Zachariah, M.R. 2006 Nanoparticle coagulation via a Navier–Stokes/Nodal methodology: evolution of the particle field. J. Aerosol Sci. 37 (5), 555–576.CrossRefGoogle Scholar
Goudeli, E. & Pratsinis, S.E. 2016 Gas-phase manufacturing of nanoparticles: molecular dynamics and mesoscale simulations. Part. Sci. Technol. 34 (4), 483–493.CrossRefGoogle Scholar
Gutmark, E. & Wygnanski, I. 1976 The planar turbulent jet. J. Fluid Mech. 73 (3), 465–495.CrossRefGoogle Scholar
Jasak, H., Weller, H.G. & Gosman, A.D. 1999 High resolution NVD differencing scheme for arbitrarily unstructured meshes. Intl J. Numer. Meth. Fluids 31 (2), 431–449.3.0.CO;2-T>CrossRefGoogle Scholar
Jenkins, P.E. & Goldschmidt, V.W. 1973 Mean temperature and velocity in a plane turbulent jet. Trans. ASME J. Fluids Engng 95 (4), 581–584.CrossRefGoogle Scholar
Junzong, Z., Haiying, Q. & Jinsheng, W. 2013 Nanoparticle dispersion and coagulation in a turbulent round jet. Intl J. Multiphase Flow 54, 22–30.CrossRefGoogle Scholar
Klein, M., Sadiki, A. & Janicka, J. 2003 a A digital filter based generation of inflow data for spatially developing direct numerical or large eddy simulations. J. Comput. Phys. 186 (2), 652–665.CrossRefGoogle Scholar
Klein, M., Sadiki, A. & Janicka, J. 2003 b Investigation of the influence of the Reynolds number on a plane jet using direct numerical simulation. Intl J. Heat Fluid Flow 24 (6), 785–794.CrossRefGoogle Scholar
Kulmala, M., Vehkamäki, H., Petäjä, T., Dal Maso, M., Lauri, A., Kerminen, V.-M., Birmili, W. & McMurry, P.H. 2004 Formation and growth rates of ultrafine atmospheric particles: a review of observations. J. Aerosol Sci. 35 (2), 143–176.CrossRefGoogle Scholar
Landgrebe, J.D. & Pratsinis, S.E. 1990 A discrete-sectional model for particulate production by gas-phase chemical reaction and aerosol coagulation in the free-molecular regime. J. Colloid Interface Sci. 139 (1), 63–86.CrossRefGoogle Scholar
Le Ribault, C., Sarkar, S. & Stanley, S.A. 1999 Large eddy simulation of a plane jet. Phys. Fluids 11 (10), 3069–3083.CrossRefGoogle Scholar
Levich, V.G. 1962 Physicochemical Hydrodynamics. Prentice-Hall.Google Scholar
Levin, L.M. & Sedunov, Y.S. 1966 Gravitational coagulation of charged cloud drops in turbulent flow. Pure Appl. Geophys. 64 (1), 185–196.CrossRefGoogle Scholar
Levin, L.M. & Sedunov, Y.S. 1968 The theoretical model of the drop spectrum formation process in clouds. Pure Appl. Geophys. 69 (1), 320–335.CrossRefGoogle Scholar
Liu, A. & Rigopoulos, S. 2019 A conservative method for numerical solution of the population balance equation, and application to soot formation. Combust. Flame 205, 506–521.CrossRefGoogle Scholar
Miller, S.E. & Garrick, S.C. 2004 Nanoparticle coagulation in a planar jet. Aerosol Sci. Technol. 38 (1), 79–89.CrossRefGoogle Scholar
Olin, M., Rönkkö, T. & Dal Maso, M. 2015 CFD modeling of a vehicle exhaust laboratory sampling system: sulfur-driven nucleation and growth in diluting diesel exhaust. Atmos. Chem. Phys. 15 (9), 5305–5323.CrossRefGoogle Scholar
Pesmazoglou, I., Kempf, A.M. & Navarro-Martinez, S. 2017 Large eddy simulation of particle aggregation in turbulent jets. J. Aerosol Sci. 111, 1–17.CrossRefGoogle Scholar
Pope, S.B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Rajaratnam, N. 1976 Turbulent Jets. Elsevier.Google Scholar
Raman, V. & Fox, R.O. 2016 Modeling of fine-particle formation in turbulent flames. Annu. Rev. Fluid Mech. 48, 159–190.CrossRefGoogle Scholar
Ramaprian, B.R. & Chandrasekhara, M.S. 1985 LDA measurements in plane turbulent jets. Trans. ASME J. Fluids Engng 107 (2), 264–271.CrossRefGoogle Scholar
Reade, W.C. & Collins, L.R. 2000 A numerical study of the particle size distribution of an aerosol undergoing turbulent coagulation. J. Fluid Mech. 415, 45–64.CrossRefGoogle Scholar
Rigopoulos, S. 2007 PDF method for population balance in turbulent reactive flow. Chem. Engng Sci. 62 (23), 6865–6878.CrossRefGoogle Scholar
Rigopoulos, S. 2010 Population balance modelling of polydispersed particles in reactive flows. Prog. Energy Combust. Sci. 36 (4), 412–443.CrossRefGoogle Scholar
Rigopoulos, S. 2019 Modelling of soot aerosol dynamics in turbulent flow. Flow Turbul. Combust. 103 (3), 565–604.CrossRefGoogle Scholar
Saffman, P.G. & Turner, J.S. 1956 On the collision of drops in turbulent clouds. J. Fluid Mech. 1 (1), 16–30.CrossRefGoogle Scholar
Scott, W.T. 1967 Poisson statistics in distributions of coalescing droplets. J. Atmos. Sci. 24 (2), 221–225.2.0.CO;2>CrossRefGoogle Scholar
Settumba, N. & Garrick, S.C. 2003 Direct numerical simulation of nanoparticle coagulation in a temporal mixing layer via a moment method. J. Aerosol Sci. 34 (2), 149–167.CrossRefGoogle Scholar
Sewerin, F. & Rigopoulos, S. 2017 An LES-PBE-PDF approach for modeling particle formation in turbulent reacting flows. Phys. Fluids 29 (10), 105105.CrossRefGoogle Scholar
Sewerin, F. & Rigopoulos, S. 2018 An LES-PBE-PDF approach for predicting the soot particle size distribution in turbulent flames. Combust. Flame 189, 62–76.CrossRefGoogle Scholar
Sewerin, F. & Rigopoulos, S. 2019 Algorithmic aspects of the LES-PBE-PDF method for modeling soot particle size distributions in turbulent flames. Combust. Sci. Technol. 191 (5–6), 766–796.CrossRefGoogle Scholar
da Silva, C.B., Lopes, D.C. & Raman, V. 2015 The effect of subgrid-scale models on the entrainment of a passive scalar in a turbulent planar jet. J. Turbul. 16 (4), 342–366.CrossRefGoogle Scholar
Stanley, S.A. & Sarkar, S. 2000 Influence of nozzle conditions and discrete forcing on turbulent planar jets. AIAA J. 38 (9), 1615–1623.CrossRefGoogle Scholar
Stanley, S.A., Sarkar, S. & Mellado González, J.P. 2002 A study of the flow-field evolution and mixing in a planar turbulent jet using direct numerical simulation. J. Fluid Mech. 450, 377–407.CrossRefGoogle Scholar
Suresh, P.R., Srinivasan, K., Sundararajan, T. & Das, S.K. 2008 Reynolds number dependence of plane jet development in the transitional regime. Phys. Fluids 20 (4), 044105.CrossRefGoogle Scholar
Tang, H.Y., Rigopoulos, S. & Papadakis, G. 2020 A methodology for coupling DNS and discretised population balance for modelling turbulent precipitation. Intl J. Heat Fluid Flow 86, 108689.CrossRefGoogle Scholar
Taylor, G.I. 1935 Statistical theory of turbulence. Proc. R. Soc. Lond. A 23 (2), 421–444.CrossRefGoogle Scholar
Thomas, F.O. & Chu, H.C. 1989 An experimental investigation of the transition of a planar jet: subharmonic suppression and upstream feedback. Phys. Fluids A 1 (9), 1566–1587.CrossRefGoogle Scholar
Vemury, S., Kusters, K.A. & Pratsinis, S.E. 1994 Time-lag for attainment of the self-preserving particle size distribution by coagulation. J. Colloid Interface Sci. 165 (1), 53–59.CrossRefGoogle Scholar
Vemury, S. & Pratsinis, S.E. 1995 Self-preserving size distributions of agglomerates. J. Aerosol Sci. 26 (2), 175–185.CrossRefGoogle Scholar
Wang, L.P., Wexler, A.S. & Zhou, Y. 1998 On the collision rate of small particles in isotropic turbulence. I. Zero-inertia case. Phys. Fluids 10 (1), 266–276.CrossRefGoogle Scholar
Warshaw, M. 1967 Cloud droplet coalescence: statistical foundations and a one-dimensional sedimentation model. J. Atmos. Sci. 24 (3), 278–286.2.0.CO;2>CrossRefGoogle Scholar
Watanabe, T., Sakai, Y., Nagata, K., Ito, Y. & Hayase, T. 2014 Vortex stretching and compression near the turbulent/non-turbulent interface in a planar jet. J. Fluid Mech. 758, 754–785.CrossRefGoogle Scholar
Whitby, E.R. & McMurry, P.H. 1997 Modal aerosol dynamics modeling. Aerosol Sci. Technol. 27 (6), 673–688.CrossRefGoogle Scholar
Williams, M.M.R. & Loyalka, S.K. 1991 Aerosol Science – Theory and Practice: With Special Applications to the Nuclear Industry. Pergamon Press.Google Scholar
Xiao, D. & Papadakis, G. 2019 Nonlinear optimal control of transition due to a pair of vortical perturbations using a receding horizon approach. J. Fluid Mech. 861, 524–555.CrossRefGoogle Scholar