Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-26T04:09:36.611Z Has data issue: false hasContentIssue false
  • English
  • Français

An experimental study of flow–structure interaction regimes of a freely falling flexible cylinder

Published online by Cambridge University Press:  05 August 2022

Manuel Lorite-Díez Open the ORCID record for Manuel Lorite-Díez [Opens in a new window] ,
Patricia Ern Open the ORCID record for Patricia Ern [Opens in a new window] ,
Sébastien Cazin ,
Jérôme Mougel Open the ORCID record for Jérôme Mougel [Opens in a new window]  and
Rémi Bourguet Open the ORCID record for Rémi Bourguet [Opens in a new window]
Manuel Lorite-Díez
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse and CNRS, France
Patricia Ern*
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse and CNRS, France
Sébastien Cazin
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse and CNRS, France
Jérôme Mougel
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse and CNRS, France
Rémi Bourguet
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse and CNRS, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The fluid–structure interaction problem composed of an elongated, finite-length, flexible cylinder falling in a fluid at rest is investigated experimentally. Tomographic reconstruction of the cylinder and three-dimensional particle tracking velocimetry of the surrounding fluid, based on the Shake-The-Box algorithm, are used jointly to capture both solid and fluid motions. Starting from the rectilinear vertical fall characterized by a steady wake, focus is placed on subsequent regimes involving, mainly in the horizontal direction, periodic rigid-body motions (RBM) of weak amplitude or periodic large-amplitude bending oscillations (BO). Two RBM regimes are explored: the TRA regime where the cylinder exhibits translational oscillations in a plane perpendicular to its axis, and the AZI regime in which the body displays an azimuthal oscillation around its centre. The associated unsteady wakes are composed of counter-rotating vortices bending near the body ends to connect with the adjacent vortex rows. Specific organizations of the vortical structures are uncovered, depending on the regime. In particular, in the AZI regime, they present an antisymmetrical distribution relative to the midspan point. For a sufficiently long cylinder, BO regimes emerge, resembling the structural modes of an unsupported beam. The associated wakes exhibit a cellular organization. Within each cell delimited by two deformation nodes, two counter-rotating vortex rows are shed per oscillation cycle. Flow velocity fluctuations are in phase opposition on each side of a deformation node. For both RBM and BO regimes, frequency and phase analyses of cylinder and wake behaviours, along the span, highlight the spatio-temporal synchronization of the unsteady flow and moving body.

JFM classification

Wakes/Jets: Wakes Vortex Flows: Vortex shedding
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 946 , 10 September 2022 , A16
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adhikari, D. & Longmire, E.K. 2012 Visual hull method for tomographic PIV measurement of flow around moving objects. Exp. Fluids 53, 943–964.10.1007/s00348-012-1338-9CrossRefGoogle Scholar
Andersen, A., Pesavento, U. & Wang, Z.J. 2005 Unsteady aerodynamics of fluttering and tumbling plates. J. Fluid Mech. 541, 65–90.10.1017/S002211200500594XCrossRefGoogle Scholar
Auguste, F., Magnaudet, J. & Fabre, D. 2013 Falling styles of disks. J. Fluid Mech. 719, 388–405.10.1017/jfm.2012.602CrossRefGoogle Scholar
Boersma, P.R., Zhao, J., Rothstein, J.P. & Modarres-Sadeghi, Y. 2021 Experimental evidence of vortex-induced vibrations at subcritical Reynolds numbers. J. Fluid Mech. 922, R3.10.1017/jfm.2021.549CrossRefGoogle Scholar
Bourguet, R. 2020 Vortex-induced vibrations of a flexible cylinder at subcritical Reynolds number. J. Fluid Mech. 902, R3.10.1017/jfm.2020.676CrossRefGoogle Scholar
Bourguet, R., Karniadakis, G. & Triantafyllou, M. 2011 a Vortex-induced vibrations of a long flexible cylinder in shear flow. J. Fluid Mech. 677, 342–382.10.1017/jfm.2011.90CrossRefGoogle Scholar
Bourguet, R., Modarres-Sadeghi, Y., Karniadakis, G.E. & Triantafyllou, M.S. 2011 b Wake–body resonance of long flexible structures is dominated by counter-clockwise orbits. Phys. Rev. Lett. 107, 134502.10.1103/PhysRevLett.107.134502CrossRefGoogle Scholar
Buffoni, E. 2003 Vortex shedding in subcritical conditions. Phys. Fluids 15, 814–816.10.1063/1.1543943CrossRefGoogle Scholar
Chaplin, J.R., Bearman, P.W., Huera-Huarte, F.J. & Pattenden, R.J. 2005 Laboratory measurements of vortex-induced vibrations of a vertical tension riser in a stepped current. J. Fluids Struct. 21, 3–24.10.1016/j.jfluidstructs.2005.04.010CrossRefGoogle Scholar
Chow, A.C. & Adams, E.E. 2011 Prediction of drag coefficient and secondary motion of free-falling rigid cylindrical particles with and without curvature at moderate Reynolds number. ASCE J. Hydraul. Engng 137 (11), 1406–1414.10.1061/(ASCE)HY.1943-7900.0000437CrossRefGoogle Scholar
Chrust, M., Bouchet, G. & Dušek, J. 2013 Numerical simulation of the dynamics of freely falling discs. Phys. Fluids 25, 044102.10.1063/1.4799179CrossRefGoogle Scholar
Cossu, C. & Morino, L. 2000 On the instability of a spring-mounted circular cylinder in a viscous flow at low Reynolds numbers. J. Fluids Struct. 14 (2), 183–196.10.1006/jfls.1999.0261CrossRefGoogle Scholar
Dahl, J.M., Hover, F.S., Triantafyllou, M.S. & Oakley, O.H. 2010 Dual resonance in vortex-induced vibrations at subcritical and supercritical Reynolds numbers. J. Fluid Mech. 643, 395–424.10.1017/S0022112009992060CrossRefGoogle Scholar
Dolci, D.I. & Carmo, B.S. 2019 Bifurcation analysis of the primary instability in the flow around a flexibly mounted circular cylinder. J. Fluid Mech. 880, R5.10.1017/jfm.2019.754CrossRefGoogle Scholar
Ern, P., Mougel, J., Cazin, S., Lorite-Díez, M. & Bourguet, R. 2020 Bending oscillations of a cylinder freely falling in still fluid. J. Fluid Mech. 905, R5.10.1017/jfm.2020.828CrossRefGoogle Scholar
Ern, P., Risso, F., Fabre, D. & Magnaudet, J. 2012 Wake-induced oscillatory paths of bodies freely rising or falling in fluids. Annu. Rev. Fluid Mech. 44, 97–121.10.1146/annurev-fluid-120710-101250CrossRefGoogle Scholar
Fabre, D., Assemat, P. & Magnaudet, J. 2011 A quasi-static approach to the stability of the path of heavy bodies falling within a viscous fluid. J. Fluids Struct. 27, 758–767.10.1016/j.jfluidstructs.2011.03.013CrossRefGoogle Scholar
Fan, D., Wang, Z., Triantafyllou, M.S. & Karniadakis, G.E. 2019 Mapping the properties of the vortex-induced vibrations of flexible cylinders in uniform oncoming flow. J. Fluid Mech. 881, 815–858.10.1017/jfm.2019.738CrossRefGoogle Scholar
Fernandes, P.C., Risso, F., Ern, P. & Magnaudet, J. 2007 Oscillatory motion and wake instability of freely rising axisymmetric bodies. J. Fluid Mech. 573, 479–502.10.1017/S0022112006003685CrossRefGoogle Scholar
Gedikli, E.D., Chelidze, D. & Dahl, J.M. 2018 Observed mode shape effects on the vortex-induced vibration of bending dominated flexible cylinders simply supported at both ends. J. Fluids Struct. 81, 399–417.10.1016/j.jfluidstructs.2018.05.010CrossRefGoogle Scholar
Horowitz, M. & Williamson, C. 2006 Dynamics of a rising and falling cylinder. J. Fluids Struct. 22, 837–843.10.1016/j.jfluidstructs.2006.04.012CrossRefGoogle Scholar
Horowitz, M. & Williamson, C.H.K. 2010 Vortex-induced vibration of a rising and falling cylinder. J. Fluid Mech. 662, 352.10.1017/S0022112010003265CrossRefGoogle Scholar
Huera-Huarte, F.J. & Bearman, P.W. 2009 Wake structures and vortex-induced vibrations of a long flexible cylinder – Part 1: dynamic response. J. Fluids Struct. 25 (6), 969–990.10.1016/j.jfluidstructs.2009.03.007CrossRefGoogle Scholar
Inoue, O. & Sakuragi, A. 2008 Vortex shedding from a circular cylinder of finite length at low Reynolds numbers. Phys. Fluids 20 (3), 033601.10.1063/1.2844875CrossRefGoogle Scholar
Jayaweera, K.O.L.F. & Mason, B.J. 1965 The behaviour of freely falling cylinders and cones in a viscous fluid. J. Fluid Mech. 22 (4), 709–720.10.1017/S002211206500109XCrossRefGoogle Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.10.1017/S0022112095000462CrossRefGoogle Scholar
Karniadakis, G.E. & Triantafyllou, G.S. 1992 Three-dimensional dynamics and transition to turbulence in the wake of bluff objects. J. Fluid Mech. 238, 1–30.10.1017/S0022112092001617CrossRefGoogle Scholar
Kramer, O.J.I., de Moel, P.J., Raaghav, S.K.R., Baars, E.T., van Vugt, W.H., Breugem, W.-P., Padding, J.T. & van der Hoek, J.P. 2021 Can terminal settling velocity and drag of natural particles in water ever be predicted accurately? Drink. Water Engng Sci. 14 (1), 53–71.10.5194/dwes-14-53-2021CrossRefGoogle Scholar
Lau, E.M., Zhang, J.D., Jia, Y.X., Huang, W.X. & Xu, C.X. 2019 Vortical structures in the wake of falling plates. J. Vis. 22, 15–24.10.1007/s12650-018-0520-4CrossRefGoogle Scholar
Marchildon, E.K., Clamen, A. & Gauvin, W.H. 1964 Drag and oscillatory motion of freely falling cylindrical particles. Can. J. Chem. Engng 42 (4), 178–182.10.1002/cjce.5450420410CrossRefGoogle Scholar
Mathai, V., Zhu, X., Sun, C. & Lohse, D. 2017 Mass and moment of inertia govern the transition in the dynamics and wakes of freely rising and falling cylinders. Phys. Rev. Lett. 119, 054501.10.1103/PhysRevLett.119.054501CrossRefGoogle ScholarPubMed
Meliga, P. & Chomaz, J.-M. 2011 An asymptotic expansion for the vortex-induced vibrations of a circular cylinder. J. Fluid Mech. 671, 137–167.10.1017/S0022112010005550CrossRefGoogle Scholar
Mittal, S. & Singh, S. 2005 Vortex-induced vibrations at subcritical $Re$. J. Fluid Mech. 534, 185–194.10.1017/S0022112005004635CrossRefGoogle Scholar
Namkoong, K., Yoo, J.Y. & Choi, H.G. 2008 Numerical analysis of two-dimensional motion of a freely falling circular cylinder in an infinite fluid. J. Fluid Mech. 604, 33–54.10.1017/S0022112008001304CrossRefGoogle Scholar
Newman, D.J. & Karniadakis, G.E. 1997 A direct numerical simulation study of flow past a freely vibrating cable. J. Fluid Mech. 344, 95–136.10.1017/S002211209700582XCrossRefGoogle Scholar
Païdoussis, M.P., Price, S.J. & De Langre, E. 2010 Fluid–Structure Interactions: Cross-Flow-Induced Instabilities. Cambridge University Press.10.1017/CBO9780511760792CrossRefGoogle Scholar
Pesavento, U. & Wang, Z.J. 2004 Falling paper: Navier–Stokes solutions. Model of fluid forces, and center of mass elevation. Phys. Rev. Lett. 93, 144501.10.1103/PhysRevLett.93.144501CrossRefGoogle ScholarPubMed
Ramberg, S.E. & Griffin, O.M. 1974 Vortex formation in the wake of a vibrating, flexible cable. J. Fluids Engng 96 (4), 317–322.10.1115/1.3447164CrossRefGoogle Scholar
Romero-Gomez, P. & Richmond, M.C. 2016 Numerical simulation of circular cylinders in free-fall. J. Fluids Struct. 61, 154–167.10.1016/j.jfluidstructs.2015.11.010CrossRefGoogle Scholar
Roshko, A. 1993 Perspectives on bluff body aerodynamics. J. Wind Engng Ind. Aerodyn. 49 (1–3), 79–100.10.1016/0167-6105(93)90007-BCrossRefGoogle Scholar
Sarpkaya, T. 2004 A critical review of the intrinsic nature of vortex-induced vibrations. J. Fluids Struct. 19 (4), 389–447.10.1016/j.jfluidstructs.2004.02.005CrossRefGoogle Scholar
Savitzky, A. & Golay, M.J.E. 1964 Smoothing and differentiation of data by simplified least squares procedures. Anal. Chem. 36 (8), 1627–1639.10.1021/ac60214a047CrossRefGoogle Scholar
Scarano, F. 2012 Tomographic PIV: principles and practice. Meas. Sci. Technol. 24 (1), 012001.10.1088/0957-0233/24/1/012001CrossRefGoogle Scholar
Schanz, D., Gesemann, S. & Schröder, A. 2016 Shake-The-Box: Lagrangian particle tracking at high particle image densities. Exp. Fluids 57 (5), 70.10.1007/s00348-016-2157-1CrossRefGoogle Scholar
Schanz, D., Gesemann, S., Schröder, A., Wieneke, B. & Novara, M. 2012 Non-uniform optical transfer functions in particle imaging: calibration and application to tomographic reconstruction. Meas. Sci. Technol. 24 (2), 024009.10.1088/0957-0233/24/2/024009CrossRefGoogle Scholar
Schanz, D., Schröder, A., Gesemann, S., Michaelis, D. & Wieneke, B. 2013 Shake-The-Box: a highly efficient and accurate tomographic particle tracking velocimetry (TOMO-PTV) method using prediction of particle positions. In 10th International Symposium on Particle Image Velocimetry, Delft, The Netherlands. Delft University of Technology.Google Scholar
Seyed-Aghazadeh, B., Edraki, M. & Modarres-Sadeghi, Y. 2019 Effects of boundary conditions on vortex-induced vibration of a fully submerged flexible cylinder. Exp. Fluids 60, 38.10.1007/s00348-019-2681-xCrossRefGoogle Scholar
Singh, S.P. & Mittal, S. 2005 Vortex-induced oscillations at low Reynolds numbers: hysteresis and vortex-shedding modes. J. Fluids Struct. 20, 1085–1104.10.1016/j.jfluidstructs.2005.05.011CrossRefGoogle Scholar
Tchoufag, J., Fabre, D. & Magnaudet, J. 2014 Global linear stability analysis of the wake and path of buoyancy-driven disks and thin cylinders. J. Fluid Mech. 740, 278–311.10.1017/jfm.2013.642CrossRefGoogle Scholar
Toupoint, C., Ern, P. & Roig, V. 2019 Kinematics and wake of freely falling cylinders at moderate Reynolds numbers. J. Fluid Mech. 866, 82–111.10.1017/jfm.2019.77CrossRefGoogle Scholar
Wieneke, B. 2008 Volume self-calibration for 3D particle image velocimetry. Exp. Fluids 45 (4), 549–556.10.1007/s00348-008-0521-5CrossRefGoogle Scholar
Wieneke, B. 2012 Iterative reconstruction of volumetric particle distribution. Meas. Sci. Technol. 24 (2), 024008.10.1088/0957-0233/24/2/024008CrossRefGoogle Scholar
Will, J.B. & Krug, D. 2021 Rising and sinking in resonance: mass distribution critically affects buoyancy-driven spheres via rotational dynamics. Phys. Rev. Lett. 126, 174502.10.1103/PhysRevLett.126.174502CrossRefGoogle ScholarPubMed
Will, J.B., Mathai, V., Huisman, S.G., Lohse, D., Sun, C. & Krug, D. 2021 Kinematics and dynamics of freely rising spheroids at high Reynolds numbers. J. Fluid Mech. 912, A16.10.1017/jfm.2020.1104CrossRefGoogle Scholar
Williamson, C.H.K. 1989 Oblique and parallel modes of vortex shedding in the wake of a circular cylinder at low Reynolds numbers. J. Fluid Mech. 206, 579–627.10.1017/S0022112089002429CrossRefGoogle Scholar
Williamson, C.H.K. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid Mech. 28, 477–539.10.1146/annurev.fl.28.010196.002401CrossRefGoogle Scholar
Williamson, C.H.K. & Brown, G.L. 1998 A series in $1/\sqrt {Re}$ to represent the Strouhal–Reynolds number relationship of the cylinder wake. J. Fluids Struct. 12 (8), 1073–1085.10.1006/jfls.1998.0184CrossRefGoogle Scholar
Williamson, C.H.K. & Govardhan, R. 2004 Vortex-induced vibrations. Annu. Rev. Fluid Mech. 36, 413–455.10.1146/annurev.fluid.36.050802.122128CrossRefGoogle Scholar
Wu, X., Ge, F. & Hong, Y. 2012 A review of recent studies on vortex-induced vibrations of long slender cylinders. J. Fluids Struct. 28, 292–308.10.1016/j.jfluidstructs.2011.11.010CrossRefGoogle Scholar
Zhang, H.-Q., Fey, U., Noack, B.R., König, M. & Eckelmann, H. 1995 On the transition of the cylinder wake. Phys. Fluids 7 (4), 779–794.10.1063/1.868601CrossRefGoogle Scholar

Lorite-Díez et al. supplementary movie 1

\caption{Illustration of the wake pattern and of the cylinder behaviour in a TRA regime with $d = 1.12$mm, $Ar\simeq47$, $Re\simeq49$, $Ca\sim2.6$, and $L/d\simeq35$. Wake is depicted by instantaneous iso-surfaces of the $\lambda_2$ criterion ($\lambda_{2}d^{2}/U^{2} = -0.0004$) colored by iso-contours of the x-vorticity ($\omega_{x}d/U$ $\in [-0.1, 0.1]$; blue to red).}

Download Lorite-Díez et al. supplementary movie 1(Video)
Video 3.1 MB

Lorite-Díez et al. supplementary movie 2

\caption{Illustration of the wake pattern and of the cylinder behaviour in a TRA regime with $d = 1.9$mm, $Ar\simeq103$, $Re\simeq124$, $Ca {\cal O} (10^{-2})$, and $L/d\simeq10$. Wake is depicted by instantaneous iso-surfaces of the $\lambda_2$ criterion ($\lambda_{2}d^{2}/U^{2} = -0.005$) colored by iso-contours of the x-vorticity ($\omega_{x}d/U$ $\in [-0.15, 0.15]$; blue to red).}

Download Lorite-Díez et al. supplementary movie 2(Video)
Video 2.6 MB

Lorite-Díez et al. supplementary movie 3

\caption{Illustration of the wake pattern and of the cylinder behaviour in an AZI regime with $d = 2.55$mm, $Ar\simeq161$, $Re\simeq217$, $Ca {\cal O} (10^{-4})$, and $L/d\simeq20$. Wake is depicted by instantaneous iso-surfaces of the $\lambda_2$ criterion ($\lambda_{2}d^{2}/U^{2} = -0.01$) colored by iso-contours of the x-vorticity ($\omega_{x}d/U$ $\in [-0.2, 0.2]$; blue to red.)}

Download Lorite-Díez et al. supplementary movie 3(Video)
Video 2.8 MB

Lorite-Díez et al. supplementary movie 4

\caption{Bending oscillations of the cylinder during its fall following a M$_1$ regime. }

Download Lorite-Díez et al. supplementary movie 4(Video)
Video 2.4 MB

Lorite-Díez et al. supplementary movie 5

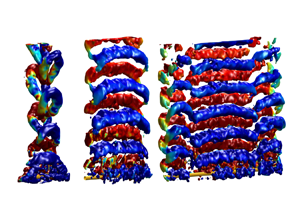
\caption{Illustration of the wake pattern and of the cylinder behaviour in a M$_1$ regime with $d = 1.09$mm, $Ar\simeq45$, $Re\simeq42$, $Ca \simeq 36$, and $L/d\simeq68$. Wake is depicted by instantaneous iso-surfaces of the $\lambda_2$ criterion ($\lambda_{2}d^{2}/U^{2} = -0.0004$) colored by iso-contours of the x-vorticity ($\omega_{x}d/U$ $\in [-0.1, 0.1]$; blue to red).}

Download Lorite-Díez et al. supplementary movie 5(Video)
Video 5.2 MB

Lorite-Díez et al. supplementary movie 6

\caption{Illustration of the wake pattern and of the cylinder behaviour in a M$_2$ regime with $d = 1.02$mm, $Ar\simeq40$, $Re\simeq37$, $Ca \simeq 210$, and $L/d\simeq107$. Wake is depicted by instantaneous iso-surfaces of the $\lambda_2$ criterion ($\lambda_{2}d^{2}/U^{2} = -0.0004$) colored by iso-contours of the x-vorticity ($\omega_{x}d/U$ $\in [-0.1, 0.1]$; blue to red).}

Download Lorite-Díez et al. supplementary movie 6(Video)
Video 5.9 MB
Supplementary material: PDF

Lorite-Díez et al. supplementary material

Captions for movies 1-6

Download Lorite-Díez et al. supplementary material(PDF)
PDF 21.1 KB