Article contents
Air entrapment at impact of a conus onto a liquid
Published online by Cambridge University Press: 03 July 2023
Abstract
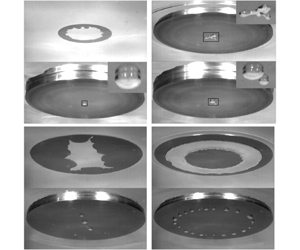
In this experimental work, a conus impacts a deep liquid pool at a speed varying from 1.3 to  $19.0\ {\rm cm}\ {\rm s}^{-1}$. Two liquids (2.5 % butanol–water solution or distilled water) and four coni made from duralumin with a diameter of 180 mm and different deadrise angles
$19.0\ {\rm cm}\ {\rm s}^{-1}$. Two liquids (2.5 % butanol–water solution or distilled water) and four coni made from duralumin with a diameter of 180 mm and different deadrise angles  $\beta$ (
$\beta$ ( $2^{\circ }$,
$2^{\circ }$,  $3^{\circ }$,
$3^{\circ }$,  $4^{\circ }$ and 5
$4^{\circ }$ and 5 $^{\circ }$) are tested. An air cushion is trapped between the conus solid surface and the liquid. Several types of bubble patterns after the collapse of the air cushion are observed: one or multiple bubbles near the conus centre (vertex), irregular trails of bubbles on the conus surface and a ring of bubbles in a ‘necklace’-shaped arrangement. With a total internal reflection set-up and appropriate image post-processing, the external and internal radii of the ring-shaped wetted area are estimated for each frame. The external (internal) radius increases (decreases) in time following a linear (exponential) law. The speed of the outer border of the wetted area is in agreement with the Wagner theory for a body impacting onto a liquid. The initial radius of the annular touchdown region is estimated as the intersection of the relevant fitting curves. In the studied range of parameters, the initial radius obeys a universal scaling law, which follows from the air–water lubrication–inertia balance.
$^{\circ }$) are tested. An air cushion is trapped between the conus solid surface and the liquid. Several types of bubble patterns after the collapse of the air cushion are observed: one or multiple bubbles near the conus centre (vertex), irregular trails of bubbles on the conus surface and a ring of bubbles in a ‘necklace’-shaped arrangement. With a total internal reflection set-up and appropriate image post-processing, the external and internal radii of the ring-shaped wetted area are estimated for each frame. The external (internal) radius increases (decreases) in time following a linear (exponential) law. The speed of the outer border of the wetted area is in agreement with the Wagner theory for a body impacting onto a liquid. The initial radius of the annular touchdown region is estimated as the intersection of the relevant fitting curves. In the studied range of parameters, the initial radius obeys a universal scaling law, which follows from the air–water lubrication–inertia balance.
- Type
- JFM Rapids
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
Carrat et al. Supplementary Movie 1
Typical impact event. Experimental conditions : liquid BWS, V = 4.2 cm/s and β = 2°.
Carrat et al. Supplementary Movie 2
One bubble. Experimental conditions : liquid BWS, V = 7.7 cm/s and β = 2°.
Carrat et al. Supplementary Movie 3
Multiple bubbles. Experimental conditions : liquid BWS, V = 4.9 cm/s and β = 2°.
- 3
- Cited by



