Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-26T06:34:07.781Z Has data issue: false hasContentIssue false
  • English
  • Français

Ageostrophic instability in rotating, stratified interior vertical shear flows

Published online by Cambridge University Press:  19 August 2014

Peng Wang ,
James C. McWilliams  and
Claire Ménesguen
Peng Wang*
Affiliation:
Department of Atmospheric and Oceanic Sciences, University of California, Los Angeles, CA 90095-1565, USA
James C. McWilliams
Affiliation:
Department of Atmospheric and Oceanic Sciences, University of California, Los Angeles, CA 90095-1565, USA
Claire Ménesguen
Affiliation:
Department of Marine Geosciences, IFREMER, 29280 Plouzané, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The linear instability of several rotating, stably stratified, interior vertical shear flows $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\overline{U}(z)$ is calculated in Boussinesq equations. Two types of baroclinic, ageostrophic instability, AI1 and AI2, are found in odd-symmetric 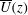$\overline{U}(z)$ for intermediate Rossby number (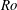$\mathit{Ro}$). AI1 has zero frequency; it appears in a continuous transformation of the unstable mode properties between classic baroclinic instability (BCI) and centrifugal instability (CI). It begins to occur at intermediate 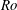$\mathit{Ro}$ values and horizontal wavenumbers ($k,l$) that are far from $l= 0$ or 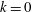$k = 0$, where the growth rate of BCI or CI is the strongest. AI1 grows by drawing kinetic energy from the mean flow, and the perturbation converts kinetic energy to potential energy. The instability AI2 has inertia critical layers (ICL); hence it is associated with inertia-gravity waves. For an unstable AI2 mode, the coupling is either between an interior balanced shear wave and an inertia-gravity wave (BG), or between two inertia-gravity waves (GG). The main energy source for an unstable BG mode is the mean kinetic energy, while the main energy source for an unstable GG mode is the mean available potential energy. AI1 and BG type AI2 occur in the neighbourhood of $A-S= 0$ (a sign change in the difference between absolute vertical vorticity and horizontal strain rate in isentropic coordinates; see McWilliams et al., Phys. Fluids, vol. 10, 1998, pp. 3178–3184), while GG type AI2 arises beyond this condition. Both AI1 and AI2 are unbalanced instabilities; they serve as an initiation of a possible local route for the loss of balance in 3D interior flows, leading to an efficient energy transfer to small scales.

JFM classification

Geophysical and Geological Flows: Baroclinic flows Instability: Instability Geophysical and Geological Flows: Waves in rotating fluids
Type
Papers
Information
Journal of Fluid Mechanics , Volume 755 , 25 September 2014 , pp. 397 - 428
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Boss, E., Paldor, N. & Thompson, L. 1996 Stability of a potential vorticity front: from quasi-geostrophy to shallow water. J. Fluid Mech. 315, 65–84.CrossRefGoogle Scholar
Bouchut, F., Ribstein, B. & Zeitlin, V. 2011 Inertial, barotropic, and baroclinic instabilities of the Bickley jet in two-layer rotating shallow water model. Phys. Fluids 23, 126601.CrossRefGoogle Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Cambridge University Press.Google Scholar
Charney, J. G. 1947 The dynamics of long waves in a baroclinic westerly current. J. Atmos. Sci. 4, 136–162.Google Scholar
Charney, J. G.1962 Integration of the primitive and balanced equations. In Proceedings Intl. Symp., Numerical Weather Prediction pp. 131–152.Google Scholar
Charney, J. G. 1971 Geostrophic turbulence. J. Atmos. Sci. 28, 1087–1094.Google Scholar
Drazin, P. G. & Reid, W. H. 1981 Hydrodynamic Stability. Cambridge University Press.Google Scholar
Dritschel, D. G. & Vanneste, J. 2006 Instability of a shallow-water potential-vorticity front. J. Fluid Mech. 561, 237–254.CrossRefGoogle Scholar
Eady, E. T. 1949 Long waves and cyclone waves. Tellus 1, 33–52.CrossRefGoogle Scholar
Gent, P. R. & McWilliams, J. C. 1982 Intermediate model solutions to the Lorenz equations: strange attractors and other phenomena. J. Atmos. Sci. 39, 3–13.Google Scholar
Griffiths, R. W., Killworth, P. D. & Stern, M. E. 1982 Ageostrophic instability of ocean currents. J. Fluid Mech. 117, 343–377.CrossRefGoogle Scholar
Gula, J., Plougonven, R. & Zeitlin, V. 2009 Ageostrophic instabilities of fronts in a channel in a stratified rotating fluid. J. Fluid Mech. 627, 485–507.CrossRefGoogle Scholar
Gula, J. & Zeitlin, V. 2010 Instabilities of buoyancy-driven coastal currents and their nonlinear evolution in the two-layer rotating shallow water model. Part 1. Passive lower layer. J. Fluid Mech. 659, 69–93.CrossRefGoogle Scholar
Gula, J., Zeitlin, V. & Bouchut, F. 2010 Instabilities of buoyancy-driven coastal currents and their nonlinear evolution in the two-layer rotating shallow water model. Part 2. Active lower layer. J. Fluid Mech. 665, 209–237.Google Scholar
Hayashi, Y. Y. & Young, W. R. 1987 Stable and unstable shear modes of rotating parallel flows in shallow waters. J. Fluid Mech. 184, 477–504.Google Scholar
Hoskins, B. J. 1974 The role of potential vorticity in symmetric stability and instability. Q. J. R. Meteorol. Soc. 100, 480–482.Google Scholar
Jones, W. L. 1967 Propagation of internal gravity waves in fluids with shear flow and rotation. J. Fluid Mech. 30, 439–448.Google Scholar
Killworth, P., Paldor, N. & Stern, M. 1984 Wave propagation and growth on a surface front in a two-layer geostrophic current. J. Mar. Res. 42, 761–785.CrossRefGoogle Scholar
Kubokawa, A. 1985 Instability of a geostrophic front and its energetics. Geophys. Astrophys. Fluid Dyn. 33, 323–357.CrossRefGoogle Scholar
Kubokawa, A. 1986 Instability caused by the coalescence of two modes of a one-layer coastal current with a surface front. J. Oceanogr. 42, 373–380.Google Scholar
Leith, C. E. 1980 Nonlinear normal mode initialization and quasi-geostrophic theory. J. Atmos. Sci. 37, 958–968.Google Scholar
Lorenz, E. 1960 Energy and numerical weather prediction. Tellus 12, 364–373.CrossRefGoogle Scholar
Lorenz, E. N. 1980 Attractor sets and quasi-geostrophic equilibrium. J. Atmos. Sci. 37, 1685–1699.Google Scholar
Lorenz, E. N. 1986 On the existence of a slow manifold. J. Atmos. Sci. 43, 1547–1558.Google Scholar
Lorenz, E. N. & Krishnamurthy, V. 1987 On the non-existence of a slow manifold. J. Atmos. Sci. 44, 2940–2950.Google Scholar
McWilliams, J. C., Molemaker, M. J. & Yavneh, I. 2004 Ageostrophic, anticyclonic instability of a geostrophic, barotropic boundary current. Phys. Fluids 16, 3720–3725.Google Scholar
McWilliams, J. C. & Yavneh, I. 1998 Fluctuation growth and instability associated with a singularity of the Balance equations. Phys. Fluids 10, 2587–2596.Google Scholar
McWilliams, J. C., Yavneh, I., Cullen, M. J. P. & Gent, P. R. 1998 The breakdown of large-scale flows in rotating, stratified fluids. Phys. Fluids 10, 3178–3184.Google Scholar
Ménesguen, C., McWilliams, J. C. & Molemaker, M. J. 2012 Ageostrophic instability in a rotating stratified interior jet. J. Fluid Mech. 711, 599–619.Google Scholar
Miles, J. W. 1961 On the stability of heterogeneous shear flows. J. Fluid Mech. 10, 496–508.Google Scholar
Molemaker, M. J., McWilliams, J. C. & Yavneh, I. 2001 Instability and equilibration of centrifugally stable stratified Taylor–Couette flow. Phys. Rev. Lett. 86, 5270–5273.Google Scholar
Molemaker, M. J., McWilliams, J. C. & Yavneh, I. 2005 Baroclinic instability and loss of balance. J. Phys. Oceanogr. 35, 1505–1517.CrossRefGoogle Scholar
Nakamura, N. 1988 Scale selection of baroclinic instability – effects of stratification and non-geostrophy. J. Atmos. Sci. 45, 3253–3268.2.0.CO;2>CrossRefGoogle Scholar
Ooyama, K. 1966 On the stability of the baroclinic circular vortex: a sufficient condition for instability. J. Atmos. Sci. 23, 43–53.Google Scholar
Paldor, N. 1983 Linear stability and stable modes of geostrophic fronts. Geophys. Astrophys. Fluid Dyn. 24, 299–326.Google Scholar
Pedlosky, J. 1979 Geophysical Fluid Dynamics. Springer.Google Scholar
Phillips, N. A. 1954 Energy transformations and meridional circulations associated with simple baroclinic waves in a two-level, quasi-geostrophic model. Tellus 6, 273–286.Google Scholar
Plougonven, R., Muraki, D. J. & Snyder, C. 2005 A baroclinic instability that couples balanced motions and gravity waves. J. Atmos. Sci. 62, 1545–1559.Google Scholar
Ripa, P. 1983 General stability conditions for zonal flows in a one-layer model on the beta-plane or the sphere. J. Fluid Mech. 126, 463–489.Google Scholar
Sakai, S. 1989 Rossby–Kelvin instability: a new type of ageostrophic instability caused by a resonance between Rossby waves and gravity waves. J. Fluid Mech. 202, 149–176.Google Scholar
Stone, P. H. 1966 On non-geostrophic baroclinic stability. J. Atmos. Sci. 23, 390–400.Google Scholar
Stone, P. H. 1970 On non-geostrophic baroclinic stability: Part II. J. Atmos. Sci. 27, 721–726.Google Scholar
Sutyrin, G. G. 2007 Ageostrophic instabilities in a horizontally uniform baroclinic flow along a slope. J. Fluid Mech. 588, 463–473.Google Scholar
Sutyrin, G. G. 2008 Lack of balance in continuously stratified rotating flows. J. Fluid Mech. 615, 93–100.Google Scholar
Vanneste, J. & Yavneh, I. 2007 Unbalanced instabilities of rapidly rotating stratified shear flows. J. Fluid Mech. 584, 373–396.Google Scholar
Wang, P., McWilliams, J. C. & Kizner, Z. 2012 Ageostrophic instability in rotating shallow water. J. Fluid Mech. 712, 327–353.Google Scholar
Warn, T. 1997 Nonlinear balance and quasi-geostrophic sets. Atmos.-Ocean 35, 135–145.Google Scholar
Warn, T., Bokhove, O., Shepherd, T. G. & Vallis, G. K. 1995 Rossby number expansions, slaving principles, and balance dynamics. Q. J. R. Meteorol. Soc. 121, 723–739.Google Scholar
Yamanaka, M. D. 1985 Inertial oscillation and symmetric motion induced in an inertio-gravity wave critical layer. J. Met. Soc. Japan 63, 715–737.Google Scholar
Yavneh, I., McWilliams, J. C. & Jeroen Molemaker, M. 2001 Non-axisymmetric instability of centrifugally stable stratified Taylor–Couette flow. J. Fluid Mech. 448, 1–21.Google Scholar