Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-28T16:44:38.391Z Has data issue: false hasContentIssue false
  • English
  • Français

Acoustic-roughness receptivity in subsonic boundary-layer flows over aerofoils

Published online by Cambridge University Press:  19 August 2021

Henrique Raposo Open the ORCID record for Henrique Raposo [Opens in a new window] ,
Shahid Mughal ,
Antoine Bensalah  and
Richard Ashworth
Henrique Raposo*
Affiliation:
Department of Mathematics, Imperial College London, South Kensington Campus, London SW7 2AZ, UK Central Research and Technology, Airbus, Bristol BS99 7AR, UK
Shahid Mughal
Affiliation:
Department of Mathematics, Imperial College London, South Kensington Campus, London SW7 2AZ, UK
Antoine Bensalah
Affiliation:
Central Research and Technology, Airbus, 92130 Issy-les-Moulineaux, France
Richard Ashworth
Affiliation:
Central Research and Technology, Airbus, Bristol BS99 7AR, UK
*
Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

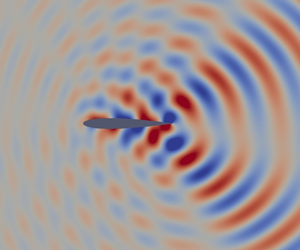
The generation of a viscous–inviscid instability through scattering of an acoustic wave by localised and distributed roughness on the upper surface of a NACA 0012 aerofoil is studied with a time-harmonic compressible adjoint linearised Navier–Stokes approach. This extends previous work by the authors dedicated to flat plate geometries. The key advancement lies in the modelling of the inviscid acoustic field external to the aerofoil boundary layer, requiring a numerical solution of the convected Helmholtz equation in a non-uniform inviscid field to determine the unsteady pressure field on the curved aerofoil surface. This externally imposed acoustic pressure field subsequently drives the acoustic boundary layer, which fundamentally determines the amplitudes of acoustic-roughness receptivity. A study of receptivity in the presence of Gaussian-shaped roughness and sinusoidally distributed roughness at Mach number $M_\infty =0.4$ and Strouhal numbers $\mathcal {S} \approx \{46,69,115\}$ shows the effects of various parameters, most notably angle of attack, angle of incidence of the externally imposed plane acoustic wave and geometry of surface roughness; the latter is varied from viewpoint of its placement on the aerofoil surface and its wavelength. The parametric study suggests that non-parallel effects are quite substantial and that considerable differences arise when using parallel flow theory to estimate the optimal width of Gaussian-shaped roughness elements to provoke the greatest response. Furthermore, receptivity amplitudes for distributed roughness are observed to be generally higher for lower angles of attack, i.e. for less adverse pressure gradients. It is also shown that the boundary layer is more receptive to upstream-travelling acoustic waves.

JFM classification

Boundary Layers: Boundary layer receptivity Acoustics: Aeroacoustics Boundary Layers: Boundary layer stability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 925 , 25 October 2021 , A7
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

†

Present address: Department of Aerospace Engineering, University of Bristol, UK.

References

REFERENCES

Anderson, D., Tannehill, J.C. & Pletcher, R.H. 2016 Computational Fluid Mechanics and Heat Transfer, 3rd edn, pp. 247–270. CRC Press.CrossRefGoogle Scholar
Ayton, L. 2014 Asymptotic approximations for the sound generated by aerofoils in unsteady subsonic flows. PhD thesis, University of Cambridge.CrossRefGoogle Scholar
Bécache, E., Dhia, A.S.B.-B. & Legendre, G. 2004 Perfectly matched layers for the convected helmholtz equation. SIAM J. Numer. Anal. 42 (1), 409–433.CrossRefGoogle Scholar
Becker, J.V. 1940 Boundary-layer transition on the NACA 0012 and 23012 airfoils in the 8-foot high-speed wind tunnel. Tech. Rep. NACA-SR-137. NACA.Google Scholar
Bensalah, A. 2018 Une approche nouvelle de la modélisation mathématique et numérique en aéroacoustique par les équations de goldstein et applications en aéronautique. PhD thesis, Universite Paris Saclay.Google Scholar
Bernots, T. 2014 Receptivity of the boundary layer in transonic flow past an aircraft wing. PhD thesis, Imperial College London.Google Scholar
Cantwell, C.D., et al. 2015 Nektar++: an open-source spectral/hp element framework. Comput. Phys. Commun. 192, 205–219.CrossRefGoogle Scholar
Carpenter, M., Choudhari, M., Li, F., Streett, C. & Chang, C.-L. 2010 Excitation of crossflow instabilities in a swept wing boundary layer. In 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition. AIAA Paper 2010-378.CrossRefGoogle Scholar
Choudhari, M. 1994 a Acoustic receptivity of compressible boundary layers: receptivity by way of surface-temperature variations. Tech. Rep. 4599. NASA, Langley Research Center.Google Scholar
Choudhari, M. 1994 b Roughness-induced generation of crossflow vortices in three-dimensional boundary layers. Theor. Comput. Fluid Dyn. 6 (1), 1–30.CrossRefGoogle Scholar
Choudhari, M. & Streett, C.L. 1992 A finite Reynolds-number approach for the prediction of boundary-layer receptivity in localized regions. Phys. Fluids A 4 (11), 2495–2514.CrossRefGoogle Scholar
Crouch, J.D. 1992 a Localized receptivity of boundary layers. Phys. Fluids A 4 (7), 1408–1414.CrossRefGoogle Scholar
Crouch, J.D. 1992 b Non-localized receptivity of boundary layers. J. Fluid Mech. 244, 567–581.CrossRefGoogle Scholar
Crouch, J.D. 1993 Receptivity of three-dimensional boundary layers. In 31st Aerospace Sciences Meeting. AIAA Paper 1993-74.CrossRefGoogle Scholar
Crouch, J.D. 1994 Influence of pressure gradient on the nonlocalized receptivity of boundary layers. In Nonlinear Instability of Nonparallel Flows (ed. S. P. Lin, W.R.C Phillips & D.T. Valentine), pp. 106–116. Springer.CrossRefGoogle Scholar
Crouch, J.D. & Spalart, P.R. 1995 A study of non-parallel and nonlinear effects on the localized receptivity of boundary layers. J. Fluid Mech. 290, 29–37.CrossRefGoogle Scholar
Dobrinsky, A.Y. 2003 Adjoint analysis for receptivity prediction. PhD thesis, Rice University.Google Scholar
Drela, M. 1989 XFOIL: an analysis and design system for low Reynolds number airfoils. In Low Reynolds Number Aerodynamics, Lecture Notes in Engineering, vol. 54, pp. 1–12. Springer.CrossRefGoogle Scholar
Duck, P.W. 1990 The response of a laminar boundary layer in supersonic flow to small-amplitude progressive waves. J. Fluid Mech. 219, 423–448.CrossRefGoogle Scholar
Duck, P.W., Ruban, A.I. & Zhikharev, C.N. 1996 The generation of Tollmien–Schlichting waves by free-stream turbulence. J. Fluid Mech. 312, 341–371.CrossRefGoogle Scholar
Fuciarelli, D., Reed, H. & Lyttle, I. 2000 Direct numerical simulation of leading-edge receptivity to sound. AIAA J. 38 (7), 1159–1165.CrossRefGoogle Scholar
Gaponov, S.A. 1977 Interaction between a supersonic boundary layer and acoustic disturbances. Fluid Dyn. 12 (6), 858–862.CrossRefGoogle Scholar
Geuzaine, C. & Remacle, J.F. 2009 Gmsh: a 3-D finite element mesh generator with built-in pre-and post-processing facilities. Intl J. Numer. Meth. Fluids 79 (11), 1309–1331.CrossRefGoogle Scholar
Goldstein, M.E. 1983 The evolution of Tollmien–Schlichting waves near a leading edge. J. Fluid Mech. 127, 59–81.CrossRefGoogle Scholar
Goldstein, M.E. 1985 Scattering of acoustic waves into Tollmien–Schlichting waves by small streamwise variations in surface geometry. J. Fluid Mech. 154, 509–529.Google Scholar
Goldstein, M.E. & Hultgren, L.S. 1989 Boundary-layer receptivity to long-wave free-stream disturbances. Annu. Rev. Fluid Mech. 21 (1), 137–166.CrossRefGoogle Scholar
Goldstein, M.E., Leib, S.J. & Cowley, S.J. 1987 Generation of Tollmien–Schlichting waves on interactive marginally separated flows. J. Fluid Mech. 181, 485–517.CrossRefGoogle Scholar
Goldstein, S. 1948 On laminar boundary-layer flow near a position of separation. Q. J. Mech. Appl. Maths 1 (1), 43–69.Google Scholar
Hammerton, P.W. & Kerschen, E.J. 1996 Boundary-layer receptivity for a parabolic leading edge. J. Fluid Mech. 310, 243–267.CrossRefGoogle Scholar
Herr, S., Wörner, A., Würz, W., Rist, U. & Wagner, S. 2002 Experimental/numerical investigation of the influence of a pressure gradient on acoustic roughness receptivity in the boundary layer of an airfoil. In New Results in Numerical and Experimental Fluid Mechanics III (ed. S. Wagner, U. Rist, H.J. Heinemann & R. Hilbig), pp. 231–238. Springer.CrossRefGoogle Scholar
Hill, D.C. 1995 Adjoint systems and their role in the receptivity problem for boundary layers. J. Fluid Mech. 292, 183–204.CrossRefGoogle Scholar
Howe, M.S. 1998 Acoustics of Fluid-Structure Interactions. Cambridge University Press.CrossRefGoogle Scholar
Jiang, L., Shan, H. & Liu, C. 1999 Direct numerical simulation of boundary-layer receptivity for subsonic flow around airfoil. In Recent Advances in DNS and LES (ed. D. Knight & L. Sakell), pp. 203–218. Springer.CrossRefGoogle Scholar
Jones, L.E., Sandberg, R.D. & Sandham, N.D. 2010 Stability and receptivity characteristics of a laminar separation bubble on an aerofoil. J. Fluid Mech. 648, 257–296.CrossRefGoogle Scholar
Kanner, H. & Schetz, J. 1999 The evolution of an acoustic disturbance up to transition in the boundary-layer on an airfoil. In 30th Fluid Dynamics Conference. AIAA Paper 1999-3791.CrossRefGoogle Scholar
Kerschen, E.J. 1991 Linear and nonlinear receptivity to vortical free-stream disturbances. In Boundary Layer Stability and Transition to Turbulence; Proceedings of the Symposium, ASME and JSME Joint Fluids Engineering Conference, pp. 43–48. American Society of Mechanical Engineers.Google Scholar
Mack, L.M. 1984 Boundary layer linear stability theory. In Special Course on Stability and Transition of Laminar Flow, pp. 3.1–3.81. AGARD Rep. 709.Google Scholar
Moore, F.K. 1951 Unsteady laminar boundary-layer flow. Tech. Rep. TM-2471. NACA.Google Scholar
Mughal, M.S. & Ashworth, R. 2013 Uncertainty quantification based receptivity modelling of crossflow instabilities induced by distributed surface roughness in swept wing boundary layers. In 43rd AIAA Fluid Dynamics Conference. AIAA Paper 2013-3106.CrossRefGoogle Scholar
Mughal, S.M. 2006 Stability analysis of complex wing geometries: parabolised stability equations in generalised non-orthogonal coordinates. In 36th AIAA Fluid Dynamics Conference and Exhibit. AIAA Paper 2006-3222.Google Scholar
Nayfeh, A.H. & Ashour, O.N. 1994 Acoustic receptivity of a boundary layer to Tollmien–Schlichting waves resulting from a finite-height hump at finite Reynolds numbers. Phys. Fluids 6 (11), 3705–3716.CrossRefGoogle Scholar
Raposo, H. 2020 Acoustic receptivity in compressible boundary layer flows over aerofoils. PhD thesis, Imperial College London.Google Scholar
Raposo, H., Mughal, S. & Ashworth, R. 2018 Acoustic receptivity and transition modeling of Tollmien–Schlichting disturbances induced by distributed surface roughness. Phys. Fluids 30 (4), 044105.CrossRefGoogle Scholar
Raposo, H., Mughal, S. & Ashworth, R. 2019 An adjoint compressible linearised Navier–Stokes approach to model generation of Tollmien–Schlichting waves by sound. J. Fluid Mech. 877, 105–129.CrossRefGoogle Scholar
Raposo, H., Mughal, S. & Ashworth, R. 2020 On the effects of sound in subsonic boundary layer flows. In Ninth IUTAM Symposium on Laminar-Turbulent Transition (ed. S. Sherwin, P. Schmid & X. Wu), pp. 769–779 (Accepted for publication). Springer.CrossRefGoogle Scholar
Reed, H.L. & Saric, W.S. 2015 Receptivity: the inspiration of Mark Morkovin. In 45th AIAA Fluid Dynamics Conference. AIAA Paper 2015-2471.CrossRefGoogle Scholar
Reshotko, E. 1976 Boundary-layer stability and transition. Annu. Rev. Fluid Mech. 8 (1), 311–349.Google Scholar
Ruban, A.I. 1985 On Tollmien–Schlichting wave generation by sound. In Laminar-Turbulent Transition (ed. V.V. Kozlov), pp. 313–320. Springer.CrossRefGoogle Scholar
Ruban, A.I., Bernots, T. & Kravtsova, M.A. 2016 Linear and nonlinear receptivity of the boundary layer in transonic flows. J. Fluid Mech. 786, 154–189.CrossRefGoogle Scholar
Saric, W., Reed, H. & Kerschen, E. 2002 Boundary layer receptivity to freestream disturbances. Annu. Rev. Fluid Mech. 34, 291–319.CrossRefGoogle Scholar
Saric, W.S. 1994 Physical description of boundary-layer transition: experimental evidence. In Special Course on Progress in Transition Modelling, p. 51. AGARD Rep. 793.Google Scholar
Sayles, R.S. & Thomas, T.R. 1978 Surface topography as a nonstationary random process. Nature 271 (5644), 431–434.CrossRefGoogle Scholar
Schlichting, H. & Gersten, K. 1960 Boundary-Layer Theory, 9th edn, vol. 7. Springer.Google Scholar
Shahriari, N., Bodony, D.J., Hanifi, A. & Henningson, D.S. 2016 Acoustic receptivity simulations of flow past a flat plate with elliptic leading edge. J. Fluid Mech. 800, R2.CrossRefGoogle Scholar
Stewartson, K. 1951 On the impulsive motion of a flat plate in a viscous fluid. Q. J. Mech. Appl. Maths 4 (2), 182–198.CrossRefGoogle Scholar
Streett, C.L. 1998 Direct harmonic linear Navier–Stokes methods for efficient simulation of wave packets. In 36th Aerospace Sciences Meeting and Exhibit. AIAA Paper 1998-784.CrossRefGoogle Scholar
Thomas, C., Mughal, S.M., Gipon, M., Ashworth, R. & Martinez-Cava, A. 2016 Stability of an infinite swept wing boundary layer with surface waviness. AIAA J. 54 (10), 3024–3038.CrossRefGoogle Scholar
Thomas, C., Mughal, S.M., Roland, H., Ashworth, R. & Martinez-Cava, A. 2018 Effect of small surface deformations on the stability of Tollmien–Schlichting disturbances. AIAA J. 56 (6), 2157–2165.CrossRefGoogle Scholar
Van Dyke, M. 1969 Higher-order boundary-layer theory. Annu. Rev. Fluid Mech. 1 (1), 265–292.CrossRefGoogle Scholar
Von Doenhoff, A.E. 1938 A method of rapidly estimating the position of the laminar separation point. Tech. Rep. NACA-TN-671. NACA.Google Scholar
Wiegel, M. & Wlezien, R. 1993 Acoustic receptivity of laminar boundary layers over wavy walls. In 3rd Shear Flow Conference. AIAA Paper 1993-3280.CrossRefGoogle Scholar
Würz, W., Herr, S., Wörner, A., Rist, U., Wagner, S. & Kachanov, Y.S. 2003 Three-dimensional acoustic-roughness receptivity of a boundary layer on an airfoil: experiment and direct numerical simulations. J. Fluid Mech. 478, 135–163.Google Scholar
Zhigulev, V.N. & Fedorov, A.V. 1987 Boundary-layer receptivity to acoustic disturbances. J. Appl. Mech. Tech. Phys. 28 (1), 28–34.CrossRefGoogle Scholar