Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-26T07:41:01.271Z Has data issue: false hasContentIssue false
  • English
  • Français

Vortex shedding frequency in open-channel lateral cavity

Published online by Cambridge University Press:  06 April 2020

C. Perrot-Minot Open the ORCID record for C. Perrot-Minot [Opens in a new window] ,
E. Mignot ,
R. Perkins ,
D. Lopez Open the ORCID record for D. Lopez [Opens in a new window]  and
N. Riviere
C. Perrot-Minot*
Affiliation:
Univ Lyon, Ecole Centrale de Lyon, INSA Lyon, Universite Claude Bernard Lyon I, CNRS, Laboratoire de Mecanique des Fluides et d’Acoustique, UMR 5509, 36 Avenue Guy de Collongue, F-69134, Ecully, France
E. Mignot
Affiliation:
Univ Lyon, INSA Lyon, Ecole Centrale de Lyon, Universite Claude Bernard Lyon I, CNRS, LMFA, UMR 5509, 20 Avenue Albert Einstein, F-69621, Villeurbanne, France
R. Perkins
Affiliation:
Univ Lyon, Ecole Centrale de Lyon, INSA Lyon, Universite Claude Bernard Lyon I, CNRS, Laboratoire de Mecanique des Fluides et d’Acoustique, UMR 5509, 36 Avenue Guy de Collongue, F-69134, Ecully, France
D. Lopez
Affiliation:
Univ Lyon, INSA Lyon, Ecole Centrale de Lyon, Universite Claude Bernard Lyon I, CNRS, LMFA, UMR 5509, 20 Avenue Albert Einstein, F-69621, Villeurbanne, France
N. Riviere
Affiliation:
Univ Lyon, INSA Lyon, Ecole Centrale de Lyon, Universite Claude Bernard Lyon I, CNRS, LMFA, UMR 5509, 20 Avenue Albert Einstein, F-69621, Villeurbanne, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

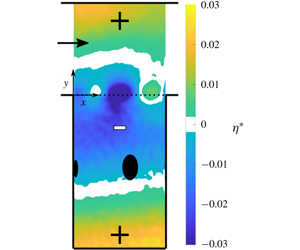
This article aims at predicting the oscillation frequency of open-channel lateral cavities, which are common sheltered zones of riverine environments, and have important ecological impact. Using a theoretical analysis based on an existing model for acoustic cavities and a free-surface lateral cavity experiment, we show that the vortex shedding in the mixing layer between the cavity and the open channel is always constrained by gravity waves even for low Froude numbers ($F<0.6$). This expands previous results from the literature showing the impact of gravity waves on the vortex shedding frequency for high Froude number ($F>0.6$). Measurements of the free-surface oscillation and transverse velocity oscillation frequencies reveal a unique frequency along the mixing layer, equal to the free-surface oscillation frequency anywhere in the cavity. Hence, it is shown that the vortex shedding frequency in an open-channel lateral cavity always equals a match between a natural frequency of the cavity and a solution of the feedback model developed herein for low to moderate Froude numbers.

JFM classification

Geophysical and Geological Flows: River dynamics Vortex Flows: Vortex shedding
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 892 , 10 June 2020 , A25
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alvarez, J. O., Kerschen, E. J. & Tumin, A. 2004 A theoritical model for cavity acoustic resonances in subsonic flow. In 10th AIAA/CEAS Aeroacoustics Conference, p. 2845. American institute of Aeronautics & Astronautics.Google Scholar
Aubourg, Q.2006 Etude experimentale de la turbulence d’ondes a la surface d’un fluide. la theorie de la turbulence faible à l’epreuve de la realite pour les ondes de capillarite et gravite. PhD thesis, Ecole Doctorale Ingenierie Materiaux, Mecanique, Environnement, Energetique, Procedes, Production. Universite Grenoble Alpes, Grenoble.Google Scholar
Bilanin, A. J. & Covert, E. 1973 Estimation of possible excitation frequencies for shallow rectangular cavities. AIAA J. 11 (3), 347–351.CrossRefGoogle Scholar
Charru, F. 2007 Instabilités Hydrodynamiques. Savoirs Actuels.Google Scholar
East, L. F. 1966 Aerodynamically induced resonance in rectangular cavities. J. Sound Vib. 3 (3), 277–287.Google Scholar
Gloefert, X.2009 Cavity noise.Google Scholar
Godreche, C. 1998 Hydrodynamics and Nonlinear Instabilities. Cambridge University Press.CrossRefGoogle Scholar
Ho, C. M. & Nosseir, N. S. 1981 Dynamics of an impinging jet. Part 1. The feedback phenomenon. J. Fluid Mech. 105, 119–142.CrossRefGoogle Scholar
Hussain, A. K. M. F. & Zaman, K. B. M. Q. 1978 The free shear layer tone phenomenon and probe interference. J. Fluid Mech. 87, 349–383.CrossRefGoogle Scholar
Jackson, T. R., Apte, S. V. & Haggerty, R. 2015 Flow structure and mean residence times of lateral cavities in open channel flows: influence of bed roughness and shape. Environ. Fluid Mech. 15, 1069–1100.CrossRefGoogle Scholar
Kegerise, M. A.1999 An experimental investigation of flow-induced cavity oscillations. PhD thesis, Syracuse University.CrossRefGoogle Scholar
Kimura, I. & Hosoda, T. 1997 Fundamental properties of flows in open channels with dead zone. J. Hydraul. Engng ASCE 123 (2), 98–127.CrossRefGoogle Scholar
Lamb, H. 1945 Hydrodynamics. Cambridge University Press.Google Scholar
Larcheveque, L., Sagaut, P., Mary, I., Labbe, O. & Comte, P. 2003 Large-eddy simulation of a compressible flow past a deep cavity. Phys. Fluids.CrossRefGoogle Scholar
Meile, T., Boillat, J.-L. & Schleiss, A. J. 2011 Water-surface oscillations in channels with aximmetric cavities. J. Hydraul Res. 49 (1), 73–81.Google Scholar
Merian, J. R.1828 Ueber die bewegung tropfbarer flüssigkeiten in gefassen [on the motion of drippable liquids in containers]. PhD thesis, Basle.Google Scholar
Mignot, E. & Brevis, W. 2019 Coherent turbulent structures within open-channel lateral cavities. J. Hydraul. Engng ASCE 146 (2).Google Scholar
Mignot, E., Cai, W., Launay, G. & Escauriaza, C. 2016 Coherent turbulent structures at the mixing-interface of a square open-channel cavity. Phys. Fluids 28, 16.CrossRefGoogle Scholar
Mignot, E., Cai, W. & Riviere, N. 2019 Analysis of the transitions between flow patterns in open-channel lateral cavities with increasing aspect ratio. Environ. Fluid Mech. 19 (1), 231–253.CrossRefGoogle Scholar
Plumbee, H. F., Gibson, J. S. & Lassiter, L. W.1962 A theoretical and experimental investigation of the acoustic response of cavities in an aerodynamic flow. Tech. Rep. US Air Force.Google Scholar
Przadka, A., Cabane, B., Pagneux, V., Maurel, A. & Petitjeans, P. 2011 Fourier transform profilometry for water waves: how to achieve clean water attenuation with diffusive reflection at the water surface. Exp. Fluids 52, 519–527.CrossRefGoogle Scholar
Rabinovitch, A. B. 2009 Seiches and Harbor Oscillations, chap. 9. World Scientific Publisher.CrossRefGoogle Scholar
Raupach, M. R., Finnigan, J. J. & Brunet, Y. 1996 Coherent Eddies and Turbulence in Vegetation Canopies: the Mixing Layer Analogy. Kluwer Academic Publishers.Google Scholar
Rockwell, D. & Knisely, C. 1978 The organized nature of flow impingement upon a corner. J. Fluid Mech. 93, 413–432.CrossRefGoogle Scholar
Rossiter, J. E.1964 Wind-tunnel experiments on the flow over rectangular cavities at subsonic and transonic speeds. Tech. Rep. Aeronautical Research Council Reports and Memoranda.Google Scholar
Rowley, C. W., Williams, D. R., Colonius, T., Murray, R. M. & Macmynowski, D. G. 2006 Linear models for control of cavity flow oscillations. J. Fluid Mech. 547, 317–330.CrossRefGoogle Scholar
Sandoval, J., Mignot, E., Mao, L., Bolster, D. & Escauriaza, C. 2019 Field and numerical investigation of transport mechanisms in a surface storage zone. J. Geophys. Res. 124 (4), 938–959.CrossRefGoogle Scholar
Sanjou, M. & Nezu, I. 2013 Hydrodynamic characteristics and related mass-transfer properties in open-channel flows with rectangular embayment zone. Environ. Fluid Mech. 13, 527–555.CrossRefGoogle Scholar
Sarohia, V. 1977 Experimental investigation of oscillations in flows over shallow cavities. AIAA J. 15 (7), 984–991.CrossRefGoogle Scholar
Selfridge, R., Reiss, J. D. & Avital, E. J. 2017 Physically derived synthesis model of a cavity tone. In Proceedings of the 20th International Conference on Digital Audio Effects, pp. 5–9.Google Scholar
Stocker, J. J. 1957 Water Waves, The Mathematical Theory with Applications. Interscience Publishers.Google Scholar
Takeda, M., Ina, H. & Kobayashi, S. 1981 Fourier-transform method of fringe-pattern analysis for computer-based topography and interferometry. J. Opt. Soc. 72 (1), 156–160.CrossRefGoogle Scholar
Tsubaki, R. & Fujita, I. 2006 Surface oscillation in flow past a side cavity using stereoscopic measurement and pod. J. Hydrosci. Hydraul. Engng 24 (2), 41–51.Google Scholar
Tuna, B. A., Tinar, E. & Rockwell, D. 2013 Shallow flow past a cavity: globally coupled oscillations as a function of depth. Exp. Fluids 54, 20.Google Scholar
Wolfinger, M., Ozen, C. A. & Rockwell, D. 2012 Shallow flow past a cavity: coupling with a standing gravity wave. Phys. Fluids 24, 16.CrossRefGoogle Scholar