Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-27T14:39:09.520Z Has data issue: false hasContentIssue false
  • English
  • Français

Velocity profiles, flow structures and scalings in a wide-gap turbulent Taylor–Couette flow

Published online by Cambridge University Press:  13 October 2017

A. Froitzheim Open the ORCID record for A. Froitzheim [Opens in a new window] ,
S. Merbold  and
C. Egbers
A. Froitzheim*
Affiliation:
Department of Aerodynamics and Fluid Mechanics, Brandenburg University of Technology Cottbus-Senftenberg, Siemens-Halske-Ring 14, 03046 Cottbus, Germany
S. Merbold
Affiliation:
Department of Aerodynamics and Fluid Mechanics, Brandenburg University of Technology Cottbus-Senftenberg, Siemens-Halske-Ring 14, 03046 Cottbus, Germany
C. Egbers
Affiliation:
Department of Aerodynamics and Fluid Mechanics, Brandenburg University of Technology Cottbus-Senftenberg, Siemens-Halske-Ring 14, 03046 Cottbus, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Fully turbulent Taylor–Couette flow between independently rotating cylinders is investigated experimentally in a wide-gap configuration ($\unicode[STIX]{x1D702}=0.5$) around the maximum transport of angular momentum. In that regime turbulent Taylor vortices are present inside the gap, leading to a pronounced axial dependence of the flow. To account for this dependence, we measure the radial and azimuthal velocity components in horizontal planes at different cylinder heights using particle image velocimetry. The ratio of angular velocities of the cylinder walls 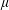$\unicode[STIX]{x1D707}$, where the torque maximum appears, is located in the low counter-rotating regime (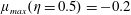$\unicode[STIX]{x1D707}_{max}(\unicode[STIX]{x1D702}=0.5)=-0.2$). This point coincides with the smallest radial gradient of angular velocity in the bulk and the detachment of the neutral surface from the outer cylinder wall, where the azimuthal velocity component vanishes. The structure of the flow is further revealed by decomposing the flow field into its large-scale and turbulent contributions. Applying this decomposition to the kinetic energy, we can analyse the formation process of the turbulent Taylor vortices in more detail. Starting at pure inner cylinder rotation, the vortices are formed and strengthened until 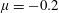$\unicode[STIX]{x1D707}=-0.2$ quite continuously, while they break down rapidly for higher counter-rotation. The same picture is shown by the decomposed Nusselt number, and the range of rotation ratios, where turbulent Taylor vortices can exist, shrinks strongly in comparison to investigations at much lower shear Reynolds numbers. Moreover, we analyse the scaling of the Nusselt number and the wind Reynolds number with the shear Reynolds number, finding a communal transition at approximately 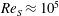$Re_{S}\approx 10^{5}$ from classical to ultimate turbulence with a transitional regime lasting at least up to 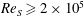$Re_{S}\geqslant 2\times 10^{5}$. Including the axial dispersion of the flow into the calculation of the wind amplitude, we can also investigate the wind Reynolds number as a function of the rotation ratio 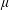$\unicode[STIX]{x1D707}$, finding a maximum in the low counter-rotating regime slightly larger than $\unicode[STIX]{x1D707}_{max}$. Based on our study it becomes clear that the investigation of counter-rotating Taylor–Couette flows strongly requires an axial exploration of the flow.

JFM classification

Turbulent Flows: Rotating turbulence Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 831 , 25 November 2017 , pp. 330 - 357
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andereck, C. D., Liu, S. S. & Swinney, H. L. 1986 Flow regimes in a circular Couette system with independently rotating cylinders. J. Fluid Mech. 164, 155–183.Google Scholar
Bilson, M. & Bremhorst, K. 2007 Direct numerical simulation of turbulent Taylor–Couette flow. J. Fluid Mech. 579, 227–270.Google Scholar
Brauckmann, H. J. & Eckhardt, B. 2013a Direct numerical simulations of local and global torque in Taylor–Couette flow up to Re = 30 000. J. Fluid Mech. 718, 398–427.Google Scholar
Brauckmann, H. J. & Eckhardt, B. 2013b Intermittent boundary layers and torque maxima in Taylor–Couette flow. Phys. Rev. E 87, 033004.Google Scholar
Brauckmann, H. J., Salewsky, M. & Eckhardt, B. 2016 Momentum transport in Taylor–Couette flow with vanishing curvature. J. Fluid Mech. 790, 419–452.Google Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability, 1st edn. Clarendon.Google Scholar
Chouippe, A., Climent, E., Legendre, D. & Gabillet, C. 2014 Numerical simulation of bubble dispersion in turbulent Taylor–Couette flow. Phys. Fluids 26, 043304.CrossRefGoogle Scholar
Diprima, R. C. & Swinney, H. L. 1981 Instabilities and transition in flow between concentric rotating cylinders. In Hydrodynamic Instabilities and the Transition to Turbulence, Topics in Applied Physics, vol. 45, pp. 139–180. Springer.Google Scholar
Dong, S. 2007 Direct numerical simulation of turbulent Taylor–Couette flow. J. Fluid Mech. 587, 373–393.Google Scholar
Dong, S. 2008 Turbulent flow between counter-rotating concentric cylinders: a direct numerical simulation study. J. Fluid Mech. 615, 371–399.Google Scholar
Dong, S. & Zheng, X. 2011 Direct numerical simulation of spiral turbulence. J. Fluid Mech. 668, 150–173.Google Scholar
Donnelly, R. J. 1991 Taylor–Couette flow: the early days. Phys. Today 44, 32–39.Google Scholar
Dubrulle, B., Dauchot, O., Daviaud, F., Longaretti, P.-Y., Richard, D. & Zahn, J.-P. 2005 Stability and turbulent transport in Taylor–Couette flow from analysis of experimental data. Phys. Fluids 17, 095103.Google Scholar
Eckhardt, B., Grossmann, S. & Lohse, D. 2007a Fluxes and energy dissipation in thermal convection and shear flows. Europhys. Lett. 78 (2), 24001.Google Scholar
Eckhardt, B., Grossmann, S. & Lohse, D. 2007b Torque scaling in turbulent Taylor–Couette flow between independently rotating cylinders. J. Fluid Mech. 581, 221–250.Google Scholar
Esser, A. & Grossmann, S. 1996 Analytic expression for Taylor–Couette stability border. Phys. Fluids 8, 1814–1819.Google Scholar
van Gils, D. P. M., Grossmann, S., Sun, C. & Lohse, D. 2012 Optimal Taylor–Couette turbulence. J. Fluid Mech. 706, 118.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23, 045108.Google Scholar
Grossmann, S., Lohse, D. & Sun, C. 2016 High-Reynolds number Taylor–Couette turbulence. Annu. Rev. Fluid Mech. 48, 53–80.Google Scholar
van Hout, R. & Katz, J. 2011 Measurements of mean flow and turbulence characteristics in high-Reynolds number counter-rotating Taylor–Couette flow. Phys. Fluids 23, 105102.Google Scholar
Huisman, S. G., van Gils, D. P. M., Grossmann, S., Sun, C. & Lohse, D. 2012 Ultimate turbulent Taylor–Couette flow. Phys. Rev. Lett. 108, 024501.Google Scholar
Huisman, S. G., van der Veen, R. C. A., Sun, C. & Lohse, D. 2014 Multiple states in highly turbulent Taylor–Couette flow. Nat. Commun. 5, 3820.Google Scholar
Lathrop, D. P., Fineberg, J. & Swinney, H. S. 1992 Transition to shear-driven turbulence in Couette–Taylor flow. Phys. Rev. A 46, 6390–6405.Google Scholar
Lewis, G. S. & Swinney, H. L. 1999 Velocity structure functions, scaling and transitions in high-Reynolds-number Couette–Taylor flow. Phys. Rev. E 59, 5457–5467.Google Scholar
Martinez-Arias, B., Peixinho, J., Crumeyrolle, O. & Mutabazi, I. 2014 Effect of the number of vortices on the torque scaling in Taylor–Couette flow. J. Fluid Mech. 748, 756–767.Google Scholar
Merbold, S., Brauckmann, H. J. & Egbers, C. 2013 Torque measurements and numerical determination in differentially rotating wide gap Taylor–Couette flow. Phys. Rev. E 87, 023014.Google ScholarPubMed
Nobach, H. & Bodenschatz, E. 2009 Limitations of accuracy in PIV due to individual variations of particle image intensities. Exp. Fluids 47, 27–38.Google Scholar
Ostilla, R., Stevens, R. J. A. M., Grossmann, S., Verzicco, R. & Lohse, D. 2013 Optimal Taylor–Couette flow: direct numerical simulations. J. Fluid Mech. 719, 14–46.Google Scholar
Ostilla-Mónico, R., Huisman, S. G., Janninik, T. J. G., van Gils, D. P. M., Verzicco, R., Grossmann, S. & Lohse, D. 2014a Optimal Taylor–Couette flow: radius ratio dependence. J. Fluid Mech. 747, 1–29.Google Scholar
Ostilla-Mónico, R., van der Poel, E. P., Verzicco, R., Grossmann, S. & Lohse, D. 2014b Exploring the phase diagram of fully turbulent Taylor–Couette flow. J. Fluid Mech. 761, 1–26.Google Scholar
Paoletti, M. S. & Lathrop, D. P. 2011 Angular momentum transport in turbulent flow between independently rotating cylinders. Phys. Rev. Lett. 106, 024501.CrossRefGoogle ScholarPubMed
Poncet, S., Viazzo, S., Aubert, A., da Soghe, R. & Bianchini, C. 2013 Turbulent Couette–Taylor flows with endwall effects: a numerical benchmark. Intl J. Heat Fluid Flow 44, 229–238.Google Scholar
Schartmann, E., Ji, H., Burin, M. J. & Goodman, J. 2012 Stability of quasi-Keplerian shear flow in a laboratory experiment. Astron. Astrophys. 543, A94.Google Scholar
van der Veen, R. C. A., Huisman, S. G., Merbold, S., Harlander, U., Egbers, C., Lohse, D. & Sun, C. 2016 Taylor–Couette turbulence at radius ratio 0.5: scaling, flow structures and plumes. J. Fluid Mech. 799, 334–351.Google Scholar
Wendt, F. 1933 Turbulente Strömungen zwischen zwei rotierenden koaxialen Zylindern. Ing.-Arch. 4, 577.Google Scholar