Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-26T15:07:36.877Z Has data issue: false hasContentIssue false
  • English
  • Français

Unsteady control of supersonic turbulent cavity flow based on resolvent analysis

Published online by Cambridge University Press:  19 August 2021

Qiong Liu Open the ORCID record for Qiong Liu [Opens in a new window] ,
Yiyang Sun Open the ORCID record for Yiyang Sun [Opens in a new window] ,
Chi-An Yeh Open the ORCID record for Chi-An Yeh [Opens in a new window] ,
Lawrence S. Ukeiley Open the ORCID record for Lawrence S. Ukeiley [Opens in a new window] ,
Louis N. Cattafesta III Open the ORCID record for Louis N. Cattafesta III [Opens in a new window]  and
Kunihiko Taira Open the ORCID record for Kunihiko Taira [Opens in a new window]
Qiong Liu*
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA90095, USA
Yiyang Sun
Affiliation:
Department of Aerospace Engineering and Mechanics, University of Minnesota, Minneapolis, MN55455, USA
Chi-An Yeh
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA90095, USA
Lawrence S. Ukeiley
Affiliation:
Department of Mechanical and Aerospace Engineering, University of Florida, Gainesville, FL32611, USA
Louis N. Cattafesta III
Affiliation:
Department of Mechanical Engineering, Florida State University, Tallahassee, FL32310, USA
Kunihiko Taira
Affiliation:
Department of Mechanical and Aerospace Engineering, University of California, Los Angeles, CA90095, USA
*
Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

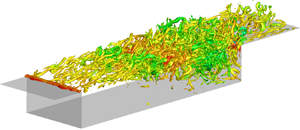
We use resolvent analysis to develop a physics-based, open-loop, unsteady control strategy to attenuate pressure fluctuations in turbulent flow over a rectangular cavity with a length-to-depth ratio of $6$ at a Mach number of $1.4$ and a Reynolds number based on cavity depth of $10\,000$. Large-eddy simulations (LES) of the baseline uncontrolled flow reveal the dominance of Rossiter modes II and IV that generate high-amplitude unsteadiness via trailing-edge impingement and oblique shock waves that obstruct the free stream. To suppress the oscillations, we introduce three-dimensional unsteady blowing along the cavity leading edge. We leverage resolvent analysis as a linear model with respect to the baseline flow to guide the selections of the optimal spanwise wavenumber and frequency of the unsteady actuation input for a fixed momentum coefficient of 0.02. Instead of choosing the most amplified resolvent forcing modes, we seek a disturbance that yields sustained amplification of the primary response mode-based kinetic energy distribution over the entire cavity length. This necessary but not sufficient guideline for effective mean flow modification is evaluated using LES of the controlled cavity flows. The most effective control case reduces the pressure root mean square level up to $52\,\%$ along cavity walls relative to the baseline and is approximately twice that achievable by comparable steady blowing. Dynamic mode decomposition on the controlled flows confirms that the optimal actuation input indeed suppresses the formation of the large-scale Rossiter modes. It is expected that the present flow control guideline derived from resolvent analysis will also be applicable at higher Reynolds numbers with the aid of physical insights and further validation.

JFM classification

Flow Control: Flow Control Compressible Flows: Compressible Flows Instability: Instability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 925 , 25 October 2021 , A5
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

†

Present address: Department of Mechanical and Aerospace Engineering, The Ohio State University, Columbus, OH 43210, USA.

‡

Present address: Department of Mechanical and Aerospace Engineering, Syracuse University, Syracuse, NY 13244, USA.

References

REFERENCES

Bechara, W., Bailly, C., Lafon, P. & Candel, S.M. 1994 Stochastic approach to noise modeling for free turbulent flows. AIAA J. 32 (3), 455–463.CrossRefGoogle Scholar
Beresh, S.J., Wagner, J.L. & Casper, K.M. 2016 Compressibility effects in the shear layer over a rectangular cavity. J. Fluid Mech. 808, 116–152.CrossRefGoogle Scholar
Brès, G.A. & Colonius, T. 2008 Three-dimensional instabilities in compressible flow over open cavities. J. Fluid Mech. 599, 309–339.CrossRefGoogle Scholar
Brès, G.A., Ham, F.E., Nichols, J.W. & Lele, S.K. 2017 Unstructured large-eddy simulations of supersonic jets. AIAA J. 55 (4), 1164–1184.CrossRefGoogle Scholar
Cattafesta, L.N. & Sheplak, M. 2011 Actuators for active flow control. Annu. Rev. Fluid Mech. 43, 247–272.CrossRefGoogle Scholar
Cattafesta, L.N., Song, Q., Williams, D.R., Rowley, C.W. & Alvi, F.S. 2008 Active control of flow-induced cavity oscillations. Prog. Aerosp. Sci. 44 (7–8), 479–502.CrossRefGoogle Scholar
Chu, B.-T. 1965 On the energy transfer to small disturbances in fluid flow (part I). Acta Mech. 1 (3), 215–234.CrossRefGoogle Scholar
Colonius, T. 2001 An overview of simulation, modeling, and active control of flow/acoustic resonance in open cavities. AIAA Paper 2001-0076.Google Scholar
De Vicente, J., Basley, J., Meseguer-Garrido, F., Soria, J. & Theofilis, V. 2014 Three-dimensional instabilities over a rectangular open cavity: from linear stability analysis to experimentation. J. Fluid Mech. 748, 189–220.CrossRefGoogle Scholar
Elimelech, Y., Vasile, J. & Amitay, M. 2011 Secondary flow structures due to interaction between a finite-span synthetic jet and a 3-D cross flow. Phys. Fluids 23 (9), 094104.Google Scholar
Faure, T.M., Adrianos, P., Lusseyran, F. & Pastur, L. 2007 Visualizations of the flow inside an open cavity at medium range Reynolds numbers. Exp. Fluids 42 (2), 169–184.CrossRefGoogle Scholar
Faure, T.M., Pastur, L., Lusseyran, F., Fraigneau, Y. & Bisch, D. 2009 Three-dimensional centrifugal instabilities development inside a parallelepipedic open cavity of various shape. Exp. Fluids 47 (3), 395–410.CrossRefGoogle Scholar
George, B., Ukeiley, L.S., Cattafesta, L.N. & Taira, K. 2015 Control of three-dimensional cavity flow using leading-edge slot blowing. AIAA Paper 2015-1059.CrossRefGoogle Scholar
Gómez, F., Blackburn, H.M., Rudman, M., McKeon, B.J., Luhar, M., Moarref, R. & Sharma, A.S. 2014 On the origin of frequency sparsity in direct numerical simulations of turbulent pipe flow. Phys. Fluids 26 (10), 101703.CrossRefGoogle Scholar
Heller, H.H., Holmes, D.G. & Covert, E.E. 1971 Flow-induced pressure oscillations in shallow cavities. J. Sound Vib. 18 (4), 545–553.Google Scholar
Jovanović, M.R. 2004 Modeling, analysis, and control of spatially distributed systems. PhD thesis, University of California, Santa Barbara.Google Scholar
Jovanović, M.R. & Bamieh, B. 2005 Componentwise energy amplification in channel flows. J. Fluid Mech. 534, 145–183.Google Scholar
Khalighi, Y., Ham, F., Moin, P., Lele, S.K. & Schlinker, R.H. 2011 Noise prediction of pressure-mismatched jets using unstructured large eddy simulation. In ASME 2011 Turbo Expo: Turbine Technical Conference and Exposition, pp. 381–387.Google Scholar
Khodkar, M.A. & Taira, K. 2020 Phase-synchronization properties of laminar cylinder wake for periodic external forcings. J. Fluid Mech. 904, R1.CrossRefGoogle Scholar
Krishnamurty, K. 1955 Acoustic radiation from two-dimensional rectangular cutouts in aerodynamic surfaces. Tech. Rep. 3487. NACA Tech. Note.Google Scholar
Larchevêque, L., Sagaut, P. & Labbé, O. 2007 Large-eddy simulation of a subsonic cavity flow including asymmetric three-dimensional effects. J. Fluid Mech. 577, 105–126.Google Scholar
Lawson, S.J. & Barakos, G.N. 2011 Review of numerical simulations for high-speed, turbulent cavity flows. Prog. Aerosp. Sci. 47 (3), 186–216.Google Scholar
Leclercq, C., Demourant, F., Poussot-Vassal, C. & Sipp, D. 2019 Linear iterative method for closed-loop control of quasiperiodic flows. J. Fluid Mech. 868, 26–65.CrossRefGoogle Scholar
Liu, Q., Gómez, F. & Theofilis, V. 2016 Linear instability analysis of low-$Re$ incompressible flow over a long rectangular finite-span open cavity. J. Fluid Mech. 799, R2.CrossRefGoogle Scholar
Lusk, T., Cattafesta, L.N. & Ukeiley, L.S. 2012 Leading edge slot blowing on an open cavity in supersonic flow. Exp. Fluids 53 (1), 187–199.CrossRefGoogle Scholar
Maull, D.J. & East, L.F. 1963 Three-dimensional flow in cavities. J. Fluid Mech. 16 (4), 620–632.CrossRefGoogle Scholar
McGrath, S.F. & Shaw, L.L. 1996 Active control of shallow cavity acoustic resonance. AIAA Paper 96-1949.CrossRefGoogle Scholar
Mcgregor, O.W. & White, R.A. 1970 Drag of rectangular cavities in supersonic and transonic flow including the effects of cavity resonance. AIAA J. 8 (11), 1959–1964.CrossRefGoogle Scholar
McKeon, B.J. 2017 The engine behind (wall) turbulence: perspectives on scale interactions. J. Fluid Mech. 817, P1.CrossRefGoogle Scholar
McKeon, B.J. 2020 A basis for flow modelling. J. Fluid Mech. 904, F1.CrossRefGoogle Scholar
McKeon, B.J. & Sharma, A.S. 2010 A critical-layer framework for turbulent pipe flow. J. Fluid Mech. 658, 336–382.CrossRefGoogle Scholar
Morra, P., Semeraro, O., Henningson, D.S. & Cossu, C. 2019 On the relevance of Reynolds stresses in resolvent analyses of turbulent wall-bounded flows. J. Fluid Mech. 867, 969–984.CrossRefGoogle Scholar
Murray, N., Sällström, E. & Ukeiley, L.S. 2009 Properties of subsonic open cavity flow fields. Phys. Fluids 21 (9), 095103.CrossRefGoogle Scholar
Nakashima, S., Fukagata, K. & Luhar, M. 2017 Assessment of suboptimal control for turbulent skin friction reduction via resolvent analysis. J. Fluid Mech. 828, 496–526.CrossRefGoogle Scholar
Picella, F., Loiseau, J.-C., Lusseyran, F., Robinet, J.-C., Cherubini, S. & Pastur, L. 2018 Successive bifurcations in a fully three-dimensional open cavity flow. J. Fluid Mech. 844, 855–877.CrossRefGoogle Scholar
Pickering, E.M., Rigas, G., Sipp, D., Schmidt, O.T. & Colonius, T. 2019 Eddy viscosity for resolvent-based jet noise models. AIAA paper 2019-2454.Google Scholar
Plumblee, H.E., Gibson, J.S. & Lassiter, L.W. 1962 A theoretical and experimental investigation of the acoustic response of cavities in an aerodynamic flow. Tech. Rep. Lockheed Aircraft Corp Marietta GA.Google Scholar
Reynolds, W.C. & Hussain, A.K.M.F. 1972 The mechanics of an organized wave in turbulent shear flow. Part 3. Theoretical models and comparisons with experiments. J. Fluid Mech. 54 (2), 263–288.Google Scholar
Ribeiro, J.H.M., Yeh, C.-A. & Taira, K. 2020 Randomized resolvent analysis. Phys. Rev. Fluids 5, 033902.CrossRefGoogle Scholar
Rizzetta, D.P. & Visbal, M.R. 2003 Large-eddy simulation of supersonic cavity flowfields including flow control. AIAA J. 41 (8), 1452–1462.CrossRefGoogle Scholar
Rockwell, D. & Naudascher, E. 1979 Self-sustained oscillations of impinging free shear layers. Annu. Rev. Fluid Mech. 11 (1), 67–94.CrossRefGoogle Scholar
Rossiter, J.E. 1964 Wind-tunnel experiments on the flow over rectangular cavities at subsonic and transonic speeds. Tech. Rep. 3438. Aeronautical Research Council Reports and Memoranda.Google Scholar
Rowley, C.W., Mezić, I., Bagheri, S., Schlatter, P. & Henningson, D.S. 2009 Spectral analysis of nonlinear flows. J. Fluid Mech. 641, 115–127.CrossRefGoogle Scholar
Rowley, C.W. & Williams, D.R. 2006 Dynamics and control of high Reynolds number flow over open cavities. Annu. Rev. Fluid Mech. 38, 251–276.CrossRefGoogle Scholar
Sarno, R.L. & Franke, M.E. 1994 Suppression of flow-induced pressure oscillations in cavities. J. Aircr. 31 (1), 90–96.CrossRefGoogle Scholar
Schmid, P.J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.CrossRefGoogle Scholar
Schmid, P.J. & Henningson, D.S. 2012 Stability and Transition in Shear Flows. Springer.Google Scholar
Schmidt, O.T. & Colonius, T. 2020 Guide to spectral proper orthogonal decomposition. AIAA J. 58 (3), 1023–1033.CrossRefGoogle Scholar
Schmidt, O.T., Towne, A., Colonius, T., Cavalieri, A.V.G., Jordan, P. & Brès, G.A. 2017 a Wavepackets and trapped acoustic modes in a turbulent jet: coherent structure eduction and global stability. J. Fluid Mech. 825, 1153–1181.CrossRefGoogle Scholar
Schmidt, O.T., Towne, A., Rigas, G., Colonius, T. & Brès, G.A. 2017 b Spectral analysis of jet turbulence. J. Fluid Mech. 855 (25), 953–982.CrossRefGoogle Scholar
Shaw, L. 1998 Active control for cavity acoustics. AIAA Paper 1998-2347.CrossRefGoogle Scholar
Sun, Y., Liu, Q., Cattafesta, L.N., Ukeiley, L.S. & Taira, K. 2019 Effects of sidewalls and leading-edge blowing on flows over long rectangular cavities. AIAA J. 57 (1), 106–119.CrossRefGoogle Scholar
Sun, Y., Liu, Q., Cattafesta, L.N., Ukeiley, L.S. & Taira, K. 2020 Resolvent analysis of compressible laminar and turbulent cavity flows. AIAA J. 58 (3), 1046–1055.CrossRefGoogle Scholar
Sun, Y., Taira, K., Cattafesta, L.N. & Ukeiley, L.S. 2017 a Biglobal instabilities of compressible open-cavity flows. J. Fluid Mech. 826, 270–301.CrossRefGoogle Scholar
Sun, Y., Taira, K., Cattafesta, L.N. & Ukeiley, L.S. 2017 b Spanwise effects on instabilities of compressible flow over a long rectangular cavity. Theor. Comput. Fluid Dyn. 31, 555–565.CrossRefGoogle Scholar
Taira, K., Brunton, S.L., Dawson, S., Rowley, C.W., Colonius, T., McKeon, B.J., Schmidt, O.T., Gordeyev, S., Theofilis, V. & Ukeiley, L.S. 2017 Modal analysis of fluid flows: an overview. AIAA J. 55 (12), 4013–4041.CrossRefGoogle Scholar
Taira, K., Hemati, M.S., Brunton, S.L., Sun, Y., Duraisamy, K., Bagheri, S., Dawson, S.T.M. & Yeh, C.-A. 2020 a Modal analysis of fluid flows: applications and outlook. AIAA J. 58 (3), 998–1022.CrossRefGoogle Scholar
Taira, K., Hemati, M.S. & Ukeiley, L.S. 2020 b Modal analysis of fluid flow: introduction to the virtual collection. AIAA J. 58 (3), 991–993.CrossRefGoogle Scholar
Taira, K. & Nakao, H. 2018 Phase-response analysis of synchronization for periodic flows. J. Fluid Mech. 846, R2.CrossRefGoogle Scholar
Theofilis, V. 2011 Global linear instability. Annu. Rev. Fluid Mech. 43, 319–352.CrossRefGoogle Scholar
Toro, E.F., Spruce, M. & Speares, W. 1994 Restoration of the contact surface in the HLL-Riemann solver. Shock Waves 4, 25–34.CrossRefGoogle Scholar
Trefethen, L.N. & Embree, M. 2005 Spectra and Pseudospectra: The Behavior of Nonnormal Matrices And Operators. Princeton University Press.CrossRefGoogle Scholar
Trefethen, L.N., Trefethen, A.E., Reddy, S.C. & Driscoll, T.A. 1993 Hydrodynamic stability without eigenvalues. Science 261 (5121), 578–584.CrossRefGoogle ScholarPubMed
Ukeiley, L.S., Ponton, M.K., Seiner, J.M. & Jansen, B. 2004 Suppression of pressure loads in cavity flows. AIAA J. 42 (1), 70–79.CrossRefGoogle Scholar
Vakili, A.D. & Gauthier, C. 1994 Control of cavity flow by upstream mass-injection. J. Aircr. 31 (1), 169–174.CrossRefGoogle Scholar
Vreman, A.W. 2004 An eddy-viscosity subgrid-scale model for turbulent shear flow: algebraic theory and applications. Phys. Fluids 16 (10), 3670–3681.CrossRefGoogle Scholar
Williams, D.R., Cornelius, D. & Rowley, C.W. 2007 Supersonic cavity response to open-loop forcing. In Active Flow Control, pp. 230–243. Springer.CrossRefGoogle Scholar
Yeh, C.-A., Benton, S.I., Taira, K. & Garmann, D.J. 2020 Resolvent analysis of an airfoil laminar separation bubble at $Re = 500, 000$. Phys. Rev. Fluids 5, 033902.CrossRefGoogle Scholar
Yeh, C.-A., Gopalakrishnan Meena, M. & Taira, K. 2021 Network broadcast analysis and control of turbulent flows. J. Fluid Mech. 910, A15.Google Scholar
Yeh, C.-A. & Taira, K. 2019 Resolvent-analysis-based design of airfoil separation control. J. Fluid Mech. 867, 572–610.CrossRefGoogle Scholar
Zhang, Y., Sun, Y., Arora, N., Cattafesta, L.N., Taira, K. & Ukeiley, L.S. 2019 Suppression of cavity flow oscillations via three-dimensional steady blowing. AIAA J. 57 (1), 90–105.CrossRefGoogle Scholar