Published online by Cambridge University Press: 20 March 2019
The flow around a sphere descending at constant speed in a very strongly stratified fluid ( $Fr\lesssim 0.2$) is investigated by the shadowgraph method and particle image velocimetry. Unlike the flow under moderately strong stratification (
$Fr\lesssim 0.2$) is investigated by the shadowgraph method and particle image velocimetry. Unlike the flow under moderately strong stratification (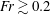 $Fr\gtrsim 0.2$), which supports a thin cylindrical jet, the flow generates an unstable jet, which often develops into turbulence. The transition from a stable jet to an unstable jet occurs for a sufficiently low Froude number
$Fr\gtrsim 0.2$), which supports a thin cylindrical jet, the flow generates an unstable jet, which often develops into turbulence. The transition from a stable jet to an unstable jet occurs for a sufficiently low Froude number 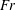 $Fr$ that satisfies
$Fr$ that satisfies 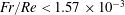 $Fr/Re<1.57\times 10^{-3}$. The Froude number
$Fr/Re<1.57\times 10^{-3}$. The Froude number 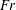 $Fr$ here is in the range of
$Fr$ here is in the range of  $0.0157<Fr<0.157$ or lower, while the Reynolds number
$0.0157<Fr<0.157$ or lower, while the Reynolds number 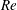 $Re$ is in the range of
$Re$ is in the range of 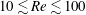 $10\lesssim Re\lesssim 100$ for which the homogeneous fluid shows steady and axisymmetric flows. Since the radius of the jet can be estimated by the primitive length scale of the stratified fluid, i.e.
$10\lesssim Re\lesssim 100$ for which the homogeneous fluid shows steady and axisymmetric flows. Since the radius of the jet can be estimated by the primitive length scale of the stratified fluid, i.e. 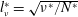 $l_{\unicode[STIX]{x1D708}}^{\ast }=\sqrt{\unicode[STIX]{x1D708}^{\ast }/N^{\ast }}$ or
$l_{\unicode[STIX]{x1D708}}^{\ast }=\sqrt{\unicode[STIX]{x1D708}^{\ast }/N^{\ast }}$ or 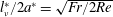 $l_{\unicode[STIX]{x1D708}}^{\ast }/2a^{\ast }=\sqrt{Fr/2Re}$, this predicts that the jet becomes unstable when it becomes thinner than approximately
$l_{\unicode[STIX]{x1D708}}^{\ast }/2a^{\ast }=\sqrt{Fr/2Re}$, this predicts that the jet becomes unstable when it becomes thinner than approximately 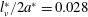 $l_{\unicode[STIX]{x1D708}}^{\ast }/2a^{\ast }=0.028$, where
$l_{\unicode[STIX]{x1D708}}^{\ast }/2a^{\ast }=0.028$, where 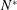 $N^{\ast }$ is the Brunt–Väisälä frequency,
$N^{\ast }$ is the Brunt–Väisälä frequency, 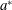 $a^{\ast }$ the radius of the sphere and
$a^{\ast }$ the radius of the sphere and 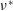 $\unicode[STIX]{x1D708}^{\ast }$ the kinematic viscosity of the fluid. The instability begins when the boundary-layer thickness becomes thin, and the disturbances generated by shear instabilities would be transferred into the jet. When the flow is marginally unstable, two unstable states, i.e. a meandering jet and a turbulent jet, can appear. The meandering jet is thin with a high vertical velocity, while the turbulent jet is broad with a much smaller velocity. The meandering jet may persist for a long time, or develop into a turbulent jet in a short time. When the instability is sufficiently strong, only the turbulent jet could be observed.
$\unicode[STIX]{x1D708}^{\ast }$ the kinematic viscosity of the fluid. The instability begins when the boundary-layer thickness becomes thin, and the disturbances generated by shear instabilities would be transferred into the jet. When the flow is marginally unstable, two unstable states, i.e. a meandering jet and a turbulent jet, can appear. The meandering jet is thin with a high vertical velocity, while the turbulent jet is broad with a much smaller velocity. The meandering jet may persist for a long time, or develop into a turbulent jet in a short time. When the instability is sufficiently strong, only the turbulent jet could be observed.