Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-27T01:07:35.214Z Has data issue: false hasContentIssue false
  • English
  • Français

Two-dimensional response of a floating ice plate to a line load moving at variable speed

Published online by Cambridge University Press:  09 March 2022

Roger J. Hosking  and
Fausto Milinazzo Open the ORCID record for Fausto Milinazzo [Opens in a new window]
Roger J. Hosking
Affiliation:
School of Mathematical Sciences, University of Adelaide, AdelaideSA5005, Australia
Fausto Milinazzo*
Affiliation:
Department of Mathematics, Royal Roads Military College, FMO Victoria, BCV0S 1B0, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

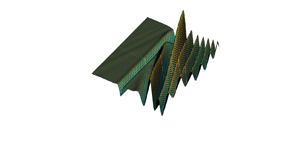
We significantly extend the results of Miles & Sneyd (J. Fluid Mech., vol. 497, 2003, pp. 435–439) for an accelerating line load on a floating ice plate in their simple linear mathematical model by proceeding to numerical calculations for the response due to a decelerating load. Our results show: (i) how the deflections produced by an impulsively started steadily moving line load begin to develop and eventually approach the well-known steady load-speed-dependent quasi-static and wave-like forms, including above the shallow water gravity wave speed where the shadow zone evolves; (ii) the singularity in the deflection predicted in the simple linear model when the load moves steadily is indeed avoided by a uniformly accelerating load, where the magnitude of the deflection continually increases and its maximum lags a little further behind as the load moves through the critical speed and beyond; (iii) there is also no singularity in the deflection due to a uniformly decelerating load, but whereas the response from a subcritical starting speed is preserved and travels with the load, the magnitude of the deflection may become quite large near a load starting from supercritical speed and approaching rest, which is attributed to constructive interference (reinforcement) as erstwhile trailing, predominantly gravity, waves catch up with the load. While this reinforcement poses no risk to Hercules transport aircraft landing on the thick sea ice at McMurdo Sound, it can account for the reported rapid sinking of the detached cockpit shortly after it came to rest in the 1974 Lockheed Electra aircraft crash in the Canadian Arctic.

JFM classification

Waves/Free-surface Flows: Elastic waves Waves/Free-surface Flows: Surface gravity waves
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 938 , 10 May 2022 , A2
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abramowitz, M. & Stegun, I. (Eds) 1964 Handbook of Mathematical Functions. Applied Mathematics Series, vol. 55. National Bureau of Standards.Google Scholar
Babaei, H., van der Sanden, J., Short, N. & Barrette, P. 2016 Lake ice cover deflection induced by moving vehicles: comparing theoretical results with satellite observations. In TAC 2016: Efficient Transportation – Managing the Demand – 2016 Conference and Exhibition of the Transportation Association of Canada..Google Scholar
Beltaos, S. 1981 Field studies on the response of ice sheets to moving loads. Can. J. Civ. Engng 8, 1–8.CrossRefGoogle Scholar
Das, S., Sahoo, T. & Meylan, M. 2018 Flexural-gravity wave dynamics in two-layer fluid: blocking and dead water analogue. J. Fluid Mech. 854, 121–145.CrossRefGoogle Scholar
Dinvay, E., Kalisch, H. & Parau, E. 2019 Fully dispersive models for moving loads on ice sheets. J. Fluid Mech. 876, 122–149.CrossRefGoogle Scholar
Goodman, D., Wadhams, P. & Squire, V. 1980 The flexural response of a tabular ice island to ocean swell. Ann. Glaciol. 1, 23–27.CrossRefGoogle Scholar
Guyenne, P. & Parau, E. 2014 Finite-depth effects on solitary waves in a floating ice sheet. J. Fluids Struct. 49, 242–262.CrossRefGoogle Scholar
Matiushina, A., Pogorelova, A. & Kozin, V.M. 2016 Effect of impact load on the ice cover during the landing of an airplane. Intl J. Offshore Polar Engng 26, 6–12.Google Scholar
Miles, J. & Sneyd, A. 2003 The response of a floating ice sheet to an accelerating line load. J. Fluid Mech. 497, 435–439.CrossRefGoogle Scholar
Nugroho, W., Wang, K., Hosking, R. & Milinazzo, F. 1999 Time-dependent response of a floating flexible plate to an impulsively started steadily moving load. J. Fluid Mech. 381, 337–355.CrossRefGoogle Scholar
Parau, E. & Dias, F. 2002 Nonlinear effects in the response of a floating ice plate to a moving load. J. Fluid Mech. 460, 281–305.CrossRefGoogle Scholar
Pogorelova, A. 2008 Wave resistance of an air-cushion vehicle in unsteady motion over an ice sheet. J. Appl. Mech. Tech. Phys. 49, 71–79.CrossRefGoogle Scholar
Squire, V., Hosking, R., Kerr, A. & Langhorne, P. 1996 Moving Loads on Ice Plates. Kluwer Academic Publishers. Also Arkiv, Springer (2012).CrossRefGoogle Scholar
Stevenson, W. 1976 ASN Aircraft accident Lockheed L-188PF Electra CF-PAB Rea Point Airfield, NT (YOX), Technical report, Flight Safety Foundation, Edmonton, AB. www.flightsafety.org.Google Scholar
Van der Sanden, J. & Short, N. 2017 Radar satellites measure ice cover displacements induced by moving vehicles. Cold Reg. Sci. Technol. 133, 56–62.CrossRefGoogle Scholar
Wadhams, P. 1997 Review of “moving loads on ice plates”, Kluwer (1996). Polar Record 33, 73–74.CrossRefGoogle Scholar
Wang, K., Hosking, R. & Milinazzo, F. 2004 Time-dependent response of a floating viscoelastic plate to an impulsively started moving load. J. Fluid Mech. 521, 295–317.CrossRefGoogle Scholar
Supplementary material: File

Hosking and Milinazzo supplementary material

Hosking and Milinazzo supplementary material

Download Hosking and Milinazzo supplementary material(File)
File 172.5 KB