Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-09T08:40:28.324Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent pipe flow downstream of a $90{{}^\circ} $ bend

Published online by Cambridge University Press:  29 October 2013

Leo H. O. Hellström ,
Metodi B. Zlatinov ,
Guangjun Cao  and
Alexander J. Smits
Leo H. O. Hellström*
Affiliation:
Mechanical and Aerospace Engineering, Princeton University, Princeton, NJ 08544, USA
Metodi B. Zlatinov
Affiliation:
Mechanical and Aerospace Engineering, Princeton University, Princeton, NJ 08544, USA
Guangjun Cao
Affiliation:
CARDC, PO Box 211 Mianyang, Sichuan 621000, PR China
Alexander J. Smits
Affiliation:
Mechanical and Aerospace Engineering, Princeton University, Princeton, NJ 08544, USA Mechanical and Aerospace Engineering, Monash University, VIC 3800, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Time-resolved stereoscopic PIV was used to investigate the curvature-induced structures downstream of a $90{{}^\circ} $ bend at Reynolds numbers between 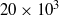$20\times 1{0}^{3} $ and 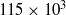$115\times 1{0}^{3} $. Data were taken at three downstream locations to investigate the evolution of the structures. Snapshot proper orthogonal decomposition (POD) analysis shows that the most energetic structure is not the well-known Dean motion but a bimodal single cell structure with alternating direction of rotation, called the ‘swirl switching’ mode. The strengths of the Dean motion and the swirl-switching structures are similar, indicating that the difference in energy is related to their duration of occurrence, where the Dean motion is associated with a comparatively rapid transition between the two states in the swirl switching mode.

JFM classification

Nonlinear Dynamical Systems: Low-dimensional models Turbulent Flows: Turbulent boundary layers Vortex Flows: Vortex dynamics
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 735 , 25 November 2013 , R7
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anwer, M. & So, R. M. C. 1993 Swirling turbulent flow through a curved pipe. Part 1. Effect of swirl and bend curvature. Exp. Fluids 14 (1), 85–96.CrossRefGoogle Scholar
Anwer, M., So, R. M. C. & Lai, Y. G. 1989 Perturbation by and recovery from bend curvature of a fully developed turbulent pipe flow. Phys. Fluids A 1 (8), 1387–1397.CrossRefGoogle Scholar
Berger, S. A., Talbot, L. & Yao, L.-S. 1983 Flow in curved pipes. Annu. Rev. Fluid Mech. 15, 461–512.CrossRefGoogle Scholar
Boiron, O., DelPlano, V. & Pelissier, R. 2007 Experimental and numerical studies on the starting effect on the secondary flow in a bend. J. Fluid Mech. 574, 109–129.CrossRefGoogle Scholar
Brücker, C. 1998 A time-recording DPIV-study of the swirl switching effect in a $90{{}^\circ} $ bend flow. In Proceedings of the 8th International Symposium on Flow Visualization, 1–4 September 1998, Sorrento, Italy. Optimage Ltd.Google Scholar
Dean, W. R. 1928 The streamline motion of fluid in a curved pipe. Phil. Mag. 30, 673–693.CrossRefGoogle Scholar
Enayet, M. M., Gibson, M. M., Taylor, A. M. K. P. & Yianneskis, M. 1982 Laser-Doppler measurements of laminar and turbulent flow in a pipe bend. Intl J. Heat Fluid Flow 3, 213–219.CrossRefGoogle Scholar
Hawthorne, W. R. 1951 Secondary circulation in fluid flow. Proc. R. Soc. Lond. A 206, 374–387.Google Scholar
Hellström, L. H. O., Sinha, A. & Smits, A. J. 2011a Visualizing the very-large-scale motions in turbulent pipe flow. Phys. Fluids 23, 011703.CrossRefGoogle Scholar
Hellström, L. H. O., Zlatinov, M. B., Smits, A. J. & Cao, G. 2011 b Turbulent pipe flow through a $90{{}^\circ} $ bend. In Proceedings of the 7th International Symposium on Turbulence and Shear Flow Phenomena, 28–31 July 2011, Ottawa, Canada.CrossRefGoogle Scholar
Kalpakli, V. A. & Örlü, 2013 Turbulent pipe flow downstream a $90{{}^\circ} $ pipe bend with and without superimposed swirl. Intl J. Heat Fluid Flow 41, 103–111.CrossRefGoogle Scholar
Meyer, K. E., Pedersen, J. M. & Ozcan, O. 2007 A turbulent jet in crossflow analysed with proper orthogonal decomposition. J. Fluid Mech. 583, 199–228.CrossRefGoogle Scholar
Rütten, F., Schröder, W. & Meinke, M. 2005 Large-eddy simulation of low frequency oscillations of the Dean vortices in turbulent pipe bend flows. Phys. Fluids 17, 035107.CrossRefGoogle Scholar
Sakakibara, J. & Machida, N. 2012 Measurement of turbulent flow upstream and downstream of a circular pipe bend. Phys. Fluids 24, 041702.CrossRefGoogle Scholar
Sirovich, L. 1987 Turbulence and the dynamics of coherent structures. Part 1. Coherent structures. Q. Appl. Maths 45, 561–571.CrossRefGoogle Scholar
So, R. M. C. & Anwer, M. 1993 Swirling turbulent flow through a curved pipe. Part 2. Recovery from swirl and bend curvature. Exp. Fluids 14 (3), 169–177.CrossRefGoogle Scholar
Sudo, K., Sumida, M. & Hibara, H. 1998 Experimental investigation on turbulent flow in a circular-sectioned 90-degree bend. Exp. Fluids 25, 42–49.CrossRefGoogle Scholar
Tunstall, M. J. & Harvey, J. K. 1968 On the effect of a sharp bend in a fully developed turbulent pipe-flow. J. Fluid Mech. 34, 595–608.CrossRefGoogle Scholar
Zagarola, M. V. & Smits, A. J. 1998 Mean-flow scaling of turbulent pipe flow. J. Fluid Mech. 373, 33–79.CrossRefGoogle Scholar