Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Coleman, G. N.
Garbaruk, A.
and
Spalart, P. R.
2015.
Direct Numerical Simulation, Theories and Modelling of Wall Turbulence with a Range of Pressure Gradients.
Flow, Turbulence and Combustion,
Vol. 95,
Issue. 2-3,
p.
261.
Rawat, Subhandu
Cossu, Carlo
Hwang, Yongyun
and
Rincon, François
2015.
On the self-sustained nature of large-scale motions in turbulent Couette flow.
Journal of Fluid Mechanics,
Vol. 782,
Issue. ,
p.
515.
Orlandi, Paolo
Bernardini, Matteo
and
Pirozzoli, Sergio
2015.
Poiseuille and Couette flows in the transitional and fully turbulent regime.
Journal of Fluid Mechanics,
Vol. 770,
Issue. ,
p.
424.
Pirozzoli, Sergio
Bernardini, Matteo
and
Orlandi, Paolo
2016.
Passive scalars in turbulent channel flow at high Reynolds number.
Journal of Fluid Mechanics,
Vol. 788,
Issue. ,
p.
614.
Ostilla-Mónico, Rodolfo
Lohse, Detlef
and
Verzicco, Roberto
2016.
Effect of roll number on the statistics of turbulent Taylor-Couette flow.
Physical Review Fluids,
Vol. 1,
Issue. 5,
SASAKI, Eiichi
KAWAHARA, Genta
SEKIMOTO, Atsushi
and
JIMÉNEZ, Javier
2016.
Unstable periodic orbits in plane Couette flow with the Smagorinsky model.
Journal of Physics: Conference Series,
Vol. 708,
Issue. ,
p.
012003.
Ostilla-Mónico, R.
Verzicco, R.
and
Lohse, D.
2016.
Turbulent Taylor–Couette flow with stationary inner cylinder.
Journal of Fluid Mechanics,
Vol. 799,
Issue. ,
Ostilla-Mónico, Rodolfo
Verzicco, Roberto
Grossmann, Siegfried
and
Lohse, Detlef
2016.
The near-wall region of highly turbulent Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 788,
Issue. ,
p.
95.
Wang, Guiquan
Abbas, Micheline
and
Climent, Eric
2017.
Modulation of large-scale structures by neutrally buoyant and inertial finite-size particles in turbulent Couette flow.
Physical Review Fluids,
Vol. 2,
Issue. 8,
Shirokov, I. A.
and
Elizarova, T. G.
2017.
Application of Quasi-Gas Dynamic Equations to Numerical Simulation of Near-Wall Turbulent Flows.
Computational Mathematics and Modeling,
Vol. 28,
Issue. 1,
p.
37.
Ostilla-Mónico, Rodolfo
and
Lee, Alpha A.
2017.
Controlling turbulent drag across electrolytes using electric fields.
Faraday Discussions,
Vol. 199,
Issue. ,
p.
159.
Miao, Sha
Hendrickson, Kelli
and
Liu, Yuming
2017.
Computation of three-dimensional multiphase flow dynamics by Fully-Coupled Immersed Flow (FCIF) solver.
Journal of Computational Physics,
Vol. 350,
Issue. ,
p.
97.
Luchini, Paolo
2017.
Universality of the Turbulent Velocity Profile.
Physical Review Letters,
Vol. 118,
Issue. 22,
Pirozzoli, Sergio
Bernardini, Matteo
Verzicco, Roberto
and
Orlandi, Paolo
2017.
Mixed convection in turbulent channels with unstable stratification.
Journal of Fluid Mechanics,
Vol. 821,
Issue. ,
p.
482.
van Hooijdonk, I. G. S.
Moene, A. F.
Scheffer, M.
Clercx, H. J. H.
and
van de Wiel, B. J. H.
2017.
Early Warning Signals for Regime Transition in the Stable Boundary Layer: A Model Study.
Boundary-Layer Meteorology,
Vol. 162,
Issue. 2,
p.
283.
Luchini, Paolo
2018.
Structure and interpolation of the turbulent velocity profile in parallel flow.
European Journal of Mechanics - B/Fluids,
Vol. 71,
Issue. ,
p.
15.
Kim, Jung Hoon
and
Lee, Jae Hwa
2018.
Direct numerical simulation of a turbulent Couette–Poiseuille flow: Turbulent statistics.
International Journal of Heat and Fluid Flow,
Vol. 72,
Issue. ,
p.
288.
Jiménez, Javier
2018.
Coherent structures in wall-bounded turbulence.
Journal of Fluid Mechanics,
Vol. 842,
Issue. ,
Gandía-Barberá, Sergio
Hoyas, Sergio
Oberlack, Martin
and
Kraheberger, Stefanie
2018.
Letter: The link between the Reynolds shear stress and the large structures of turbulent Couette-Poiseuille flow.
Physics of Fluids,
Vol. 30,
Issue. 4,
Kawata, Takuya
and
Alfredsson, P. Henrik
2018.
Inverse Interscale Transport of the Reynolds Shear Stress in Plane Couette Turbulence.
Physical Review Letters,
Vol. 120,
Issue. 24,
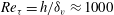 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathit{Re}_{\tau } = h/\delta _v \approx 1000$, where
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathit{Re}_{\tau } = h/\delta _v \approx 1000$, where 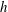 $h$ is the channel half-height and
$h$ is the channel half-height and 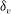 $\delta _v$ is the viscous length scale at which some phenomena representative of the asymptotic Reynolds-number regime manifest themselves. While a logarithmic mean velocity profile is found to provide a reasonable fit of the data, including the skin friction, closer scrutiny shows that deviations from the log law are systematic, and probably increasing at higher Reynolds numbers. The Reynolds stress distribution shows the formation of a secondary outer peak in the streamwise velocity variance, which is associated with significant excess of turbulent production as compared to the local dissipation. This excess is related to the formation of large-scale streaks and rollers, which are responsible for a substantial fraction of the turbulent shear stress in the channel core, and for significant increase of the turbulence intermittency in the near-wall region.
$\delta _v$ is the viscous length scale at which some phenomena representative of the asymptotic Reynolds-number regime manifest themselves. While a logarithmic mean velocity profile is found to provide a reasonable fit of the data, including the skin friction, closer scrutiny shows that deviations from the log law are systematic, and probably increasing at higher Reynolds numbers. The Reynolds stress distribution shows the formation of a secondary outer peak in the streamwise velocity variance, which is associated with significant excess of turbulent production as compared to the local dissipation. This excess is related to the formation of large-scale streaks and rollers, which are responsible for a substantial fraction of the turbulent shear stress in the channel core, and for significant increase of the turbulence intermittency in the near-wall region.