Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-17T17:54:19.829Z Has data issue: false hasContentIssue false
  • English
  • Français

Transient dispersion regimes

Published online by Cambridge University Press:  01 November 2013

Rusty C. Holleman Open the ORCID record for Rusty C. Holleman [Opens in a new window]  and
Mark T. Stacey
Rusty C. Holleman*
Affiliation:
Department of Civil and Environmental Engineering, University of California Berkeley, Berkeley, CA 94720, USA
Mark T. Stacey
Affiliation:
Department of Civil and Environmental Engineering, University of California Berkeley, Berkeley, CA 94720, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Characterizing scalar dispersion is a key concern in a wide variety of applications, including both steady-state and time-dependent studies of wastewater outfalls, salinity distribution in estuaries, and the spreading of pollutants from industrial spills. As the size of a scalar plume grows with respect to the size of the containing water body, the effective dispersion varies, from the well-known ${ \sigma }_{x}^{2} \sim {t}^{3} $ behaviour for a plume enveloped in a region of linear shear, to the ${ \sigma }_{x}^{2} \sim t$ behaviour at the limit of a laterally well-mixed plume. We introduce an additional regime in which the plume extends across the full range of the available shear, but is not significantly affected by the lateral bounds of the water body. Through an analytic treatment we show that this regime exhibits a 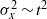${ \sigma }_{x}^{2} \sim {t}^{2} $ behaviour, independent of lateral mixing coefficient. Particle tracking results in an idealized, tidal channel–shoal basin demonstrate this regime as particle clouds straddle the channel–shoal interface. Quantitative analysis of spatial moments as plumes transition between regimes show good correlation between the observed parameters and parameters predicted by the analytic framework.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Geophysical and Geological Flows: Shallow water flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 736 , 10 December 2013 , pp. 130 - 149
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aris, R. 1956 On the dispersion of a solute in a fluid flowing through a tube. Proc. R. Soc. Lond. A 235, 67–77.Google Scholar
Barton, N. G. 1983 On the method of moments for solute dispersion. J. Fluid Mech. 126, 205–218.Google Scholar
Feng, S., Cheng, R. T. & Xi, P. 1986 On tide-induced Lagrangian residual current and residual transport 2. Residual transport with application in South San Francisco Bay, California. Water Resour. Res. 22 (12), 1635–1646.Google Scholar
Fischer, H. B., List, E. J., Koh, R. C. Y., Imberger, J. & Brooks, N. H. 1979 Mixing in Inland and Coastal Waters. Academic.Google Scholar
Fringer, O. B., Gerritsen, M. & Street, R. L. 2006 An unstructured-grid, finite-volume, nonhydrostatic, parallel coastal ocean simulator. Ocean Model. 14, 139–173.Google Scholar
Gross, E. S., MacWilliams, M. L., Holleman, R. C. & Hervier, T. A. 2011 Particle tracking model: testing and applications report. Study. Interagency Ecological Program, Berkeley, CA.Google Scholar
LaBolle, E. M., Quastel, J., Fogg, G. E. & Gravner, J. 2000 Diffusion processes in composite porous media and their numerical integration by random walks: generalized stochastic differential equations with discontinuous coefficients. Water Resour. Res. 36 (3), 651–662.Google Scholar
Li, C. & O’Donnell, J. 2005 The effect of channel length on the residual circulation in tidally dominated channels. J. Phys. Oceanogr. 35, 1826–1840.CrossRefGoogle Scholar
Okubo, A. 1973 Effect of shoreline irregularities on streamwise dispersion in estuaries and other embayments. Netherlands J. Sea Res. 6 (1-2), 213–224.Google Scholar
Ross, O. N. & Sharples, J. 2004 Recipe for 1-D Lagrangian particle tracking models in space-varying diffusivity. Limnol. Oceanogr.: Methods 2, 289–302.Google Scholar
Saffman, P. G. 1962 The effect of wind shear on horizontal spread from an instantaneous ground source. Q. J. R. Meteorol. Soc. 88 (378), 382–393.Google Scholar
Salah-Mars, S. & McCann, M. W. Jr. 2007 Delta risk management strategy: water analysis module. Tech. Mem. URS Corporation, Oakland, CA.Google Scholar
Spydell, M. S. & Feddersen, F. 2012 The effect of a non-zero Lagrangian time scale on bounded shear dispersion. J. Fluid Mech. 691, 69–94.Google Scholar
Stommel, H. & Farmer, H. G. 1952 On the nature of estuarine circulation. Tech. Rep. Woods Hole Oceanographic Institution.Google Scholar
Taylor, G. I. 1953 Dispersion of soluble matter in solvent flowing slowly through a tube. Proc. R. Soc. Lond. A 219, 186–203.Google Scholar
Taylor, G. I. 1954 The Dispersion of matter in turbulent flow through a pipe. Proc. R. Soc. Lond. A 223 (1155), 446–468.Google Scholar
Young, W. R. & Jones, S. 1991 Shear dispersion. Phys. Fluids A 3 (5), 1087–1101.Google Scholar
Young, W. R., Rhines, P. B. & Garrett, C. J. R. 1982 Shear-flow dispersion, internal waves and horizontal mixing in the ocean. J. Phys. Oceanogr. 12, 515–527.Google Scholar