Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-17T09:17:56.497Z Has data issue: false hasContentIssue false
  • English
  • Français

Synchronization of second-mode instability waves for high-enthalpy hypersonic boundary layers

Published online by Cambridge University Press:  25 January 2018

Leonardo C. Salemi Open the ORCID record for Leonardo C. Salemi [Opens in a new window]  and
Hermann F. Fasel
Leonardo C. Salemi
Affiliation:
Department of Aerospace and Mechanical Engineering, The University of Arizona, Tucson AZ 85721, USA
Hermann F. Fasel*
Affiliation:
Department of Aerospace and Mechanical Engineering, The University of Arizona, Tucson AZ 85721, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

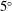The stability of a hypersonic boundary layer for a $5^{\circ }$ half-angle cone at the Caltech T5 high-enthalpy flow conditions was investigated using direct numerical simulations. For the ‘linear’ stability investigations, the boundary layer was perturbed by small axisymmetric disturbances with very small amplitudes, and for the nonlinear regime, three-dimensional pulse disturbances with larger amplitudes were introduced. The surprising result from these investigations was that the 3D wave packet undergoes strong spatial modulations, which we have not observed for other experimental conditions (e.g. the Purdue BAM6QT). This modulation was found to be directly due to the synchronization between second-mode wave components and vorticity/entropy modes. Furthermore, it was found that a synchronization with slow acoustic waves leads to a sudden and strong emission of acoustic waves deep into the free stream, which was observed for both a linear wave train and a 3D nonlinear wave packet. Therefore, it can be concluded that this is a linear mechanism that is not suppressed by nonlinear effects.

JFM classification

Boundary Layers: Boundary layer stability Compressible Flows: Compressible boundary layers Aerodynamics: High-speed flow
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 838 , 10 March 2018 , R2
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bitter, N. E. & Shepherd, J. E. 2015 Stability of highly cooled hypervelocity boundary layers. J. Fluid Mech. 778, 586–620.Google Scholar
Chuvakhov, P. V. & Fedorov, A. V. 2016 Spontaneous radiation of sound by instability of a highly cooled hypersonic boundary layer. J. Fluid Mech. 805, 188–206.CrossRefGoogle Scholar
Gross, A. & Fasel, H. F. 2008 High-order accurate numerical method for complex flows. AIAA J. 46 (1), 204–214.Google Scholar
Hornung, H.1992 Performance data of the new free-piston shock tunnel at GALCIT. AIAA Conf. AIAA Paper 1992-3943.Google Scholar
Jewell, J. S. & Shepherd, J. E.2012 T5 conditions. Tech. Rep. Graduate Aerospace Laboratories, California Institute of Technology, Pasadena, CA.Google Scholar
Lafferty, J. F., Coblish, J. J., Marineau, E., Norris, J. D., Kurits, I., Lewis, D. R., Smith, M. & Marana, M.2015 The hypervelocity wind tunnel no. 9; continued excellence through improvement and modernization. AIAA Conf. AIAA Paper 2015-1340.Google Scholar
Laible, A. C.2011 Numerical investigation of boundary-layer transition for cones at Mach 3.5 and 6.0. PhD thesis, The University of Arizona, Tucson, USA.Google Scholar
Mack, L. M.1984 Boundary layer linear stability theory. Special Course 709. AGARD, Pasadena, California: Jet Propulsion Laboratory. California Institute of Technology.Google Scholar
Parziale, N. J., Shepherd, J. E. & Hornung, H. G. 2013 Differential interferometric measurement of instability in a hypervelocity boundary layer. AIAA J. 51 (3), 750–754.CrossRefGoogle Scholar
Parziale, N. J., Shepherd, J. E. & Hornung, H. G. 2015 Observations of hypervelocity boundary-layer instability. J. Fluid Mech. 781, 87–112.Google Scholar
Salemi, L. C.2016 Numerical investigation of hypersonic conical boundary-layer stability including high-enthalpy and three-dimensional effects. PhD thesis, The University of Arizona, Tucson, USA.Google Scholar
Salemi, L. C., Fasel, H. F., Wernz, S. & Marquart, E.2014 Numerical investigation of wave-packets in a hypersonic high-enthalpy boundary-layer on a $5^{\circ }$ sharp cone. AIAA Conf. AIAA Paper 2014-2775.Google Scholar
Salemi, L. C., Fasel, H. F., Wernz, S. & Marquart, E.2015 Numerical investigation of nonlinear wave-packets in a hypersonic high-enthalpy boundary-layer on a 5 deg sharp cone. AIAA Conf. AIAA Paper 2015-2318.Google Scholar
Schneider, S. P. 2008 Development of hypersonic quiet tunnels. J. Spacecr. Rockets 45 (4), 641–664.Google Scholar
Thumm, A.1991 Numerische Untersuchungen zum laminar–turbulenten Strömungsumschlag in transsonischen Grenzschichtströmungen. PhD thesis, Universität Stuttgart, Stuttgart, Germany.Google Scholar
Tumin, A., Wang, X. & Zhong, X. 2011 Numerical simulation and theoretical analysis of perturbations in hypersonic boundary layers. AIAA J. 49 (3), 463–471.Google Scholar
Wagnild, R. M.2012 High enthalpy effects on two boundary layer disturbances in supersonic and hypersonic flow. PhD thesis, University of Minnesota, Minneapolis, USA.Google Scholar