Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-26T11:45:52.088Z Has data issue: false hasContentIssue false
  • English
  • Français

Stretching of capsules in an elongation flow, a route to constitutive law

Published online by Cambridge University Press:  20 February 2015

C. de Loubens ,
J. Deschamps ,
G. Boedec  and
M. Leonetti
C. de Loubens
Affiliation:
Aix-Marseille Universite, CNRS, Centrale Marseille, IRPHE UMR 7342, 13384 Marseille, France
J. Deschamps
Affiliation:
Aix-Marseille Universite, CNRS, Centrale Marseille, IRPHE UMR 7342, 13384 Marseille, France
G. Boedec
Affiliation:
Aix-Marseille Universite, CNRS, Centrale Marseille, IRPHE UMR 7342, 13384 Marseille, France
M. Leonetti*
Affiliation:
Aix-Marseille Universite, CNRS, Centrale Marseille, IRPHE UMR 7342, 13384 Marseille, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Soft bio-microcapsules are drops bounded by a thin elastic shell made of cross-linked proteins. Their shapes and their dynamics in flow depend on their membrane constitutive law characterized by shearing and area-dilatation resistance. The deformations of such capsules are investigated experimentally in planar elongation flows and compared with numerical simulations for three bidimensional models: Skalak, neo-Hookean and generalized Hooke. An original cross-flow microfluidic set-up allows the visualization of the deformed shape in the two perpendicular main fields of view. Whatever the elongation rate, the three semi-axis lengths of the ellipsoid fitting the experimental shape are measured up to 180 % of stretching of the largest axis. 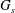The geometrical analysis in the two views is sufficient to determine the constitutive law and the Poisson ratio of the membrane without a preliminary knowledge of the shear elastic modulus $G_{s}$. We conclude that the membrane of human serum albumin capsules obeys the generalized Hooke law with a Poisson ratio of 0.4. The shear elastic modulus is then determined by the combination of numerical and experimental variations of the Taylor parameter with the capillary number.

JFM classification

Biological Fluid Dynamics: Biological Fluid Dynamics Biological Fluid Dynamics: Capsule/cell dynamics Low-Reynolds-number flows: Low-Reynolds-number flows
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 767 , 25 March 2015 , R3
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abreu, D., Levant, M., Steinberg, V. & Seifert, U. 2014 Fluid vesicles in flow. Adv. Colloid Interface Sci. 208, 129–141.Google Scholar
Andry, M. C., Edwards-Levy, F. & Levy, M. C. 1996 Free amino group content of serum albumin microcapsules. III. A study at low pH values. Intl J. Appl. Pharmacol. 128, 197–202.Google Scholar
Bagchi, P. & Kalluri, R. M. 2009 Dynamics of nonspherical capsules in shear flow. Phys. Rev. E 80, 016307.Google Scholar
Barthès-Biesel, D. 2011 Modeling the motion of capsules in flow. Curr. Opin. Colloid Interface Sci. 16, 3–12.Google Scholar
Barthès-Biesel, D., Diaz, A. & Dhenin, E. 2002 Effect of constitutive laws for two-dimensional membranes on flow-induced capsule deformation. J. Fluid Mech. 460, 211–222.Google Scholar
Biben, T., Farutin, A. & Misbah, C. 2011 Three-dimensional vesicles under shear flow: numerical study of dynamics and phase diagram. Phys. Rev. E 83, 031921.Google Scholar
Boedec, G., Leonetti, M. & Jaeger, M. 2011 3D vesicle dynamics simulations with a linearly triangulated surface. J. Comput. Phys. 230, 1020–1034.Google Scholar
Breyiannis, G. & Pozrikidis, C. 2000 Simple shear flow of suspensions of elastic capsules. J. Theor. Comput. Fluid Dyn. 13, 327–347.CrossRefGoogle Scholar
Carin, M., Barthès-Biesel, D., Edwards-Lévy, F., Postel, C. & Andrei, D. C. 2003 Compression of biocompatible liquid-filled HSA-alginate capsules: determination of the membrane mechanical properties. Biotechnol. Bioengng 82, 207–212.Google Scholar
Chang, K. S. & Olbricht, W. 1993 Experimental studies of the deformation of a synthetic capsule in extensional flow. J. Fluid Mech. 250, 587–608.Google Scholar
Dimitrakopoulos, P. 2014 Effects of membrane hardness and scaling analysis for capsules in planar extensional flows. J. Fluid Mech. 745, 487–508.Google Scholar
Doddi, S. K. & Bagchi, P. 2009 Three-dimensional computational modeling of multiple deformable cells flowing in microvessels. Phys. Rev. E 79, 046318.Google Scholar
Dodson, W. R. III & Dimitrakopoulos, P. 2009 Dynamics of strain-hardening and strain-softening capsules in strong planar extensional flows via an interfacial spectral boundary element algorithm for elastic membranes. J. Fluid Mech. 641, 263–296.Google Scholar
Dupont, C., Salsac, A.-V. & Barthès-Biesel, D. 2013 Off-plane motion of a prolate capsule in a shear flow. J. Fluid Mech. 721, 180–198.Google Scholar
Finken, R. & Seifert, U. 2006 Wrinkling of microcapsules in shear flow. J. Phys.: Condens. Matter 18, L185–L191.Google Scholar
Freund, J. B. 2014 Numerical simulation of flowing blood cells. Annu. Rev. Fluid Mech. 46, 67–95.Google Scholar
Gunes, D. Z., Pouzot, M., Ulrich, S. & Mezzenga, R. 2011 Tuneable thickness barriers for composite o/w and w/o capsules, films, and their decoration with particles. Soft Matt. 7, 9206–9215.Google Scholar
Kanstler, V., Segre, E. & Steinberg, V. 2007 Vesicle dynamics in time-dependent elongation flow: wrinkling instability. Phys. Rev. Lett. 99, 178102.Google Scholar
Krüger, T., Kaoui, B. & Harting, J. 2014 Interplay of inertia and deformability on rheological properties of a suspension of capsules. J. Fluid Mech. 751, 725–745.Google Scholar
Kumar, A., Henriquez Rivera, R. G. & Graham, M. D. 2014 Flow-induced segregation in confined multicomponent suspensions: effects of particle size and rigidity. J. Fluid Mech. 738, 423–462.Google Scholar
Lac, E., Barthès-Biesel, D., Pelekasis, N. & Tsamopoulos, J. 2004 Spherical capsules in three-dimensional unbounded Stokes flows: effect of the membrane constitutive law and onset of buckling. J. Fluid Mech. 516, 303–334.Google Scholar
Lefebvre, Y., Leclerc, E., Barthès-Biesel, D., Walter, J. & Edwards-Lévy, F. 2008 Flow of artificial microcapsules in microfluidic channels: a method for determining the elastic properties of the membrane. Phys. Fluids 20, 123102.Google Scholar
Lei, H., Fedosov, D. A., Caswell, B. & Karniadakis, G. Em. 2013 Blood flow in small tubes: quantifying the transition to the non-continuum regime. J. Fluid Mech. 722, 214–239.Google Scholar
Li, X., Vlahovska, P. M. & Karniadakis, G. E. 2013 Continuum- and particle-based modeling of shapes and dynamics of red blood cells in health and disease. Soft Matt. 9, 28–37.Google Scholar
Loop, C. 1987 Smooth Subdivision Surfaces Based on Triangles. University of Utah.Google Scholar
de Loubens, C., Deschamps, J., Georgelin, M., Charrier, A., Edwards-Lévy, F. & Leonetti, M. 2014 Mechanical characterization of cross-linked serum albumin microcapsules. Soft Matt. 10, 4561–4568.Google Scholar
Macosko, C. 1994 Rheology: Principles, Measurements and Applications. Wiley-VCH.Google Scholar
Noguchi, H. & Gompper, G. 2005 Shape transitions of fluid vesicles and red blood cells in capillary flows. Proc. Natl Acad. Sci. USA 102, 14159–14164.Google Scholar
Pozrikidis, C. 2003 Numerical Simulation of Cell Motion in Tube Flow. Chapman & Hall.Google Scholar
Pozrikidis, C. 2005 Modeling and simulation of capsules and biological cells. Ann. Biomed. Engng 33, 165–178.Google Scholar
Queguiner, C. & Barthès-Biesel, D. 1997 Axisymmetric motion of capsules through cylindrical channels. J. Fluid Mech. 348, 349–376.Google Scholar
Ramanujan, S. & Pozrikidis, C. 1998 Deformation of liquid capsules enclosed by elastic membranes in simple shear flow: large deformations and the effect of fluid viscosities. J. Fluid Mech. 361, 117–143.Google Scholar
Risso, F., Colle-Paillot, F. & Zagzoule, M. 2006 Experimental investigation of a bioartificial capsule flowing in a narrow tube. J. Fluid Mech. 547, 149–173.Google Scholar
Skalak, R., Tozeren, A., Zarda, R. P. & Chien, S. 1973 Strain energy function of red blood cell membranes. Biophys. J. 13, 245–264.Google Scholar
Stone, H. 1994 Dynamics of drop deformation and breakup in viscous fluids. Annu. Rev. Fluid Mech. 25, 65–102.Google Scholar
Vlahovska, P. M., Podgorski, T. & Misbah, C. 2009 Vesicles and red blood cells in flow: from individual dynamics to rheology. C. R. Acad. Sci. Paris 10, 775–789.Google Scholar
Vlahovska, P. M., Young, Y.-N., Danker, G. & Misbah, C. 2011 Dynamics of a non-spherical microcapsule with incompressible interface in shear flow. J. Fluid Mech. 678, 221–247.Google Scholar
Walter, A., Rehage, H. & Leonhard, H. 2001 Shear induced deformation of microcapsules: shape oscillations and membrane folding. Colloids Surf. A 123, 183–185.Google Scholar
Walter, J., Salsac, A.-V., Barthès-Biesel, D. & Le Tallec, P. 2010 Coupling of finite element and boundary integral methods for a capsule in a Stokes flow. Intl J. Numer. Meth. Engng 83, 829–850.Google Scholar
Wang, Z., Sui, Y., Selt, P. D. M. & Wang, W. 2013 Three-dimensional dynamics of oblate and prolate capsules in shear flow. Phys. Rev. E 88, 053021.Google Scholar
Winkler, R. G., Fedosov, D. A. & Gompper, G. 2014 Dynamical and rheological properties of soft colloid suspensions. Curr. Opin. Colloid Interface Sci. 19, 594–610.Google Scholar
Yazdani, A. Z. K., Kalluri, R. M. & Bagchi, P. 2011 Tank-treading and tumbling frequencies of capsules and red blood cells. Phys. Rev. E 83, 046305.Google Scholar
Zhao, H. & Shaqfeh, E. S. G. 2011 The dynamics of a vesicle in simple shear flow. J. Fluid Mech. 674, 578–604.Google Scholar
Zhao, H. & Shaqfeh, E. S. G. 2013 The dynamics of a non-dilute vesicle suspension in a simple shear flow. J. Fluid Mech. 725, 709–731.Google Scholar
Zhu, L., Roral, C., Mitra, D. & Brandt, L. 2014 A microfluidic device to sort capsules by deformability: a numerical study. Soft Matt. 10, 7705–7711.Google Scholar
Supplementary material: PDF

de Loubens supplementary material

de Loubens supplementary material 1

Download de Loubens supplementary material(PDF)
PDF 1.2 MB