Hostname: page-component-669899f699-qzcqf Total loading time: 0 Render date: 2025-04-25T01:30:45.906Z Has data issue: false hasContentIssue false
  • English
  • Français

Steady solutions of quasi-geostrophic flows in basins, gulfs and channels on a β-plane

Published online by Cambridge University Press:  10 October 2024

Jeasson F. Gonzalez Open the ORCID record for Jeasson F. Gonzalez [Opens in a new window] ,
L. Zavala Sansón Open the ORCID record for L. Zavala Sansón [Opens in a new window]  and
F. Graef Open the ORCID record for F. Graef [Opens in a new window]
Jeasson F. Gonzalez*
Affiliation:
Secretaría de Educación del Distrito Capital, Bogotá 110931, Colombia
L. Zavala Sansón
Affiliation:
Departamento de Oceanografía Física, CICESE, Ensenada 22860, México
F. Graef
Affiliation:
Departamento de Oceanografía Física, CICESE, Ensenada 22860, México
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

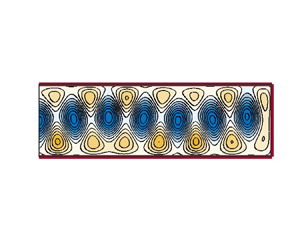
Nonlinear steady solutions of the barotropic quasi-geostrophic equation in basins, gulfs and channels on a $\beta$-plane are presented. The domains are rectangular with arbitrary aspect ratios. The two-dimensional solutions assume a linear relationship between the potential vorticity $q$ and the stream function $\psi$. The sign of the slope in the linear $q\unicode{x2013}\psi$ relationship defines two broad sets of solutions. For a positive slope, the solutions in a closed basin correspond to the inertial gyres derived by Fofonoff in 1954. The negative slope solutions consist of normal modes that can be resonant. For gulfs and channels, the conditions at the open boundaries are almost arbitrary flows entering or leaving the domain. Such conditions allow a great variety of solutions in the interior, characterised mainly by arrays of vortices with alternate signs. Several examples are presented and discussed.

JFM classification

Geophysical and Geological Flows: Quasi-geostrophic flows Vortex Flows: Vortex dynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 997 , 25 October 2024 , A24
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Berman, T., Paldor, N. & Brenner, S. 2000 Simulation of wind-driven circulation in the Gulf of Elat (Aqaba). J. Mar. Sys. 26, 349–365.CrossRefGoogle Scholar
Bower, A.S. & Fratantoni, D.M. 2002 Gulf of Aden eddies and their impact on Red Sea water. Geophys. Res. Let. 29 (21), 1–4.CrossRefGoogle Scholar
Brands, H., Maassen, S.R. & Clercx, H.J.H. 1999 Statistical-mechanical predictions and Navier–Stokes dynamics of two-dimensional flows on a bounded domain. Phys. Rev. E 60 (3), 2864–2874.CrossRefGoogle Scholar
Bretherton, F.B. & Haidvogel, D. 1976 Two-dimensional turbulence over topography. J. Fluid Mech. 78, 129–154.CrossRefGoogle Scholar
Carnevale, G.F. & Fredericksen, J.D. 1987 Nonlinear stability and statistical mechanics of flow over topography. J. Fluid Mech. 175, 157–181.CrossRefGoogle Scholar
Cummins, P.F. 1992 Inertial gyres in decaying and forced geostrophic turbulence. J. Mar. Res. 50 (4), 545–566.CrossRefGoogle Scholar
Fofonoff, N.P. 1954 Steady flow in a frictionless homogeneous ocean. J. Mar. Res. 13 (3), 254–262.Google Scholar
Gonzalez, J.F. & Zavala Sansón, L. 2021 Quasi-geostrophic vortex solutions over isolated topography. J. Fluid Mech. 915, A64.CrossRefGoogle Scholar
González Vera, A.S. & Zavala Sansón, L. 2015 The evolution of a continuously forced shear flow in a closed rectangular domain. Phys. Fluids 27 (3), 034106.CrossRefGoogle Scholar
Griffa, A.C. & Salmon, R. 1989 Wind-driven ocean circulation and equilibrium statistical mechanics J. Mar. Res. 47 (3), 457–492.CrossRefGoogle Scholar
van Heijst, G.J.F., Davies, P.A. & Davis, R.G. 1990 Spin-up in a rectangular container. Phys. Fluids A 2 (2), 150–159.CrossRefGoogle Scholar
Köhl, A. 2007 Generation and stability of a quasi-permanent vortex in the Lofoten Basin. J. Phys. Oceanogr. 37 (11), 2637–2651.CrossRefGoogle Scholar
LaCasce, J.H. 2002 On turbulence and normal modes in a basin. J. Mar. Res. 60, 431–460.CrossRefGoogle Scholar
LaCasce, J.H., Nøst, O.A. & Isachsen, P.E. 2008 Asymmetry of free circulations in closed ocean gyres. J. Phys. Oceanogr. 38 (2), 517–526.CrossRefGoogle Scholar
Lavín, M.F., Castro, R., Beier, E. & Godinez, V.M. 2013 Mesoscale eddies in the southern Gulf of California during summer: characteristics and interaction with the wind stress. J. Geophys. Res. 118, 1367–1381.CrossRefGoogle Scholar
Leith, C.E. 1984 Minimum enstrophy vortices. Phys. Fluids 27 (6), 1388–1395.CrossRefGoogle Scholar
Maassen, S.R., Clercx, H.J.H. & van Heijst, G.J.F. 2003 Self-organization of decaying quasi-two-dimensional turbulence in stratified fluid in rectangular containers. J. Fluid Mech. 495, 19–33.CrossRefGoogle Scholar
Meleshko, V.V. & van Heijst, G.J.F. 1994 On Chaplygin's investigations of two-dimensional vortex structures in an inviscid fluid. J. Fluid Mech. 272, 157–182.CrossRefGoogle Scholar
Merryfield, W.J., Cummins, P.F. & Holloway, G. 2001 Equilibrium statistical mechanics of barotropic flow over finite topography. J. Phys. Oceanogr. 31 (7), 1880–1890.10.1175/1520-0485(2001)031<1880:ESMOBF>2.0.CO;22.0.CO;2>CrossRefGoogle Scholar
Pantoja, D.A., Marinone, S.G. & Filonov, A. 2017 Modeling the effect of a submarine canyon on eddy generation in Banderas Bay, Mexico. J. Coast. Res. 33 (3), 564–572.CrossRefGoogle Scholar
Rhines, P.B. 1975 Waves and turbulence on $\beta$-plane. J. Fluid Mech. 69, 417–443.CrossRefGoogle Scholar
Robert, R. & Sommeria, J. 1991 Statistical equilibrium states for two-dimensional flows. J. Fluid Mech. 229, 291–310.CrossRefGoogle Scholar
Salmon, R., Holloway, G. & Hendershott, M.C. 1976 The equilibrium statistical mechanics of simple quasi-geostrophic models. J. Fluid Mech. 75 (4), 691–703.CrossRefGoogle Scholar
Stern, M.E. 1975 Minimal properties of planetary eddies. J. Mar. Res. 33, 1–13.Google Scholar
Stommel, H. 1948 The westward intensification of wind-driven ocean currents. Trans. Am. Geophys. Union 29 (2), 202–206.Google Scholar
Trieling, R.R., van Heijst, G.J.F. & Kizner, Z. 2010 Laboratory experiments on multipolar vortices in a rotating fluid. Phys. Fluids 22 (9), 094104.CrossRefGoogle Scholar
Vallis, G.K. 2017 Atmospheric and Oceanic Fluid Dynamics. Cambridge University Press.CrossRefGoogle Scholar
Wang, J. & Vallis, G.K. 1994 Emergence of Fofonoff states in inviscid and viscous ocean circulation models. J. Mar. Res. 52, 83–127.CrossRefGoogle Scholar
Wibowo, M.A., Tanjung, A., Rifardi, Elizal, Mubarak, , Yoswaty, D., Susanti, R., Muttaqin, A.S., Fajary, F.R. & Anwika, Y.M. 2022 Understanding the mechanism of currents through the Malacca Strait study case 2020–2022: mean state, seasonal and monthly variation. IOP Conf. 1118, 012069.Google Scholar
Young, W.R. 1987 Selective decay of enstrophy and the excitation of barotropic waves in a channel. J. Atmos. Sci. 44 (19), 2804–2812.2.0.CO;2>CrossRefGoogle Scholar
Zavala Sansón, L. 2003 The two-dimensional character of spin-up in a rectangular container. Phys. Fluids 15 (6), 1811–1814.CrossRefGoogle Scholar
Zavala Sansón, L. 2010 Solutions of barotropic trapped waves around seamounts. J. Fluid Mech. 661, 32–44.CrossRefGoogle Scholar
Zavala Sansón, L. 2022 Effects of mesoscale turbulence on the wind-driven circulation in a closed basin with topography. Geophys. Astrophys. Fluid Dyn. 116 (3), 159–184.CrossRefGoogle Scholar
Zavala Sansón, L., González-Villanueva, A. & Flores, L.M. 2010 Evolution and decay of a rotating flow over random topography. J. Fluid Mech. 642, 159–180.CrossRefGoogle Scholar
Zavala Sansón, L., Sheinbaum, J. & Pérez-Brunius, P. 2018 Single-particle statistics in the southern Gulf of Mexico. Geofís. Intl 57 (2), 139–150.Google Scholar
Zhan, P., Subramanian, A.C., Yao, F. & Hoteit, I. 2014 Eddies in the Red Sea: a statistical and dynamical study. J. Geophys. Res. 119 (6), 3909–3925.CrossRefGoogle Scholar