Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-23T07:24:53.342Z Has data issue: false hasContentIssue false
  • English
  • Français

Statistical characteristics of turbulent mixing in spherical and cylindrical converging Richtmyer–Meshkov instabilities

Published online by Cambridge University Press:  04 October 2021

Xinliang Li ,
Yaowei Fu Open the ORCID record for Yaowei Fu [Opens in a new window] ,
Changping Yu Open the ORCID record for Changping Yu [Opens in a new window]  and
Li Li
Xinliang Li
Affiliation:
LHD, Institute of Mechanics, Chinese Academy of Sciences, Beijing100190, PR China School of Engineering Science, University of Chinese Academy of Sciences, Beijing100049, PR China
Yaowei Fu
Affiliation:
LHD, Institute of Mechanics, Chinese Academy of Sciences, Beijing100190, PR China School of Engineering Science, University of Chinese Academy of Sciences, Beijing100049, PR China
Changping Yu*
Affiliation:
LHD, Institute of Mechanics, Chinese Academy of Sciences, Beijing100190, PR China School of Engineering Science, University of Chinese Academy of Sciences, Beijing100049, PR China
Li Li*
Affiliation:
Institute of Applied Physics and Computational Mathematics, Beijing100094, PR China
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

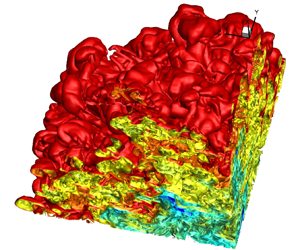
In this paper, the Richtmyer–Meshkov instabilities in spherical and cylindrical converging geometries with a Mach number of approximately 1.5 are investigated by using the high resolution implicit large eddy simulation method, and the influence of the geometric effect on the turbulent mixing is investigated. The heavy fluid is sulphur hexafluoride (SF6), and the light fluid is nitrogen (N2). The shock wave converges from the heavy fluid into the light fluid. The Atwood number is 0.678. The total structured and uniform Cartesian grid node number in the main computational domain is 20483. In addition, to avoid the influence of boundary reflection, a sufficiently long sponge layer with 50 non-uniform coarse grids is added for each non-periodic boundary. Present numerical simulations have high and nonlinear initial perturbation levels, which rapidly lead to turbulent mixing in the mixing layers. Firstly, some physical-variable mean profiles, including mass fraction, Taylor Reynolds number, turbulent kinetic energy, enstrophy and helicity, are provided. Second, the mixing characteristics in the spherical and cylindrical turbulent mixing layers are investigated, such as molecular mixing fraction, efficiency Atwood number, turbulent mass-flux velocity and density self-correlation. Then, Reynolds stress and anisotropy are also investigated. Finally, the radial velocity, velocity divergence and enstrophy in the spherical and cylindrical turbulent mixing layers are studied using the method of conditional statistical analysis. Present numerical results show that the geometric effect has a great influence on the converging Richtmyer–Meshkov instability mixing layers.

JFM classification

Turbulent Flows: Compressible turbulence Compressible Flows: Shock waves Mixing: Turbulent mixing
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 928 , 10 December 2021 , A10
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bell, G.I. 1951 Taylor instability on cylinders and spheres in the small amplitude approximation. Report LA-1321. Los Alamos Scientific Laboratory.Google Scholar
Biamino, L., Jourdan, G., Mariani, C., Houas, L., Vandenboomgaerde, M. & Souffland, D. 2015 On the possibility of studying the converging Richtmyer–Meshkov instability in a conventional shock tube. Exp. Fluids 56, 26.CrossRefGoogle Scholar
Boureima, I., Ramaprabhu, P. & Attal, N. 2018 Properties of the turbulent mixing layer in a spherical implosion. J. Fluids Engng 140, 050905.CrossRefGoogle Scholar
Brouillette, M. 2002 The Richtmyer-Meshkov instability. Annu. Rev. Fluid Mech. 34, 445–468.CrossRefGoogle Scholar
Chapman, P.R. & Jacobs, J.W. 2006 Experiments on the three-dimensional incompressible Richtmyer-Meshkov instability. Phys. Fluids 18, 074101.CrossRefGoogle Scholar
Cook, A.W., Cabot, W. & Miller, P.L. 2004 The mixing transition in Rayleigh–Taylor instability. J. Fluid Mech. 511, 333–362.CrossRefGoogle Scholar
Courtiaud, S., Lecysyn, N., Damamme, G., Poinsot, T. & Selle, L. 2019 Analysis of mixing in high-explosive fireballs using small-scale pressurised spheres. Shock Waves 29, 339–353.CrossRefGoogle Scholar
Design, R. 2008 Theory manual. Available at: chemkin/chemkin-pro.Google Scholar
Ding, J., Li, J., Sun, R., Zhai, Z. & Luo, X. 2019 Convergent Richtmyer-Meshkov instability of a heavy gas layer with perturbed outer interface. J. Fluid Mech. 878, 277–291.CrossRefGoogle Scholar
Ding, J., Si, T., Yang, J., Lu, X., Zhai, Z. & Luo, X. 2017 Measurement of a Richtmyer-Meshkov instability at an air-SF6 interface in a semiannular shock tube. Phys. Rev. Lett. 119, 014501.CrossRefGoogle Scholar
El Rafei, M., Flaig, M., Youngs, D.L. & Thornber, B. 2019 Three-dimensional simulations of turbulent mixing in spherical implosions. Phys. Fluids 31, 114101.CrossRefGoogle Scholar
Epstein, R. 2004 On the bell-plesset effects: the effects of uniform compression and geometrical convergence on the classical Rayleigh-Taylor instability. Phys. Plasmas 11, 5114–5124.CrossRefGoogle Scholar
Fu, Y., Yu, C. & Li, X. 2020 Energy transport characteristics of converging Richtmyer-Meshkov instability. AIP Advances 10, 105302.CrossRefGoogle Scholar
Groom, M. & Thornber, B. 2021 Reynolds number dependence of turbulence induced by the Richtmyer-Meshkov instability using direct numerical simulations. J. Fluid Mech. 908, A31.CrossRefGoogle Scholar
Hahn, M., Drikakis, D., Youngs, D., & Williams, R.J.R. 2011 Richtmyer-Meshkov turbulent mixing arising from an inclined material interface with realistic surface perturbations and reshocked flow. Phys. Fluids 23, 046101.CrossRefGoogle Scholar
Hill, D.L. & Abarzhi, S.I. 2020 On the dynamics of Richtmyer-Meshkov bubbles in unstable three-dimensional interfacial coherent structures with time-dependent acceleration. Phys. Fluids 32, 062107.CrossRefGoogle Scholar
Hosseini, S.H.R. & Takayama, K. 2005 Experimental study of Richtmyer-Meshkov instability induced by cylindrical shock waves. Phys. Fluids 17, 084101.CrossRefGoogle Scholar
Lei, F., Ding, J., Si, T., Zhai, Z. & Luo, X. 2017 Experimental study on a sinusoidal air/SF6 interface accelerated by a cylindrically converging shock. J. Fluid Mech. 826, 819–829.CrossRefGoogle Scholar
Lesieur, M. 1997 Turbulence in Fluids. Kluwer Academic Publishers.CrossRefGoogle Scholar
Li, J., Ding, J., Si, T. & Luo, X. 2020 Convergent Richtmyer-Meshkov instability of light gas layer with perturbed outer surface. J. Fluid Mech. 884, R2.CrossRefGoogle Scholar
Li, X., Leng, Y. & He, Z. 2013 Optimized sixth-order monotonicity-preserving scheme by nonlinear spectral analysis. Intl J. Numer. Meth. Fluids 73, 560–577.CrossRefGoogle Scholar
Liang, Y., Liu, L., Zhai, Z., Si, T. & Wen, C. 2020 a Evolution of shock-accelerated heavy gas layer. J. Fluid Mech. 886, A7.CrossRefGoogle Scholar
Liang, Y., Zhai, Z., Ding, J. & Luo, X. 2019 Richtmyer-Meshkov instability on a quasi-single-mode interface. J. Fluid Mech. 872, 729–751.CrossRefGoogle Scholar
Liang, Y., Zhai, Z., Luo, X. & Wen, C. 2020 b Interfacial instability at a heavy/light interface induced by rarefaction waves. J. Fluid Mech. 885, A42.CrossRefGoogle Scholar
Liu, W., He, X. & Yu, C. 2012 Cylindrical effects on Richtmyer-Meshkov instability for arbitrary Atwood numbers in weakly nonlinear regime. Phys. Plasmas 19, 072108.Google Scholar
Liu, W., Li, X., Yu, C., Fu, Y., Wang, P., Wang, L. & Ye, W. 2018 Theoretical study on finite-thickness effect on harmonics in Richtmyer-Meshkov instability for arbitrary Atwood numbers. Phys. Plasmas 25, 122103.CrossRefGoogle Scholar
Liu, H. & Xiao, Z. 2016 Scale-to-scale energy transfer in mixing flow induced by the Richtmyer-Meshkov instability. Phys. Rev. E 93, 053112.CrossRefGoogle ScholarPubMed
Liu, H., Yu, B., Chen, H., Zhang, B., Xu, H. & Liu, H. 2020 Contribution of viscosity to the circulation deposition in the Richtmyer-Meshkov instability. J. Fluid Mech. 895, A10.CrossRefGoogle Scholar
Liu, W., Yu, C., Ye, W., Wang, L. & He, X. 2014 Nonlinear theory of classical cylindrical Richtmyer-Meshkov instability for arbitrary Atwood numbers. Phys. Plasmas 21, 062119.Google Scholar
Livescu, D. 2020 Turbulence with large thermal and compositional density variations. Annu. Rev. Fluid Mech. 52, 309–341.CrossRefGoogle Scholar
Lombardini, M. 2008 Richtmyer-Meshkov instability in converging geometries. Ph.D. thesis, California Institute of Technology.Google Scholar
Lombardini, M. & Pullin, D.I. 2009 Small-amplitude perturbations in the three-dimensional cylindrical Richtmyer-Meshkov instability. Phys. Fluids 21, 114103.CrossRefGoogle Scholar
Lombardini, M., Pullin, D.I. & Meiron, D.I. 2014 a Turbulent mixing driven by spherical implosions. Part 1. Flow description and mixing-layer growth. J. Fluid Mech. 748, 85–112.CrossRefGoogle Scholar
Lombardini, M., Pullin, D.I. & Meiron, D.I. 2014 b Turbulent mixing driven by spherical implosions. Part 2. Turbulence statistics. J. Fluid Mech. 748, 113–142.CrossRefGoogle Scholar
Luo, X., Ding, J., Wang, M., Zhai, Z. & Si, T. 2015 A semi-annular shock tube for studying cylindrically converging Richtmyer-Meshkov instability. Phys. Fluids 27, 091702.CrossRefGoogle Scholar
Luo, X., Li, M., Ding, J., Zhai, Z. & Si, T. 2019 a Nonlinear behaviour of convergent Richtmyer-Meshkov instability. J. Fluid Mech. 877, 130–141.CrossRefGoogle Scholar
Luo, X., Liang, Y., Si, T. & Zhai, Z. 2019 b Effects of non-periodic portions of interface on Richtmyer-Meshkov instability. J. Fluid Mech. 861, 309–327.CrossRefGoogle Scholar
Luo, X., Zhang, F., Ding, J., Si, T., Yang, J., Zhai, Z. & Wen, C. 2018 Long-term effect of Rayleigh-Taylor stabilization on converging Richtmyer-Meshkov instability. J. Fluid Mech. 849, 231–244.CrossRefGoogle Scholar
Markstein, G.H. 1957 Flow disturbances induced near a slightly wavy contact surface, or flame front, traversed by a shock wave. J. Aerosp. Sci. 24, 238–239.Google Scholar
Meshkov, E.E. 1969 Instability of the interface of two gases accelerated by a shock wave. Sov. Fluid Dyn. 4 (5), 101–104.CrossRefGoogle Scholar
Mikaelian, K.O. 1990 Rayleigh-Taylor and Richtmyer-Meshkov instabilities and mixing in stratified spherical shells. Phys. Rev. A 42, 3400–3420.CrossRefGoogle ScholarPubMed
Mikaelian, K.O. 2005 Rayleigh-Taylor and Richtmyer-Meshkov instabilities and mixing in stratified cylindrical shells. Phys. Fluids 17, 094105.CrossRefGoogle Scholar
Mohaghar, M., Carter, J., Musci, B., Reilly, D., Mcfarland, J. & Ranjan, D. 2017 Evaluation of turbulent mixing transition in a shock-driven variable-density flow. J. Fluid Mech. 831, 779–825.CrossRefGoogle Scholar
Morgan, B.E. & Wickett, M.E. 2015 Three-equation model for the self-similar growth of Rayleigh-Taylor and Richtmyer-Meskov instabilities. Phys. Rev. E 91, 043002.CrossRefGoogle ScholarPubMed
Peng, N., Yang, Y., Wu, J. & Xiao, Z. 2021 Mechanism and modelling of the secondary baroclinic vorticity in the Richtmyer–Meshkov instability. J. Fluid Mech. 911, A56.CrossRefGoogle Scholar
Plesset, M.S. 1954 On the stability of fluid flows with spherical symmetry. J. Appl. Phys. 25, 96–98.CrossRefGoogle Scholar
Rayleigh, Lord 1883 Investigation of the character of the equilibrium of an incompressible heavy fluid of variable density. Proc. Lond. Math. Soc. 14, 170–177.Google Scholar
Reese, D.T., Ames, A.M., Noble, C.D., Oakley, J.G., Rathamer, D.A. & Bonazza, R. 2018 Simultaneous direct measurements of concentration and velocity in the Richtmyer-Meshkov instability. J. Fluid Mech. 849, 541–575.CrossRefGoogle Scholar
Richtmyer, R.D. 1960 Taylor instability in shock acceleration of compressible fluids. Commun. Pure Appl. Maths 13, 297–319.CrossRefGoogle Scholar
Rodriguez, V., Saurel, R., Jourdan, G. & Houas, L. 2017 Impulsive dispersion of a granular layer by a weak blast wave. Shock Waves 27, 187–198.CrossRefGoogle Scholar
Sun, P., Ding, J., Huang, S., Luo, X. & Cheng, W. 2020 a Microscopic Richtmyer-Meshkov instability under strong shock. Phys. Fluids 32, 024109.CrossRefGoogle Scholar
Sun, R., Ding, J., Zhai, Z., Si, T. & Luo, X. 2020 b Convergent RichtmyerMeshkov instability of heavy gas layer with perturbed inner surface. J. Fluid Mech. 902, A3.CrossRefGoogle Scholar
Tang, J., Zhang, F., Luo, X. & Zhai, Z. 2021 Effect of Atwood number on convergent Richtmyer–Meshkov. Acta Mechanica Sin. 37, 434–446.CrossRefGoogle Scholar
Taylor, G.I. 1950 The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. I. Proc. R. Soc. Lond. A 201, 192–196.Google Scholar
Tritschler, V.K., Zubel, M., Hickel, S. & Adams, N.A. 2014 Evolution of length scales and statistics of Richtmyer-Meshkov instability from direct numerical simulations. Phys. Rev. E 90, 063001.CrossRefGoogle ScholarPubMed
Vandenboomgaerde, M., Rouzier, P., Souffland, D., Biamino, L., Jourdan, G., Houas, L. & Mariani, C. 2018 Nonlinear growth of the converging Richtmyer-Meshkov instability in a conventional shock tube. Phys. Rev. Fluids 3, 014001.CrossRefGoogle Scholar
Wilke, C.R. 1950 A viscosity equation for gas mixtures. J. Chem. Phys. 18, 517–519.CrossRefGoogle Scholar
Youngs, D.L. 1991 Three-dimensional numerical simulation of turbulent mixing by Rayleigh-Taylor instability. Phys. Fluids A Fluid Dyn. 3, 1312.CrossRefGoogle Scholar
Youngs, D.L. 1994 Numerical simulation of mixing by Rayleigh-Taylor and Richtmyer-Meshkov instabilities. Laser Part. Beams 12, 725–750.CrossRefGoogle Scholar
Youngs, D.L. & Williams, R.J.R. 2008 Turbulent mixing in spherical implosions. Intl J. Numer. Meth. Fluids 56, 1597–1603.CrossRefGoogle Scholar
Yu, C., Hong, R., Xiao, Z. & Chen, S. 2013 Subgrid-scale eddy viscosity model for helical turbulence. Phys. Fluids 25, 095101.CrossRefGoogle Scholar
Zhai, Z., Li, W., Si, T., Luo, X., Yang, J. & Lu, X. 2017 Refraction of cylindrical converging shock wave at an air/helium gaseous interface. Phys. Fluids 29, 016102.CrossRefGoogle Scholar
Zhai, Z., Zhang, F., Zhou, Z., Ding, J. & Wen, C. 2019 Numerical study on Rayleigh-Taylor effect on cylindrically converging Richtmyer-Meshkov instability. Sci. China-Phys. Mech. Astron. 62, 124712.CrossRefGoogle Scholar
Zhang, Y., Ni, W., Ruan, Y. & Xie, H. 2020 a Quantifying mixing of Rayleigh-Taylor turbulence. Phys. Rev. Fluids 5, 104501.CrossRefGoogle Scholar
Zhang, Y., Ruan, Y., Xie, H. & Tian, B. 2020 b Mixed mass of classical Rayleigh-Taylor mixing at arbitrary density ratios. Phys. Fluids 32, 011702.CrossRefGoogle Scholar
Zhao, Y., Xia, M. & Cao, Y. 2020 A study of bubble growth in the compressible Rayleigh-Taylor and Richtmyer-Meshkov instabilities. AIP Advances 10, 015056.CrossRefGoogle Scholar
Zhou, Y. 2017 a Rayleigh–Taylor and Richtmyer-Meshkov instability induced flow, turbulence, and mixing. I. Phys. Rep. 720–722, 1–136.Google Scholar
Zhou, Y. 2017 b Rayleigh–Taylor and Richtmyer-Meshkov instability induced flow, turbulence, and mixing. II. Phys. Rep. 723–725, 1–160.Google Scholar
Zhou, Y., Cabot, W.H. & Thornber, B. 2016 Asymptotic behavior of the mixed mass in Rayleigh-Taylor and Richtmyer-Meshkov instability induced flows. Phys. Plasmas 23, 052712.CrossRefGoogle Scholar
Zhou, Y., Clark, T.T., Clark, D.S. & Glendinning, S.G. 2019 Turbulent mixing and transition criteria of flows induced by hydrodynamic instabilities. Phys. Plasmas 26, 080901.CrossRefGoogle Scholar
Zhou, Y., Williams, R.J.R., Ramaprabhu, P., et al. 2021 Rayleigh-Taylor and Richtmyer-Meshkov instabilities: a journey through scales. Physica D 423, 132838.CrossRefGoogle Scholar
Zou, L., Al-Marouf, M., Cheng, W., Samtaney, R., Ding, J. & Luo, X. 2019 Richtmyer-Meshkov instability of an unperturbed interface subjected to a diffracted convergent shock. J. Fluid Mech. 879, 448–467.CrossRefGoogle Scholar