Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-17T09:18:28.900Z Has data issue: false hasContentIssue false
  • English
  • Français

Splashing of droplets impacting superhydrophobic substrates

Published online by Cambridge University Press:  07 May 2019

Enrique S. Quintero ,
Guillaume Riboux Open the ORCID record for Guillaume Riboux [Opens in a new window]  and
José Manuel Gordillo Open the ORCID record for José Manuel Gordillo [Opens in a new window]
Enrique S. Quintero
Affiliation:
Área de Mecánica de Fluidos, Departamento de Ingenería Aeroespacial y Mecánica de Fluidos, Universidad de Sevilla, Avenida de los Descubrimientos s/n 41092, Sevilla, Spain
Guillaume Riboux
Affiliation:
Área de Mecánica de Fluidos, Departamento de Ingenería Aeroespacial y Mecánica de Fluidos, Universidad de Sevilla, Avenida de los Descubrimientos s/n 41092, Sevilla, Spain
José Manuel Gordillo*
Affiliation:
Área de Mecánica de Fluidos, Departamento de Ingenería Aeroespacial y Mecánica de Fluidos, Universidad de Sevilla, Avenida de los Descubrimientos s/n 41092, Sevilla, Spain
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A drop of radius $R$ of a liquid of density 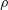$\unicode[STIX]{x1D70C}$, viscosity 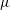$\unicode[STIX]{x1D707}$ and interfacial tension coefficient $\unicode[STIX]{x1D70E}$ impacting a superhydrophobic substrate at a velocity 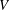$V$ keeps its integrity and spreads over the solid for 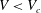$V<V_{c}$ or splashes, disintegrating into tiny droplets violently ejected radially outwards for 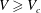$V\geqslant V_{c}$, with 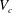$V_{c}$ the critical velocity for splashing. In contrast with the case of drop impact onto a partially wetting substrate, Riboux & Gordillo (Phys. Rev. Lett., vol. 113, 2014, 024507), our experiments reveal that the critical condition for the splashing of water droplets impacting a superhydrophobic substrate at normal atmospheric conditions is characterized by a value of the critical Weber number, $We_{c}=\unicode[STIX]{x1D70C}\,V_{c}^{2}\,R/\unicode[STIX]{x1D70E}\sim O(100)$, which hardly depends on the Ohnesorge number 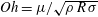$Oh=\unicode[STIX]{x1D707}/\sqrt{\unicode[STIX]{x1D70C}\,R\,\unicode[STIX]{x1D70E}}$ and is noticeably smaller than the corresponding value for the case of partially wetting substrates. Here we present a self-consistent model, in very good agreement with experiments, capable of predicting 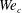$We_{c}$ as well as the full dynamics of the drop expansion and disintegration for 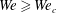$We\geqslant We_{c}$. In particular, our model is able to accurately predict the time evolution of the position of the rim bordering the expanding lamella for 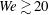$We\gtrsim 20$ as well as the diameters and velocities of the small and fast droplets ejected when 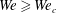$We\geqslant We_{c}$.

JFM classification

Drops and Bubbles: Drops Drops and Bubbles: Breakup/coalescence
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 870 , 10 July 2019 , pp. 175 - 188
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antonini, C., Amirfazli, A. & Marengo, M. 2012 Drop impact and wettability: from hydrophilic to superhydrophobic surfaces. Phys. Fluids 24, 102104.Google Scholar
Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Bergeron, V., Bonn, D., Martin, J. Y. & Vovelle, L. 2000 Controlling droplet deposition with polymer additives. Nature 405, 772–775.Google Scholar
Blossey, R. 2003 Self-cleaning surfaces – virtual realities. Nat. Mater. 2, 301–306.Google Scholar
Clanet, C., Béguin, C., Richard, D. & Quéré, D. 2004 Maximal deformation of an impacting drop. J. Fluid Mech. 517, 199–208.Google Scholar
Culick, F. E. C. 1960 Comments on a ruptured soap film. J. Appl. Phys. 31, 1128.Google Scholar
Eggers, J., Fontelos, M. A., Josserand, C. & Zaleski, S. 2010 Drop dynamics after impact on a solid wall: theory and simulations. Phys. Fluids 22, 062101.Google Scholar
Eggers, J. & Villermaux, E. 2008 Physics of liquid jets. Rep. Progr. Phys. 71 (3), 036601.Google Scholar
Gart, S., Mates, J. E., Megaridis, C. M. & Jung, S. 2015 Droplet impacting a cantilever: a leaf-raindrop system. Phys. Rev. A 3, 044019.Google Scholar
Gilet, T. & Bourouiba, L. 2015 Fluid fragmentation shapes rain-induced foliar disease transmission. J. R. Soc. Interface 12, 20141092.Google Scholar
de Goede, T. C., Laan, N., de Bruin, K. G. & Bonn, D. 2018 Effect of wetting on drop splashing of Newtonian fluids and blood. Langmuir 34 (18), 5163–5168.Google Scholar
Gordillo, J. M., Riboux, G. & Quintero, E. S. 2019 A theory on the spreading of impacting droplets. J. Fluid Mech. 866, 298–315.Google Scholar
Kim, H., Lee, C., Kim, M. H. & Kim, J. 2012 Drop impact characteristics and structure effects of hydrophobic surfaces with micro- and/or nanoscaled structures. Langmuir 28 (30), 11250–11257.Google Scholar
Lejeune, S., Gilet, T. & Bourouiba, L. 2018 Edge effect: liquid sheet and droplets formed by drop impact close to an edge. Phys. Rev. Fluids 3, 083601.Google Scholar
Lv, C., Hao, P., Zhang, X. & He, F. 2016 Drop impact upon superhydrophobic surfaces with regular and hierarchical roughness. Appl. Phys. Lett. 108, 141602.Google Scholar
Mishchenko, L., Hatton, B., Bahadur, V., Taylor, J. A., Krupenkin, T. & Aizenberg, J. 2010 Design of ice-free nanostructured surfaces based on repulsion of impacting water droplets. ACS Nano 4 (12), 7699–7707.Google Scholar
Quéré, D. 2005 Non-sticking drops. Rep. Prog. Phys. 68, 2495–2532.Google Scholar
Riboux, G. & Gordillo, J. M. 2014 Experiments of drops impacting a smooth solid surface: a model of the critical impact speed for drop splashing. Phys. Rev. Lett. 113, 024507.Google Scholar
Riboux, G. & Gordillo, J. M. 2015 The diameters and velocities of the droplets ejected after splashing. J. Fluid Mech. 772, 630–648.Google Scholar
Riboux, G. & Gordillo, J. M. 2016 Maximum drop radius and critical Weber number for splashing in the dynamical Leidenfrost regime. J. Fluid Mech. 803, 516–527.Google Scholar
Riboux, G. & Gordillo, J. M. 2017 Boundary–layer effects in droplet splashing. Phys. Rev. E 96, 013105.Google Scholar
Roisman, I. V. 2009 Inertia dominated drop collisions. II. An analytical solution of the Navier–Stokes equations for a spreading viscous film. Phys. Fluids 21, 052104.Google Scholar
Staat, H. J. J., Tran, T., Geerdink, B., Riboux, G., Sun, C., Gordillo, J. M. & Lohse, D. 2015 Phase diagram for droplet impact on superheated surfaces. J. Fluid Mech. 779, R3.Google Scholar
Taylor, G. I. 1959 The dynamics of thin sheets of fluid. iii. Desintegration of fluid sheets. Proc. R. Soc. Lond. A 253, 1274.Google Scholar
Tran, T., Staat, H. J. J., Prosperetti, A., Sun, C. & Lohse, D. 2012 Drop impact on superheated surfaces. Phys. Rev. Lett. 108, 036101.Google Scholar
Tsai, P., Hendrix, M. H. W., Dijkstra, R. R. M., Shui, L. & Lohse, D. 2011 Microscopic structure influencing macroscopic splash at high Weber number. Soft Matt. 7, 11325–11333.Google Scholar
Wang, Y. & Bourouiba, L. 2018 Unsteady sheet fragmentation: droplet sizes and speeds. J. Fluid Mech. 848, 946–967.Google Scholar
Weisensee, P. B., Tian, J., Miljkovic, N. & King, W. P. 2016 Water droplet impact on elastic superhydrophobic surfaces. Sci. Rep. 6, 30328.Google Scholar
Wildeman, S., Visser, C. W., Sun, C. & Lohse, D. 2016 On the spreading of impacting drops. J. Fluid Mech. 805, 636–655.Google Scholar