Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Abreu, Leandra I.
Cavalieri, André V.G.
Schlatter, Philipp
Vinuesa, Ricardo
and
Henningson, Dan S.
2020.
Resolvent modelling of near-wall coherent structures in turbulent channel flow.
International Journal of Heat and Fluid Flow,
Vol. 85,
Issue. ,
p.
108662.
Dawson, Scott T.M.
and
McKeon, Beverley J.
2020.
Prediction of resolvent mode shapes in supersonic turbulent boundary layers.
International Journal of Heat and Fluid Flow,
Vol. 85,
Issue. ,
p.
108677.
Charkrit, Sita
Shrestha, Pushpa
and
Liu, Chaoqun
2020.
Liutex core line and POD analysis on hairpin vortex formation in natural flow transition.
Journal of Hydrodynamics,
Vol. 32,
Issue. 6,
p.
1109.
Fukami, Kai
Nakamura, Taichi
and
Fukagata, Koji
2020.
Convolutional neural network based hierarchical autoencoder for nonlinear mode decomposition of fluid field data.
Physics of Fluids,
Vol. 32,
Issue. 9,
Ng, Henry C.-H.
Collignon, Emile
Poole, Robert J.
and
Dennis, David J. C.
2021.
Energetic motions in turbulent partially filled pipe flow.
Physics of Fluids,
Vol. 33,
Issue. 2,
Abreu, Leandra I.
Tanarro, Alvaro
Cavalieri, André V.G.
Schlatter, Philipp
Vinuesa, Ricardo
Hanifi, Ardeshir
and
Henningson, Dan S.
2021.
Spanwise-coherent hydrodynamic waves around flat plates and airfoils.
Journal of Fluid Mechanics,
Vol. 927,
Issue. ,
Wang, Hongping
Yang, Zixuan
Wu, Ting
and
Wang, Shizhao
2021.
Coherent structures associated with interscale energy transfer in turbulent channel flows.
Physical Review Fluids,
Vol. 6,
Issue. 10,
Tissot, Gilles
Cavalieri, André V. G.
and
Mémin, Étienne
2021.
Stochastic linear modes in a turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 912,
Issue. ,
Torres, Pablo
Le Clainche, Soledad
and
Vinuesa, Ricardo
2021.
On the Experimental, Numerical and Data-Driven Methods to Study Urban Flows.
Energies,
Vol. 14,
Issue. 5,
p.
1310.
Cavalieri, André V. G.
and
da Silva, André F. C.
2021.
Cross proper orthogonal decomposition.
Physical Review Fluids,
Vol. 6,
Issue. 1,
Salvador, F. J.
Carreres, M.
Quintero, P.
and
González-Montero, L. A.
2021.
Analysis of vortex core generation in pipe flows under different Reynolds number conditions.
Journal of the Brazilian Society of Mechanical Sciences and Engineering,
Vol. 43,
Issue. 6,
Amaral, Filipe R.
Cavalieri, André V.G.
Martini, Eduardo
Jordan, Peter
and
Towne, Aaron
2021.
Resolvent-based estimation of turbulent channel flow using wall measurements.
Journal of Fluid Mechanics,
Vol. 927,
Issue. ,
Kaplan, Oğuzhan
Jordan, Peter
Cavalieri, André V.G.
and
Brès, Guillaume A.
2021.
Nozzle dynamics and wavepackets in turbulent jets.
Journal of Fluid Mechanics,
Vol. 923,
Issue. ,
Fukami, Kai
Murata, Takaaki
Zhang, Kai
and
Fukagata, Koji
2021.
Sparse identification of nonlinear dynamics with low-dimensionalized flow representations.
Journal of Fluid Mechanics,
Vol. 926,
Issue. ,
Cavalieri, André V. G.
2021.
Structure interactions in a reduced-order model for wall-bounded turbulence.
Physical Review Fluids,
Vol. 6,
Issue. 3,
Sharak, M. Niknam
Safavinejad, A.
and
Moayyedi, M.K.
2022.
A fast method based on Dynamic Mode Decomposition for radiative heat transfer in participating media.
Journal of Quantitative Spectroscopy and Radiative Transfer,
Vol. 288,
Issue. ,
p.
108248.
Wang, Ruiqin
He, Xiao
and
Yan, Xin
2022.
Spectral proper orthogonal decomposition analysis of trailing edge cutback film cooling flow.
Physics of Fluids,
Vol. 34,
Issue. 10,
Schlander, Rasmus Korslund
Rigopoulos, Stelios
and
Papadakis, George
2022.
Analysis of wall mass transfer in turbulent pipe flow combining extended proper orthogonal decomposition and Fukagata-Iwamoto-Kasagi identity.
Physical Review Fluids,
Vol. 7,
Issue. 2,
Cavalieri, André V. G.
and
Nogueira, Petrônio A. S.
2022.
Reduced-order Galerkin models of plane Couette flow.
Physical Review Fluids,
Vol. 7,
Issue. 10,
Cavalieri, André V.G.
Rempel, Erico L.
and
Nogueira, Petrônio A.S.
2022.
Transition to chaos in a reduced-order model of a shear layer.
Journal of Fluid Mechanics,
Vol. 932,
Issue. ,
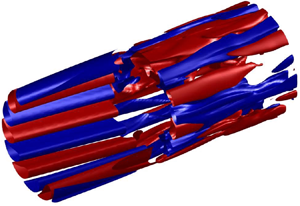
 $Re_{\tau } = 180$ and
$Re_{\tau } = 180$ and  $550$. The database was analysed using spectral proper orthogonal decomposition (SPOD) to identify energetically dominant coherent structures, most of which turn out to be streaks and quasi-streamwise vortices. To understand how such structures can be modelled, the linear flow responses to harmonic forcing were computed using the singular value decomposition of the resolvent operator, using the mean field as a base flow. The SPOD and resolvent analysis were calculated for several combinations of frequencies and wavenumbers, allowing the mapping out of similarities between SPOD modes and optimal responses for a wide range of relevant scales in turbulent pipe flows. In order to explore physical reasons behind the agreement between both methods, an indicator of lift-up mechanism in the resolvent analysis was introduced, activated when optimal forcing is dominated by the wall-normal and azimuthal components, and associated response corresponds to streaks of streamwise velocity. Good agreement between leading SPOD and resolvent modes is observed in a large region of parameter space. In this region, a significant gain separation is found in resolvent analysis, which may be attributed to the strong amplification associated with the lift-up mechanism, here understood as nonlinear forcing terms leading to the appearance of streamwise vortices, which in turn form high-amplitude streaks. For both Reynolds numbers, the observed concordances were generally for structures with large energy in the buffer layer. The results highlight resolvent analysis as a pertinent reduced-order model for coherent structures in wall-bounded turbulence, particularly for streamwise elongated structures corresponding to near-wall streamwise vortices and streaks.
$550$. The database was analysed using spectral proper orthogonal decomposition (SPOD) to identify energetically dominant coherent structures, most of which turn out to be streaks and quasi-streamwise vortices. To understand how such structures can be modelled, the linear flow responses to harmonic forcing were computed using the singular value decomposition of the resolvent operator, using the mean field as a base flow. The SPOD and resolvent analysis were calculated for several combinations of frequencies and wavenumbers, allowing the mapping out of similarities between SPOD modes and optimal responses for a wide range of relevant scales in turbulent pipe flows. In order to explore physical reasons behind the agreement between both methods, an indicator of lift-up mechanism in the resolvent analysis was introduced, activated when optimal forcing is dominated by the wall-normal and azimuthal components, and associated response corresponds to streaks of streamwise velocity. Good agreement between leading SPOD and resolvent modes is observed in a large region of parameter space. In this region, a significant gain separation is found in resolvent analysis, which may be attributed to the strong amplification associated with the lift-up mechanism, here understood as nonlinear forcing terms leading to the appearance of streamwise vortices, which in turn form high-amplitude streaks. For both Reynolds numbers, the observed concordances were generally for structures with large energy in the buffer layer. The results highlight resolvent analysis as a pertinent reduced-order model for coherent structures in wall-bounded turbulence, particularly for streamwise elongated structures corresponding to near-wall streamwise vortices and streaks.