Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-17T13:19:56.108Z Has data issue: false hasContentIssue false
  • English
  • Français

Spanwise turbulence structure over permeable walls

Published online by Cambridge University Press:  01 June 2017

Kazuhiko Suga Open the ORCID record for Kazuhiko Suga [Opens in a new window] ,
Yuka Nakagawa  and
Masayuki Kaneda
Kazuhiko Suga*
Affiliation:
Department of Mechanical Engineering, Osaka Prefecture University, Osaka 599-8531, Japan
Yuka Nakagawa
Affiliation:
Department of Mechanical Engineering, Osaka Prefecture University, Osaka 599-8531, Japan
Masayuki Kaneda
Affiliation:
Department of Mechanical Engineering, Osaka Prefecture University, Osaka 599-8531, Japan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Spanwise flow field measurements are carried out for turbulent flows in channels with permeable bottom walls by particle image velocimetry (PIV) to understand the effects of the wall permeability on turbulence structure near porous walls. The porous media used are three kinds of foamed ceramics which have the same porosities (0.8) but different permeabilities. The turbulent flow fields in spanwise planes are discussed using instantaneous and statistical measurement data. 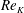At a small permeability Reynolds number ($Re_{K}$), low-speed and high-speed streaks, which are similar to those of solid-wall turbulence, are observed near the walls while at a large 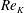$Re_{K}$ the observed structure is very different from that of the solid-wall turbulence. It is found that the obtained spanwise scales of the structure can be reasonably correlated with the wall normal distance plus the zero-plane displacement which is estimated from the mean velocity profile. With the distribution profiles of the spanwise streak spacing and integral length scales, the transitional change of the turbulence structure over permeable walls is discussed.

JFM classification

Turbulent Flows: Turbulent boundary layers Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 822 , 10 July 2017 , pp. 186 - 201
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adrian, R. J., Meinhart, C. D. & Tomkins, C. D. 2000 Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 422, 1–54.CrossRefGoogle Scholar
Beavers, G. S. & Joseph, D. D. 1967 Boundary conditions at a naturally permeable wall. J. Fluid Mech. 30, 197–207.CrossRefGoogle Scholar
Best, A. C.1935 Transfer of heat and momentum in lowest layers of the atmosphere. Tech. Rep. 65. Geophys. Mem., Met. Off. Lond.Google Scholar
Breugem, W. P., Boersma, B. J. & Uittenbogaard, R. E. 2006 The influence of wall permeability on turbulent channel flow. J. Fluid Mech. 562, 35–72.CrossRefGoogle Scholar
Chandesris, M., D’Hueppe, A., Mathieu, B., Jamet, D. & Goyeau, B. 2013 Direct numerical simulation of turbulent heat transfer in a fluid-porous domain. Phys. Fluids 25 (12), 125110.CrossRefGoogle Scholar
Ho, R. T. & Gelhar, L. W. 1973 Turbulent flow with wavy permeable boundaries. J. Fluid Mech. 58, 403–414.CrossRefGoogle Scholar
Host-Madsen, A. & McCluskey, D. R. 1994 On the accuracy and reliability of PIV measurements. In 7th International Symposium Application of Laser Techniques to Fluid Mechanics, Lisboa, Portugal, pp. 214–226.Google Scholar
Iritani, Y., Kasagi, N. & Hirata, M. 1985 Heat transfer mechanism and associated turbulence structure in the near-wall region of a turbulent boundary layer. Turb. Shear Flows 4, 2223–2234.Google Scholar
Jiménez, J., Uhlmann, M., Pinelli, A. & Kawahara, G. 2001 Turbulent shear flow over active and passive porous surfaces. J. Fluid Mech. 442, 89–117.CrossRefGoogle Scholar
Kim, J., Moin, P. & Moser, R. 1987 Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 177, 133–166.CrossRefGoogle Scholar
Kong, F. Y. & Schetz, J. A.1982 Turbulent boundary layer over porous surfaces with different surface geometries. AIAA Paper 82-0030.CrossRefGoogle Scholar
Kuwata, Y. & Suga, K. 2016a Lattice Boltzmann direct numerical simulation of interface turbulence over porous and rough walls. Intl J. Heat Fluid Flow 61, 145–157.CrossRefGoogle Scholar
Kuwata, Y. & Suga, K. 2016b Transport mechanism of interface turbulence over porous and rough walls. Flow Turbul. Combust. 97, 1071–1093.CrossRefGoogle Scholar
Lovera, F. & Kennedy, J. F. 1969 Friction factors for flat bed flows in sand channels. J. Hydraul. Div. ASCE 95, 1227–1234.CrossRefGoogle Scholar
Manes, C., Poggi, D. & Ridol, L. 2011 Turbulent boundary layers over permeable walls: scaling and near-wall structure. J. Fluid Mech. 687, 141–170.CrossRefGoogle Scholar
Nepf, H. & Ghisalberti, M. 2008 Flow and transport in channels with submerged vegetation. Acta Geophys. 56, 753–777.CrossRefGoogle Scholar
Nikora, V., Koll, K., McLean, S., Dittrich, A. & Aberle, J. 2002 Zero-plane displacement for rough-bed open-channel flows. In International Conference on Fluvial Hydraulics River Flow 2002, Louvain-la-Neuve, Belgium, pp. 83–92. Swets & Zeitlinger, Lisse, The Netherland.Google Scholar
Nikuradse, J.1933 Strömungsgesetze in rauhen Rohren. VDI-Forschungsheft 361.Google Scholar
Pokrajac, D. & Manes, C. 2009 Velocity measurements of a free-surface turbulent flow penetrating a porous medium composed of uniform-size spheres. Transp. Porous Med. 78, 367–383.CrossRefGoogle Scholar
Prasad, A. K., Adrian, R. J., Landreth, C. C. & Offutt, P. W. 1992 Effect of resolution on the speed and accuracy of particle image velocimetry interrogation. Exp. Fluids 13, 105–116.CrossRefGoogle Scholar
Raupach, M. R., Antonia, R. A. & Rajagopalan, S. 1991 Rough-wall turbulent boundary layers. Appl. Mech. Rev. 44, 1–25.CrossRefGoogle Scholar
Rosti, M. E., Cortelezzi, L. & Quadrio, M. 2015 Direct numerical simulation of turbulent channel flow over porous walls. J. Fluid Mech. 784, 396–442.CrossRefGoogle Scholar
Ruff, J. F. & Gelhar, L. W. 1972 Turbulent shear flow in porous boundary. J. Engng Mech. ASCE 98, 975–991.Google Scholar
Smith, C. R. & Metzler, S. P. 1983 The characteristics of low-speed streaks in the near-wall region of a turbulent boundary layer. J. Fluid Mech. 129, 27–54.CrossRefGoogle Scholar
Suga, K. 2016 Understanding and modelling turbulence over and inside porous media. Flow Turb. Combust. 96, 717–756.CrossRefGoogle Scholar
Suga, K., Matsumura, Y., Ashitaka, Y., Tominaga, S. & Kaneda, M. 2010 Effects of wall permeability on turbulence. Intl J. Heat Fluid Flow 31, 974–984.CrossRefGoogle Scholar
Suga, K., Mori, M. & Kaneda, M. 2011 Vortex structure of turbulence over permeable walls. Intl J. Heat Fluid Flow 32, 586–595.CrossRefGoogle Scholar
Tomkins, C. D. & Adrian, R. J. 2003 Spanwise structure and scale growth in turbulent boundary layers. J. Fluid Mech. 490, 37–74.CrossRefGoogle Scholar
Willert, C. E. & Gharib, M. 1991 Digital particle image velocimetry. Exp. Fluids 10, 181–193.CrossRefGoogle Scholar
Zagni, A. F. E. & Smith, K. V. H. 1976 Channel flow over permeable beds of graded spheres. J. Hydraul. Div. 102, 207–222.CrossRefGoogle Scholar
Zippe, H. J. & Graf, W. H. 1983 Turbulent boundary-layer flow over permeable and non-permeable rough surfaces. J. Hydraul. Res. 21, 51–65.CrossRefGoogle Scholar