Published online by Cambridge University Press: 17 February 2014
We numerically characterize the temporal regimes for solutal convection from almost first contact to high dissolved solute concentration in a two-dimensional ideal porous layer for Rayleigh numbers 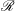 $\mathcal{R}$ between
$\mathcal{R}$ between 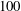 $100$ and
$100$ and 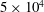 $5\times 10^4$. The lower boundary is impenetrable. The upper boundary is saturated with dissolved solute and either impermeable or partially permeable to fluid flow. In the impermeable case, initially there is pure diffusion of solute away from the upper boundary, followed by the birth and growth of convective fingers. Eventually fingers interact and merge, generating complex downwelling plumes. Once the inter-plume spacing is sufficient, small protoplumes reinitiate on the boundary layer and are swept into the primary plumes. The flow is now in a universal regime characterized by a constant (dimensionless) dissolution flux
$5\times 10^4$. The lower boundary is impenetrable. The upper boundary is saturated with dissolved solute and either impermeable or partially permeable to fluid flow. In the impermeable case, initially there is pure diffusion of solute away from the upper boundary, followed by the birth and growth of convective fingers. Eventually fingers interact and merge, generating complex downwelling plumes. Once the inter-plume spacing is sufficient, small protoplumes reinitiate on the boundary layer and are swept into the primary plumes. The flow is now in a universal regime characterized by a constant (dimensionless) dissolution flux 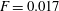 $F=0.017$ (the rate at which solute dissolves from the upper boundary). The horizontally averaged concentration profile stretches as a simple self-similar wedge beneath a diffusive horizontal boundary layer. Throughout, the plume width broadens proportionally to
$F=0.017$ (the rate at which solute dissolves from the upper boundary). The horizontally averaged concentration profile stretches as a simple self-similar wedge beneath a diffusive horizontal boundary layer. Throughout, the plume width broadens proportionally to 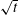 $\sqrt{t}$, where
$\sqrt{t}$, where  $t$ is (dimensionless) time. The above behaviour is parameter independent; the Rayleigh number only controls when transition occurs to a final
$t$ is (dimensionless) time. The above behaviour is parameter independent; the Rayleigh number only controls when transition occurs to a final 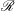 $\mathcal{R}$-dependent shut-down regime. For the constant-flux and shut-down regimes, we rigourously derive upscaled equations connecting the horizontally averaged concentration, vertical advective flux and plume widths. These are partially complete; a universal expression for the plume width remains elusive. We complement these governing equations with phenomenological boundary conditions based on a marginally stable diffusive boundary layer at the top and zero advective flux at the bottom. Making appropriate approximations in each regime, we find good agreement between predictions from this model and simulated results for both solutal and thermal convection. In the partially permeable upper boundary case, fluid from the convecting layer can penetrate an overlying separate-phase-solute bearing layer where it immediately saturates. The regime diagram remains almost the same as for the impermeable case, but the dissolution flux is significantly augmented. Our work is motivated by dissolution of carbon dioxide relevant to geological storage, and we conclude with a simple flux parameterization for inclusion in gravity current models and suggest that the upscaled equations could lay the foundation for accurate inclusion of dissolution in reservoir simulators.
$\mathcal{R}$-dependent shut-down regime. For the constant-flux and shut-down regimes, we rigourously derive upscaled equations connecting the horizontally averaged concentration, vertical advective flux and plume widths. These are partially complete; a universal expression for the plume width remains elusive. We complement these governing equations with phenomenological boundary conditions based on a marginally stable diffusive boundary layer at the top and zero advective flux at the bottom. Making appropriate approximations in each regime, we find good agreement between predictions from this model and simulated results for both solutal and thermal convection. In the partially permeable upper boundary case, fluid from the convecting layer can penetrate an overlying separate-phase-solute bearing layer where it immediately saturates. The regime diagram remains almost the same as for the impermeable case, but the dissolution flux is significantly augmented. Our work is motivated by dissolution of carbon dioxide relevant to geological storage, and we conclude with a simple flux parameterization for inclusion in gravity current models and suggest that the upscaled equations could lay the foundation for accurate inclusion of dissolution in reservoir simulators.
Evolution of the concentration profile at early times forR=50000 and epsilon=0.01 in a small region of the domain near the upper boundary (the lower boundary does not yet play a role in the dynamics). The diffusive, linear-growth, flux-growth and merging regimes are seen.
Evolution of the concentration profile at early times forR=50000 and epsilon=0.01 in a small region of the domain near the upper boundary (the lower boundary does not yet play a role in the dynamics). The diffusive, linear-growth, flux-growth and merging regimes are seen.