Article contents
Short- to long-wave resonance and soliton formation in boundary-layer interaction with a liquid film
Published online by Cambridge University Press: 12 July 2010
Abstract
Dynamic interaction between a boundary layer of air and a liquid film is investigated in this paper. The low air-to-film-viscosity ratio is considered in which case the boundary layer is quasi-steady on the time scale within which interfacial waves develop. The base flow consists of a boundary layer that drags a film of constant shear. Linear analysis, in the context of triple-deck theory, predicts the formation of a wavepacket of capillary waves that advances and spreads with time. The Froude number of de-/anti-icing fluids or water interacting with air falls well within the supercritical regime, i.e. Fr > FrCr. Numerical simulations of such flow systems were performed in the context of triple-deck theory, and they do not exhibit wave saturation or formation of uniform wavetrains. The long-term interaction is mainly dependent on film inertia as this is characterized by parameter 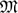 = (μ/μf)2(ρf/ρ), which involves film and air viscosity and density ratios, and the dimensionless film thickness, H0, and shear, λ, provided by the base flow. Weakly nonlinear analysis taking into consideration mean drift, i.e. generation of long waves, due to self-interaction of the linear wave to O(ϵ2) in amplitude of the initial disturbance, reveals resonance between the wavepacket predicted by linear theory and long waves when the group velocity of the former happens to coincide with the phase velocity, H0λ, of long interfacial waves. Numerical simulations with anti-icing fluids and water verify this pattern. In both cases, long waves eventually dominate the dynamics and, as they are modulated with time, they lead to soliton-type structures. Anti-icing fluids eventually exhibit oscillatory spikes whose mean value never exceeds 2H0, roughly. Water films exhibit a single spike that keeps growing, thus generating a large separation bubble.
= (μ/μf)2(ρf/ρ), which involves film and air viscosity and density ratios, and the dimensionless film thickness, H0, and shear, λ, provided by the base flow. Weakly nonlinear analysis taking into consideration mean drift, i.e. generation of long waves, due to self-interaction of the linear wave to O(ϵ2) in amplitude of the initial disturbance, reveals resonance between the wavepacket predicted by linear theory and long waves when the group velocity of the former happens to coincide with the phase velocity, H0λ, of long interfacial waves. Numerical simulations with anti-icing fluids and water verify this pattern. In both cases, long waves eventually dominate the dynamics and, as they are modulated with time, they lead to soliton-type structures. Anti-icing fluids eventually exhibit oscillatory spikes whose mean value never exceeds 2H0, roughly. Water films exhibit a single spike that keeps growing, thus generating a large separation bubble.
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2010
References
REFERENCES
- 3
- Cited by


