Article contents
Self-induced flow over a cylinder in a stratified fluid
Published online by Cambridge University Press: 05 June 2023
Abstract
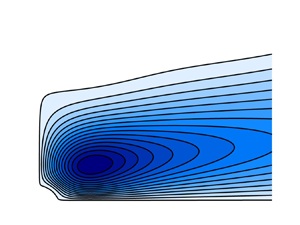
In this paper we study the self-induced low-Reynolds-number flow generated by a cylinder immersed in a stratified fluid. In the low Péclet limit, where the Péclet number is the ratio of the radius of the cylinder and the Phillips length scale, the flow is captured by a set of linear equations obtained by linearising the governing equations with respect to the prescribed far field conditions. We specifically focus on the low Péclet regime and develop a Green's function approach to solve the linearised equations governing the flow over the cylinder. We cross check our analytical solution against numerical solution of the nonlinear equations to obtain the range of the Péclet numbers for which the linear solution is valid. We then take advantage of the analytical solution to find explicit far-field decay rates of the flow. Our detailed analysis points out that the streamfunction and the velocity field decays algebraically in the far field. Intriguingly, this algebraic decay of the flow is much slower when compared with the exponential decay of the flow generated by a slow moving cylinder in the homogeneous Stokes regime, in the absence of stratification. Consequently, the flow generated by a cylinder in the stratified Stokes regime will have a larger domain of influence when compared with the flow generated by a cylinder in the homogeneous Stokes regime.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 2
- Cited by



