Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zhang, Xinxian
Watanabe, Tomoaki
and
Nagata, Koji
2018.
Turbulent/nonturbulent interfaces in high-resolution direct numerical simulation of temporally evolving compressible turbulent boundary layers.
Physical Review Fluids,
Vol. 3,
Issue. 9,
Nagata, R.
Watanabe, T.
and
Nagata, K.
2018.
Turbulent/non-turbulent interfaces in temporally evolving compressible planar jets.
Physics of Fluids,
Vol. 30,
Issue. 10,
Mistry, Dhiren
Philip, Jimmy
and
Dawson, James R.
2019.
Kinematics of local entrainment and detrainment in a turbulent jet.
Journal of Fluid Mechanics,
Vol. 871,
Issue. ,
p.
896.
Buxton, O. R. H.
Breda, M.
and
Dhall, K.
2019.
Importance of small-scale anisotropy in the turbulent/nonturbulent interface region of turbulent free shear flows.
Physical Review Fluids,
Vol. 4,
Issue. 3,
Zhang, Xinxian
Watanabe, Tomoaki
and
Nagata, Koji
2019.
Passive scalar mixing near turbulent/non-turbulent interface in compressible turbulent boundary layers.
Physica Scripta,
Vol. 94,
Issue. 4,
p.
044002.
Neamtu-Halic, Marius M.
Krug, Dominik
Haller, George
and
Holzner, Markus
2019.
Lagrangian coherent structures and entrainment near the turbulent/non-turbulent interface of a gravity current.
Journal of Fluid Mechanics,
Vol. 877,
Issue. ,
p.
824.
Elsinga, G. E.
and
da Silva, C. B.
2019.
How the turbulent/non-turbulent interface is different from internal turbulence.
Journal of Fluid Mechanics,
Vol. 866,
Issue. ,
p.
216.
Watanabe, Tomoaki
da Silva, Carlos B.
and
Nagata, Koji
2019.
Non-dimensional energy dissipation rate near the turbulent/non-turbulent interfacial layer in free shear flows and shear free turbulence.
Journal of Fluid Mechanics,
Vol. 875,
Issue. ,
p.
321.
Yu, Jia-Long
and
Lu, Xi-Yun
2019.
Topological evolution near the turbulent/non-turbulent interface in turbulent mixing layer.
Journal of Turbulence,
Vol. 20,
Issue. 5,
p.
300.
Breda, M.
and
Buxton, O. R. H.
2019.
Behaviour of small-scale turbulence in the turbulent/non-turbulent interface region of developing turbulent jets.
Journal of Fluid Mechanics,
Vol. 879,
Issue. ,
p.
187.
Tanaka, Shusei
Watanabe, Tomoaki
and
Nagata, Koji
2019.
Multi-particle model of coarse-grained scalar dissipation rate with volumetric tensor in turbulence.
Journal of Computational Physics,
Vol. 389,
Issue. ,
p.
128.
Wu, Zhao
Zaki, Tamer A.
and
Meneveau, Charles
2020.
High-Reynolds-number fractal signature of nascent turbulence during transition.
Proceedings of the National Academy of Sciences,
Vol. 117,
Issue. 7,
p.
3461.
Watanabe, T.
da Silva, C. B.
and
Nagata, K.
2020.
Scale-by-scale kinetic energy budget near the turbulent/nonturbulent interface.
Physical Review Fluids,
Vol. 5,
Issue. 12,
Neamtu-Halic, Marius M.
Krug, Dominik
Mollicone, Jean-Paul
van Reeuwijk, Maarten
Haller, George
and
Holzner, Markus
2020.
Connecting the time evolution of the turbulence interface to coherent structures.
Journal of Fluid Mechanics,
Vol. 898,
Issue. ,
Kohan, Khashayar F.
and
Gaskin, Susan
2020.
The effect of the geometric features of the turbulent/non-turbulent interface on the entrainment of a passive scalar into a jet.
Physics of Fluids,
Vol. 32,
Issue. 9,
Balamurugan, G.
Rodda, A.
Philip, J.
and
Mandal, A. C.
2020.
Characteristics of the turbulent non-turbulent interface in a spatially evolving turbulent mixing layer.
Journal of Fluid Mechanics,
Vol. 894,
Issue. ,
Freedland, Graham A.
Eliason, G.
Solovitz, S.A.
and
Cal, R.B.
2020.
The role of turbulent inflow on the development of a round jet in cross-flow..
International Journal of Heat and Fluid Flow,
Vol. 84,
Issue. ,
p.
108592.
Fiscaletti, D.
Buxton, O. R. H.
and
Attili, A.
2021.
Internal layers in turbulent free-shear flows.
Physical Review Fluids,
Vol. 6,
Issue. 3,
Denker, Dominik
Attili, Antonio
Gauding, Michael
Niemietz, Kai
Bode, Mathis
and
Pitsch, Heinz
2021.
A new modeling approach for mixture fraction statistics based on dissipation elements.
Proceedings of the Combustion Institute,
Vol. 38,
Issue. 2,
p.
2681.
Long, Yanguang
Wu, Di
and
Wang, Jinjun
2021.
A novel and robust method for the turbulent/non-turbulent interface detection.
Experiments in Fluids,
Vol. 62,
Issue. 7,
 $142\leqslant Re_{\unicode[STIX]{x1D706}}\leqslant 400$. For
$142\leqslant Re_{\unicode[STIX]{x1D706}}\leqslant 400$. For 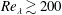 $Re_{\unicode[STIX]{x1D706}}\gtrsim 200$ the thickness of the TNTI (
$Re_{\unicode[STIX]{x1D706}}\gtrsim 200$ the thickness of the TNTI (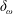 $\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D714}}$), like that of its two sublayers – the viscous superlayer (VSL,
$\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D714}}$), like that of its two sublayers – the viscous superlayer (VSL, 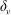 $\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D708}}$) and the turbulent sublayer (TSL,
$\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D708}}$) and the turbulent sublayer (TSL, 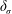 $\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70E}}$) – all scale with the Kolmogorov micro-scale
$\unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70E}}$) – all scale with the Kolmogorov micro-scale 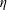 $\unicode[STIX]{x1D702}$, while the particular scaling constant depends on the sublayer. Specifically, for
$\unicode[STIX]{x1D702}$, while the particular scaling constant depends on the sublayer. Specifically, for 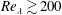 $Re_{\unicode[STIX]{x1D706}}\gtrsim 200$ while the VSL is always of the order of
$Re_{\unicode[STIX]{x1D706}}\gtrsim 200$ while the VSL is always of the order of 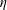 $\unicode[STIX]{x1D702}$, with
$\unicode[STIX]{x1D702}$, with 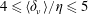 $4\leqslant \langle \unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D708}}\rangle /\unicode[STIX]{x1D702}\leqslant 5$, the TSL and the TNTI are typically equal to
$4\leqslant \langle \unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D708}}\rangle /\unicode[STIX]{x1D702}\leqslant 5$, the TSL and the TNTI are typically equal to 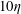 $10\unicode[STIX]{x1D702}$, with
$10\unicode[STIX]{x1D702}$, with  $10.4\leqslant \langle \unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70E}}\rangle /\unicode[STIX]{x1D702}\leqslant 12.5$, and
$10.4\leqslant \langle \unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D70E}}\rangle /\unicode[STIX]{x1D702}\leqslant 12.5$, and 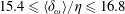 $15.4\leqslant \langle \unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D714}}\rangle /\unicode[STIX]{x1D702}\leqslant 16.8$, respectively.
$15.4\leqslant \langle \unicode[STIX]{x1D6FF}_{\unicode[STIX]{x1D714}}\rangle /\unicode[STIX]{x1D702}\leqslant 16.8$, respectively.

