Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-27T05:42:57.508Z Has data issue: false hasContentIssue false
  • English
  • Français

Regimes of tonal noise on an airfoil at moderate Reynolds number

Published online by Cambridge University Press:  04 September 2015

S. Pröbsting ,
F. Scarano  and
S. C. Morris
S. Pröbsting*
Affiliation:
Department of Aerodynamics, Wind Energy, Flight Performance, and Propulsion, Delft University of Technology, 2629 HS Delft, The Netherlands
F. Scarano
Affiliation:
Department of Aerodynamics, Wind Energy, Flight Performance, and Propulsion, Delft University of Technology, 2629 HS Delft, The Netherlands
S. C. Morris
Affiliation:
Department of Aerospace and Mechanical Engineering, University of Notre Dame, Notre Dame, IN 46556, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Tonal noise generated by airfoils at low to moderate Reynolds number is relevant for applications in, for example, small-scale wind turbines, fans and unmanned aerial vehicles. Coherent and convected vortical structures scattering at the trailing edge from the pressure or suction sides of the airfoil have been identified to be responsible for such tonal noise generation. Controversy remains on the respective significance of pressure- and suction-side events, along with their interaction for tonal noise generation. 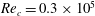The present study surveys the regimes of tonal noise generation for low to moderate chord-based Reynolds number between $\mathit{Re}_{c}=0.3\times 10^{5}$ and $2.3\times 10^{5}$ and effective angle of attack between 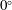$0^{\circ }$ and 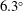$6.3^{\circ }$ for the NACA 0012 airfoil profile. Extensive acoustic measurements with smooth surface and with transition to turbulence forced by boundary layer tripping are presented. Results show that, at non-zero angle of attack, tonal noise generation is dominated by suction-side events at low Reynolds number and by pressure-side events at high Reynolds number. At smaller angle of attack, interaction between events on the two sides becomes increasingly important. Particle image velocimetry measurements complete the information on the flow field structure in the source region around the trailing edge. The influences of both angle of attack and Reynolds number on tonal noise generation are explained by changes in the mean flow topology, namely the presence and location of reverse flow regions on the two sides. Data gathered from experimental and numerical studies in the literature are reviewed and interpreted in view of the different regimes.

JFM classification

Acoustics: Aeroacoustics Boundary Layers: Boundary layer separation Vortex Flows: Vortex shedding
Type
Papers
Information
Journal of Fluid Mechanics , Volume 780 , 10 October 2015 , pp. 407 - 438
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Amiet, R. 1976 Noise due to turbulent flow past a trailing edge. J. Sound Vib. 47 (3), 387–393.Google Scholar
Arbey, H. & Bataille, J. 1983 Noise generated by airfoil profiles placed in a uniform laminar flow. J. Fluid Mech. 134, 33–47.Google Scholar
Arcondoulis, E. J. G., Doolan, C. J. & Zander, A. C. 2009 Airfoil noise measurements at various angles of attack and low Reynolds number. In Proceedings of ACOUSTICS 2009. Australian Acoustical Society.Google Scholar
Arcondoulis, E. J. G., Doolan, C. J., Zander, A. C. & Brooks, L. A. 2010 A review of trailing edge noise generated by airfoils at low to moderate Reynolds number. Acoust. Aust. 38 (3), 387–393.Google Scholar
Arcondoulis, E. J. G., Doolan, C. J., Zander, A. C. & Brooks, L. A. 2013 An experimental investigation of airfoil tonal noise caused by an acoustic feedback loop. In Proceedings of ACOUSTICS 2013. Australian Acoustical Society.Google Scholar
Atobe, T., Tuinstra, M. & Takagi, S. 2009 Airfoil tonal noise generation in resonant environments. Trans. Japan. Soc. Aeronaut. Space Sci. 52 (176), 74–80.Google Scholar
Boutilier, M. & Yarusevych, S. 2012 Parametric study of separation and transition characteristics over an airfoil at low Reynolds number. Exp. Fluids 52 (6), 1491–1506.CrossRefGoogle Scholar
Brooks, T. F., Marcolini, M. A. & Pope, D. S. 1986 Airfoil trailing-edge flow measurements. AIAA J. 24 (8), 1245–1251.Google Scholar
Brooks, T. F., Pope, D. S. & Marcolini, M. A.1989 Airfoil self-noise and prediction. Tech. Rep. 1218. NASA Reference Publication.Google Scholar
Chong, T. P. & Joseph, P. F. 2012 ‘Ladder’ structure in tonal noise generated by laminar flow around an airfoil. J. Acoust. Soc. Am. 131 (6), 461–467.CrossRefGoogle ScholarPubMed
Chong, T. P. & Joseph, P. F. 2013 An experimental study of airfoil instability tonal noise with trailing edge serrations. J. Sound Vib. 332, 6335–6358.CrossRefGoogle Scholar
Desquesnes, G., Terracol, M. & Sagaut, P. 2007 Numerical investigation of the tone noise mechanism over laminar airfoils. J. Fluid Mech. 591, 155–182.Google Scholar
Drela, M.1989 XFOIL: an analysis and design system for low Reynolds number airfoils. In Conference on Low Reynolds Number Airfoil Aerodynamics, University of Notre Dame. It also appeared as chapter in: Low Reynolds Number Aerodynamics (ed T. J. Mueller), Lecture Notes in Engineering, vol. 54. Springer.CrossRefGoogle Scholar
Fink, M. R. 1975 Prediction of airfoil tone frequencies. J. Aircraft 12, 118–120.Google Scholar
Fosas de Pando, M., Schmid, P. J. & Sipp, D. 2014 A global analysis of tonal noise in flows around aerofoils. J. Fluid Mech. 754, 5–38.CrossRefGoogle Scholar
Golubev, V. V., Nguyen, L., Mankbadi, R., Roger, M. & Visbal, M. R. 2013 Acoustic feedback-loop interactions in transitional airfoils. In Proceedings of the 19th AIAA/CEAS Aeroacoustics Conference. AIAA/CEAS.Google Scholar
Golubev, V. V., Nguyen, L., Mankbadi, R. R., Roger, M. & Visbal, M. R. 2014 On flow–acoustic resonant interactions in transitional airfoils. Intl J. Aeroacoust. 13 (1), 1–38.Google Scholar
Ikeda, T., Atobe, T. & Takagi, S. 2012 Direct simulations of trailing-edge noise generation from two-dimensional airfoils at low Reynolds numbers. J. Sound Vib. 331 (3), 556–574.Google Scholar
Inasawa, A., Kamijo, T. & Asai, M. 2010 Generation mechanism of trailing-edge noise of airfoil at low Reynolds numbers. In Proceedings of the 13th Asian Congress of Fluid Mechanics, pp. 125–128. Bangladesh Society of Mechanical Engineers.Google Scholar
Jones, L. E. & Sandberg, R. D. 2011 Numerical analysis of tonal airfoil self-noise and acoustic feedback-loops. J. Sound Vib. 330, 6137–6152.CrossRefGoogle Scholar
Kingan, M. J. & Pearse, J. R. 2009 Laminar boundary layer instability noise produced by an aerofoil. J. Sound Vib. 322, 808–828.Google Scholar
Lowson, M. V., Fiddes, S. P. & Nash, E. C. 1994 Laminar boundary layer aeroacoustic instabilities. In Proceedings of 32nd Aerospace Sciences Meeting and Exhibit. AIAA.Google Scholar
Lowson, M. V., McAlpine, A. & Nash, E. C. 1998 The generation of boundary layer instability noise on aerofoils. In Proceedings of 36th Aerospace Sciences Meeting and Exhibit. AIAA.Google Scholar
Moreau, S. & Henner, M. 2003 Analysis of flow conditions in freejet experiments for studying airfoil self-noise. AIAA J. 41 (10), 1895–1905.Google Scholar
Mueller, T. J., Scharpf, D. F., Batill, S. M., Strebinger, R. B., Sullivan, C. J. & Subramanian, S. 1992 The design of a subsonic low-noise, low-turbulence wind tunnel for acoustic measurements. In Proceedings of 17th Aerospace Ground Testing Conference. AIAA.Google Scholar
Nash, E. C.1996 Boundary layer instability noise on aerofoils. PhD thesis, University of Bristol.Google Scholar
Nash, E. C. & Lowson, M. V. 1995 Noise due to boundary layer instabilities. In Proceedings of 1st AIAA/CEAS Aeroacoustics Conference, pp. 95–124. AIAA/CEAS.Google Scholar
Nash, E. C., Lowson, M. V. & McAlpine, A. 1999 Boundary layer instability noise on airfoils. J. Fluid Mech. 382, 27–61.CrossRefGoogle Scholar
Paterson, R. W., Vogt, P., Fink, M. R. & Munch, C. 1973 Vortex noise of isolated airfoils. J. Aircraft 10 (5), 296–302.CrossRefGoogle Scholar
Plogmann, B., Herrig, A. & Würz, W. 2013 Experimental investigations of a trailing edge noise feedback mechanism on a NACA 0012 airfoil. Exp. Fluids 54, 1480.Google Scholar
Pröbsting, S. & Scarano, F. 2014 Experimental investigation of isolated aerofoil noise. In Proceedings of 21st International Congress on Sound and Vibration. Acoustical Society of China.Google Scholar
Pröbsting, S., Serpieri, J. & Scarano, F. 2014 Experimental investigation of aerofoil tonal noise generation. J. Fluid Mech. 747, 656–687.Google Scholar
Pröbsting, S. & Yarusevych, S. 2015 Laminar separation bubble development on an airfoil emitting tonal noise. J. Fluid Mech. 780, 167–191.Google Scholar
Sandberg, R. D., Jones, L. E., Sandham, N. D. & Joseph, P. F. 2009 Direct numerical simulations of tonal noise generated by laminar flow past airfoils. J. Sound Vib. 320, 838–858.Google Scholar
Schlichting, H. & Gersten, K. 2000 Boundary-layer Theory, 8th edn. Springer.Google Scholar
Schumacher, K. L., Doolan, C. J. & Kelso, R. M. 2014a The effect of a cavity on airfoil tones. J. Sound Vib. 333, 1913–1931.Google Scholar
Schumacher, K. L., Doolan, C. J. & Kelso, R. M. 2014b The effect of acoustic forcing on an airfoil tonal noise mechanism. J. Acoust. Soc. Am. 136 (2), EL78.Google Scholar
Takagi, S. & Konishi, Y. 2010 Frequency selection mechanism of airfoil trailing-edge noise. J. Aircraft 47 (4), 1111–1116.CrossRefGoogle Scholar
Tam, C. K. W. 1974 Discrete tones of isolated airfoils. J. Acoust. Soc. Am. 55 (6), 1173–1177.Google Scholar
Tam, C. K. W. & Ju, H. 2012 Aerofoil tones at moderate Reynolds number. J. Fluid Mech. 690, 536–570.Google Scholar
Torrence, C. & Compo, G. 1998 A practical guide to wavelet analysis. Bull. Am. Meteorol. Soc. Google Scholar
Welch, P. D. 1967 The use of fast Fourier transform for the estimation of power spectra: a method based on time averaging over short, modified periodograms. IEEE Trans. Audio Electroacoust. 15, 70–73.CrossRefGoogle Scholar
Wright, S. E. 1976 The acoustic spectrum of axial flow machines. J. Sound Vib. 45 (2), 165–223.Google Scholar