Published online by Cambridge University Press: 30 March 2015
We examine the linear stability of a homogeneous gas–solid suspension of small Stokes number particles, with a moderate mass loading, subject to a simple shear flow. The modulation of the gravitational force exerted on the suspension, due to preferential concentration of particles in regions of low vorticity, in response to an imposed velocity perturbation, can lead to an algebraic instability. Since the fastest growing modes have wavelengths small compared with the characteristic length scale ( $U_{g}/{\it\Gamma}$) and oscillate with frequencies large compared with
$U_{g}/{\it\Gamma}$) and oscillate with frequencies large compared with 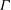 ${\it\Gamma}$,
${\it\Gamma}$, 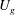 $U_{g}$ being the settling velocity and
$U_{g}$ being the settling velocity and 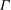 ${\it\Gamma}$ the shear rate, we apply the WKB method, a multiple scale technique. This analysis reveals the existence of a number density mode which travels due to the settling of the particles and a momentum mode which travels due to the cross-streamline momentum transport caused by settling. These modes are coupled at a turning point which occurs when the wavevector is nearly horizontal and the most amplified perturbations are those in which a momentum wave upstream of the turning point creates a downstream number density wave. The particle number density perturbations reach a finite, but large amplitude that persists after the wave becomes aligned with the velocity gradient. The growth of the amplitude of particle concentration and fluid velocity disturbances is characterised as a function of the wavenumber and Reynolds number (
${\it\Gamma}$ the shear rate, we apply the WKB method, a multiple scale technique. This analysis reveals the existence of a number density mode which travels due to the settling of the particles and a momentum mode which travels due to the cross-streamline momentum transport caused by settling. These modes are coupled at a turning point which occurs when the wavevector is nearly horizontal and the most amplified perturbations are those in which a momentum wave upstream of the turning point creates a downstream number density wave. The particle number density perturbations reach a finite, but large amplitude that persists after the wave becomes aligned with the velocity gradient. The growth of the amplitude of particle concentration and fluid velocity disturbances is characterised as a function of the wavenumber and Reynolds number (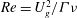 $\mathit{Re}=U_{g}^{2}/{\it\Gamma}{\it\nu}$) using both asymptotic theory and a numerical solution of the linearised equations.
$\mathit{Re}=U_{g}^{2}/{\it\Gamma}{\it\nu}$) using both asymptotic theory and a numerical solution of the linearised equations.