Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-26T15:44:28.864Z Has data issue: false hasContentIssue false
  • English
  • Français

Perturbative corrections for the scaling of heat transport in a Hele-Shaw geometry and its application to geological vertical fractures

Published online by Cambridge University Press:  11 February 2019

Juvenal A. Letelier Open the ORCID record for Juvenal A. Letelier [Opens in a new window] ,
Nicolás Mujica Open the ORCID record for Nicolás Mujica [Opens in a new window]  and
Jaime H. Ortega Open the ORCID record for Jaime H. Ortega [Opens in a new window]
Juvenal A. Letelier*
Affiliation:
Departamento de Ingeniería Civil, Recursos Hídricos y Medio Ambiente, Facultad de Ciencias Físicas y Matemáticas, Universidad de Chile, Avenida Blanco Encalada 2002, Santiago 8370449, Chile Centro de Excelencia en Geotermia de los Andes, Plaza Ercilla 803, Santiago 8370450, Chile
Nicolás Mujica
Affiliation:
Departamento de Física, Facultad de Ciencias Físicas y Matemáticas, Universidad de Chile, Avenida Blanco Encalada 2008, Santiago 8370449, Chile
Jaime H. Ortega
Affiliation:
Departamento de Ingeniería Matemática y Centro de Modelamiento Matemático, Facultad de Ciencias Físicas y Matemáticas, Universidad de Chile, Beauchef 851, Santiago 8370456, Chile
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this work, we investigate numerically the perturbative effects of cell aperture in heat transport and thermal dissipation rate for a vertical Hele-Shaw geometry, which is used as an analogue representation of a planar vertical fracture at the laboratory scale. To model the problem, we derive a two-dimensional set of equations valid for this geometry. 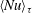For Hele-Shaw cells heated from below and above, with periodic boundary conditions in the horizontal direction, the model gives new nonlinear scalings for both the time-averaged Nusselt number $\langle Nu\rangle _{\unicode[STIX]{x1D70F}}$ and dimensionless mean thermal dissipation rate $\langle \unicode[STIX]{x1D717}\rangle _{\unicode[STIX]{x1D70F}}$ in the high-Rayleigh regime. We demonstrate that 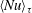$\langle Nu\rangle _{\unicode[STIX]{x1D70F}}$ and 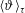$\langle \unicode[STIX]{x1D717}\rangle _{\unicode[STIX]{x1D70F}}$ depend upon the cell anisotropy ratio $\unicode[STIX]{x1D716}$, which measures the ratio between the cell gap and height. We show that 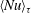$\langle Nu\rangle _{\unicode[STIX]{x1D70F}}$ values in the high-Rayleigh regime decrease when $\unicode[STIX]{x1D716}$ grows, supporting the field observations at the fracture scale. When 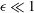$\unicode[STIX]{x1D716}\ll 1$, our results are in agreement with the scalings found using the Darcy model. The numerical results satisfy the theoretical relation 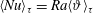$\langle Nu\rangle _{\unicode[STIX]{x1D70F}}=Ra\langle \unicode[STIX]{x1D717}\rangle _{\unicode[STIX]{x1D70F}}$, which is obtained from the model. This latter relation is valid for all values of Rayleigh number considered. The perturbative effects of cell aperture are observed only in the exponents of the scalings 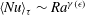$\langle Nu\rangle _{\unicode[STIX]{x1D70F}}\sim Ra^{\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D716})}$ and 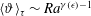$\langle \unicode[STIX]{x1D717}\rangle _{\unicode[STIX]{x1D70F}}\sim Ra^{\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D716})-1}$.

JFM classification

Convection: Convection in porous media Low-Reynolds-number flows: Hele-Shaw flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 864 , 10 April 2019 , pp. 746 - 767
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adams, B. M., Kuehn, T. H., Bielicki, J. M., Randolph, J. B. & Saar, M. O. 2014 On the importance of the thermosiphon effect in CPG (CO2 plume geothermal) power systems. Energy 69, 409–418.Google Scholar
Ahlers, G., Funfschilling, D. & Bodenschatz, E. 2009 Transitions in heat transport by turbulent convection at Rayleigh numbers up to 1015 . New J. Phys. 11, 123001.Google Scholar
Backhaus, S., Turitsyn, K. & Ecke, R. E. 2011 Convective instability and mass transport of diffusion layers in a Hele-Shaw geometry. Phys. Rev. Lett. 106, 104501.Google Scholar
Battistelli, A., Calore, C. & Pruess, K. 1997 The simulator TOUGH2/EWASG for modelling geothermal reservoirs with brines and non-condensible gas. Geothermics 26 (4), 437–464.Google Scholar
Benson, S., Cook, P. et al. 2006 Underground geological storage. In Carbon Dioxide Capture and Storage. Special Report of the Intergovernmental Panel on Climate Change (ed. Metz, B., Davidson, O., de Coninck, H., Loos, M. & Meyer, L.), pp. 195–276. Cambridge University Press.Google Scholar
Bizon, C., Werne, J., Predtechensky, A. A., Julien, K., McCormick, W. D., Swift, J. B. & Swinney, H. L. 1997 Plume dynamics in quasi-2D turbulent convection. Chaos 7, 107–124.Google Scholar
Brown, D. 2000 A hot dry rock geothermal energy concept utilizing supercritical CO2 instead of water. In Proceedings of the Twenty-Fifth Workshop on Geothermal Reservoir Engineering, pp. 233–238. Stanford University.Google Scholar
Cherkaoui, A. & Wilcock, W. 2001 Laboratory studies of high Rayleigh number circulation in an open-top Hele-Shaw cell: an analog to mid-ocean ridge hydrothermal systems. J. Geophys. Res. 106, 10983.Google Scholar
Cooper, C., Crews, J., Schumer, R., Breitmeyer, R., Voepel, H. & Decker, D. 2014 Experimental investigation of transient thermal convection in porous media. Trans. Porous Med. 104, 335–347.Google Scholar
Croucher, A. & O’Sullivan, M. 2008 Application of the computer code TOUGH2 to the simulation of supercritical conditions in geothermal systems. Geothermics 37, 622–634.Google Scholar
Davies, G. F. 1999 Dynamic Earth: Plates, Plumes and Mantle Convection. Cambridge University Press.Google Scholar
Elder, J. W. 1967 Steady free convection in a porous medium heated from below. J. Fluid Mech. 27, 29–48.Google Scholar
Foster, T. D. 1965 Stability of a homogeneous fluid cooled uniformly from above. Phys. Fluids 8, 1249–1257.Google Scholar
Gondret, P. & Rabaud, M. 1997 Shear instability of two-fluid parallel flow in a Hele-Shaw cell. Phys. Fluids 9, 3267–3274.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23, 045108.Google Scholar
Hewitt, D. R., Neufeld, J. A. & Lister, J. R. 2012 Ultimate regime of high Rayleigh number convection in a porous medium. Phys. Rev. Lett. 108, 224503.Google Scholar
Hidalgo, J. J., Fe, J., Cueto-Felgueroso, L. & Juanes, R. 2012 Scaling of convective mixing in porous media. Phys. Rev. Lett. 109, 264503.Google Scholar
Jenny, P., Lee, J. S., Meyer, D. W. & Tchelepi, H. A. 2014 Scale analysis of miscible density-driven convection in porous media. J. Fluid Mech. 749, 519–541.Google Scholar
Jha, B., Cueto-Felgueroso, L. & Juanes, R. 2011 Quantifying mixing in viscously unstable porous media flows. Phys. Rev. E 84, 066312.Google Scholar
Jha, B., Cueto-Felgueroso, L. & Juanes, R. 2013 Synergetic fluid mixing from viscous fingering and alternating injection. Phys. Rev. Lett. 111, 144501.Google Scholar
Joseph, D., Huang, A. & Hu, H. 1996 Non-solenoidal velocity effects and Korteweg stresses in simple mixtures of incompressible liquids. Physica D 97, 104–125.Google Scholar
Kvernvold, O. & Tyvand, P. A. 1981 Dispersion effects on thermal convection in a Hele-Shaw cell. Intl J. Heat Mass Transfer 24, 887–890.Google Scholar
Lele, S. K. 1992 Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103, 16–42.Google Scholar
Letelier, J. A., Herrera, P., Mujica, N. & Ortega, J. H. 2016 Enhancement of synthetic schlieren image resolution using total variation optical flow: application to thermal experiments in a Hele-Shaw cell. Exp. Fluids 57 (2), 1–14.Google Scholar
López, D. L. & Smith, L. 1995 Fluid flow in fault zones: analysis of the interplay of convective circulation and topographically driven groundwater flow. Water Resour. Res. 31, 1489–1503.Google Scholar
Malkus, W. V. 1954 The heat transport and spectrum of thermal turbulence. Proc. R. Soc. Lond. A 225, 196–212.Google Scholar
Murphy, H. D. 1979 Convective instabilities in vertical fractures and faults. J. Geophys. Res. 84, 6121–6130.Google Scholar
Neufeld, J. A., Hesse, M. A., Riaz, A., Hallworth, M. A., Tchelepi, H. A. & Huppert, H. E. 2010 Convective dissolution of carbon dioxide in saline aquifers. Geophys. Res. Lett. 37, L22404.Google Scholar
Nield, D. & Bejan, A. 2006 Convection in Porous Media, 3rd edn. Springer.Google Scholar
Nigon, B., Englert, A. & Pascal, C. 2015 Modeling to heat transport through fractures with emphasis to roughness and aperture variability. In EGU General Assembly Conf. Abstracts, vol. 17, p. 8821.Google Scholar
Oltean, C., Felder, C. H., Panfilov, M. & Bues, M. A. 2004 Transport with a very low density contrast in Hele-Shaw cell and porous medium: evolution of the mixing zone. Trans. Porous Med. 55, 339–360.Google Scholar
Oltean, C., Golfier, C. & Bues, M. A. 2008 Experimental and numerical study of the validity of Hele-Shaw cell as analogue model for variable-density flow in homogeneous porous media. Adv. Water Resour. 31, 82–95.Google Scholar
Otero, J., Dontcheva, L. A., Johnston, H., Worthing, R. A., Kurganov, A., Petrova, G. & Doering, C. R. 2004 High Rayleigh number convection in a fluid-saturated porous layer. J. Fluid Mech. 500, 263–281.Google Scholar
Palm, E., Weber, J. E. & Kvernvold, O. 1972 On steady convection in a porous medium. J. Fluid Mech. 54, 153–161.Google Scholar
Pramanik, S. & Mishra, M. 2015 Viscosity scaling of fingering instability in finite slices with Korteweg stress. Eur. Phys. Lett. 109, 64001.Google Scholar
Pruess, K.1991 TOUGH2: a general-purpose numerical simulator for multiphase nonisothermal flows. Tech. Rep. Lawrence Berkeley Laboratory, CA.Google Scholar
Randolph, J. B. & Saar, M. O. 2011 Coupling carbon dioxide sequestration with geothermal energy capture in naturally permeable, porous geologic formations: implications for CO2 sequestration. Energy Proc. 4, 2206–2213.Google Scholar
Riaz, A., Hesse, M., Tchelepi, H. A. & Orr, F. M. 2006 Onset of convection in a gravitationally unstable diffusive boundary layer in porous media. J. Fluid Mech. 548, 87–111.Google Scholar
Ruyer-Quil, C. 2001 Inertial corrections to the Darcy law in a Hele-Shaw cell. C. R. Acad. Sci. Paris IIb 329, 337–342.Google Scholar
Scheck-Wenderoth, M., Cacace, M., Petrovich, Y., Cherubini, Y., Noack, V., Onno, B., Sippel, J. & Bjorn, L. 2014 Models of heat transport in the Central European Basin System: effective mechanisms at different scales. Mar. Petrol. Geol. 55, 315–331.Google Scholar
Scheidegger, A. E. 1974 The Physics of Flow through Porous Media. University of Toronto Press.Google Scholar
Schoofs, S., Spera, F. & Hansen, U. 1999 Chaotic thermohaline convection in low-porosity hydrothermal systems. Earth Planet. Sci. Lett. 174, 213–229.Google Scholar
Sheu, L., Tam, L., Chen, J., Chen, H., Lin, K. & Kang, Y. 2008 Chaotic convection of viscoelastic fluids in porous media. Chaos, Solitons Fractals 37, 113–124.Google Scholar
Waleffe, F., Boonkasane, A. & Smith, L. 2015 Heat transport by coherent Rayleigh–Bénard convection. Phys. Fluids 27, 051702.Google Scholar
Winters, K. B. & de la Fuente, A. 2012 Modelling rotating stratified flows at laboratory-scale using spectrally-based DNS. Ocean Model. 49–50, 47–59.Google Scholar
Zhao, C., Hoobs, B. E. & Ord, A. 2008 Convective and Advective Heat Transfer in Geological Systems, chap. 9. Springer.Google Scholar