Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-27T01:32:46.621Z Has data issue: false hasContentIssue false
  • English
  • Français

Optimal heat transport solutions for Rayleigh–Bénard convection

Published online by Cambridge University Press:  06 November 2015

David Sondak ,
Leslie M. Smith  and
Fabian Waleffe
David Sondak*
Affiliation:
Department of Mathematics, University of Wisconsin-Madison, Madison, WI 53706, USA
Leslie M. Smith
Affiliation:
Department of Mathematics, University of Wisconsin-Madison, Madison, WI 53706, USA Department of Engineering Physics, University of Wisconsin-Madison, Madison, WI 53706, USA
Fabian Waleffe
Affiliation:
Department of Mathematics, University of Wisconsin-Madison, Madison, WI 53706, USA Department of Engineering Physics, University of Wisconsin-Madison, Madison, WI 53706, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

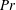Steady flows that optimize heat transport are obtained for two-dimensional Rayleigh–Bénard convection with no-slip horizontal walls for a variety of Prandtl numbers $\mathit{Pr}$ and Rayleigh number up to 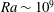$\mathit{Ra}\sim 10^{9}$. Power-law scalings of $\mathit{Nu}\sim \mathit{Ra}^{{\it\gamma}}$ are observed with 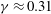${\it\gamma}\approx 0.31$, where the Nusselt number $\mathit{Nu}$ is a non-dimensional measure of the vertical heat transport. Any dependence of the scaling exponent on 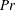$\mathit{Pr}$ is found to be extremely weak. On the other hand, the presence of two local maxima of $\mathit{Nu}$ with different horizontal wavenumbers at the same 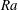$\mathit{Ra}$ leads to the emergence of two different flow structures as candidates for optimizing the heat transport. For 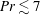$\mathit{Pr}\lesssim 7$, optimal transport is achieved at the smaller maximal wavenumber. In these fluids, the optimal structure is a plume of warm rising fluid, which spawns left/right horizontal arms near the top of the channel, leading to downdraughts adjacent to the central updraught. For 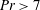$\mathit{Pr}>7$ at high enough 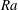$\mathit{Ra}$, the optimal structure is a single updraught lacking significant horizontal structure, and characterized by the larger maximal wavenumber.

JFM classification

Mathematical Foundations: Computational methods Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 784 , 10 December 2015 , pp. 565 - 595
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.Google Scholar
Amati, G., Koal, K., Massaioli, F., Sreenivasan, K. R. & Verzicco, R. 2005 Turbulent thermal convection at high Rayleigh numbers for a Boussinesq fluid of constant Prandtl number. Phys. Fluids 17, 121701.CrossRefGoogle Scholar
Ascher, U. M., Ruuth, S. J. & Wetton, B. T. R 1995 Implicit–explicit methods for time-dependent partial differential equations. SIAM J. Numer. Anal. 32 (3), 797–823.Google Scholar
Bailon-Cuba, J., Emran, M. S. & Schumacher, J. 2010 Aspect ratio dependence of heat transfer and large-scale flow in turbulent convection. J. Fluid Mech. 655, 152–173.Google Scholar
Breuer, M., Wessling, S., Schmalzl, J. & Hansen, U. 2004 Effect of inertia in Rayleigh–Bénard convection. Phys. Rev. E 69 (2), 026302.Google Scholar
Busse, F. H. 1969 On Howard’s upper bound for heat transport by turbulent convection. J. Fluid Mech. 37 (3), 457–477.Google Scholar
Chandrasekhar, S. 2013 Hydrodynamic and Hydromagnetic Stability. Dover.Google Scholar
Chavanne, X., Chilla, F., Chabaud, B., Castaing, B. & Hebral, B. 2001 Turbulent Rayleigh–Bénard convection in gaseous and liquid He. Phys. Fluids 13 (5), 1300–1320.Google Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35 (7), 1–25.Google Scholar
Constantin, P. & Doering, C. R. 1995 Variational bounds on energy dissipation in incompressible flows. II. Channel flow. Phys. Rev. E 51 (4), 3192–3198.Google Scholar
Dennis, J. E. & Schnabel, R. B. 1996 Numerical Methods for Unconstrained Optimization and Nonlinear Equations. vol. 16. SIAM.CrossRefGoogle Scholar
Doering, C. R. & Constantin, P. 1992 Energy dissipation in shear driven turbulence. Phys. Rev. Lett. 69 (11), 1648–1651.Google Scholar
Doering, C. R. & Constantin, P. 1994 Variational bounds on energy dissipation in incompressible flows: shear flow. Phys. Rev. E 49 (5), 4087–4099.CrossRefGoogle ScholarPubMed
Doering, C. R. & Constantin, P. 1996 Variational bounds on energy dissipation in incompressible flows. III: Convection. Phys. Rev. E 53 (6), 5957–5981.Google Scholar
Doering, C. R., Otto, F. & Reznikoff, M. G. 2006 Bounds on vertical heat transport for infinite-Prandtl-number Rayleigh–Bénard convection. J. Fluid Mech. 560, 229–241.CrossRefGoogle Scholar
Drazin, P. G. & Reid, W. H. 2004 Hydrodynamic Stability. Cambridge University Press.CrossRefGoogle Scholar
Emran, M. S. & Schumacher, J. 2015 Large-scale mean patterns in turbulent convection. J. Fluid Mech. 776, 96–108.CrossRefGoogle Scholar
Gibson, J. F., Halcrow, J. & Cvitanović, P. 2008 Visualizing the geometry of state space in plane Couette flow. J. Fluid Mech. 611, 107–130.Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl numbers. Phys. Rev. Lett. 86 (15), 3316–3319.Google Scholar
Grossmann, S. & Lohse, D. 2002 Prandtl and Rayleigh number dependence of the Reynolds number in turbulent thermal convection. Phys. Rev. E 66 (1), 016305.Google Scholar
Grossmann, S. & Lohse, D. 2004 Fluctuations in turbulent Rayleigh–Bénard convection: the role of plumes. Phys. Fluids 16 (12), 4462–4472.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23 (4), 045108.Google Scholar
Grötzbach, G. 1983 Spatial resolution requirements for direct numerical simulation of the Rayleigh–Bénard convection. J. Comput. Phys. 49 (2), 241–264.Google Scholar
Hassanzadeh, P., Chini, G. P. & Doering, C. R. 2014 Wall to wall optimal transport. J. Fluid Mech. 751, 627–662.Google Scholar
He, X., Funfschilling, D., Bodenschatz, E. & Ahlers, G. 2012a Heat transport by turbulent Rayleigh–Bénard convection for $Pr\approx 0.8$ and $4\times 10^{11}\lesssim Ra\lesssim 2\times 10^{14}$ : ultimate-state transition for aspect ratio ${\it\Gamma}=1.00$ . New J. Phys. 14 (6), 063030.Google Scholar
He, X., Funfschilling, D., Nobach, H., Bodenschatz, E. & Ahlers, G. 2012b Transition to the ultimate state of turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 108 (2), 024502.Google Scholar
Howard, L. N. 1963 Heat transport by turbulent convection. J. Fluid Mech. 17 (3), 405–432.Google Scholar
Jeffreys, H. 1928 Some cases of instability in fluid motion. Proc. R. Soc. Lond. A 118, 195–208.Google Scholar
Kerswell, R. R. 2001 New results in the variational approach to turbulent Boussinesq convection. Phys. Fluids 13 (1), 192–209.Google Scholar
Kim, J., Moin, P. & Moser, R. 1987 Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 177, 133–166.Google Scholar
Knoll, D. A & Keyes, D. E. 2004 Jacobian-free Newton–Krylov methods: a survey of approaches and applications. J. Comput. Phys. 193 (2), 357–397.Google Scholar
Kraichnan, R. H. 1962 Turbulent thermal convection at arbitrary Prandtl number. Phys. Fluids 5 (11), 1374–1389.Google Scholar
Lappa, M. 2009 Thermal Convection: Patterns, Evolution and Stability. John Wiley & Sons.Google Scholar
Malkus, W. V. R. 1954 The heat transport and spectrum of thermal turbulence. Proc. R. Soc. Lond. A 225 (1161), 196–212.Google Scholar
Net, M., Alonso, A. & Sánchez, J. 2003 From stationary to complex time-dependent flows in two-dimensional annular thermal convection at moderate Rayleigh numbers. Phys. Fluids 15 (5), 1314–1326.Google Scholar
Niemela, J. J., Skrbek, L., Sreenivasan, K. R. & Donnelly, R. J. 2000 Turbulent convection at very high Rayleigh numbers. Nature 404 (6780), 837–840.Google Scholar
Niemela, J. J. & Sreenivasan, K. R. 2006 Turbulent convection at high Rayleigh numbers and aspect ratio 4. J. Fluid Mech. 557, 411–422.Google Scholar
Pellew, A. & Southwell, R. V. 1940 On maintained convective motion in a fluid heated from below. Proc. R. Soc. Lond. A 176 (966), 312–343.Google Scholar
van der Poel, E. P., Ostilla-Mónico, R., Donners, J. & Verzicco, R. 2015 A pencil distributed finite difference code for strongly turbulent wall-bounded flows. Comput. Fluids 116, 10–16.Google Scholar
van der Poel, E. P., Stevens, R. J. A. M. & Lohse, D. 2013 Comparison between two- and three-dimensional Rayleigh–Bénard convection. J. Fluid Mech. 736, 177–194.CrossRefGoogle Scholar
Reid, W. H. & Harris, D. L. 1958 Some further results on the Bénard problem. Phys. Fluids 1 (2), 102–110.Google Scholar
Roche, P.-E., Castaing, B., Chabaud, B. & Hébral, B. 2001 Observation of the $1/2$ power law in Rayleigh–Bénard convection. Phys. Rev. E 63 (4), 045303.CrossRefGoogle Scholar
Saad, Y. & Schultz, M. H. 1986 GMRES: a generalized minimal residual algorithm for solving nonsymmetric linear systems. SIAM J. Sci. Stat. Comput. 7 (3), 856–869.Google Scholar
Sánchez, J., Net, M., Garcıa-Archilla, B. & Simó, C. 2004 Newton–Krylov continuation of periodic orbits for Navier–Stokes flows. J. Comput. Phys. 201 (1), 13–33.Google Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.Google Scholar
Shraiman, B. I. & Siggia, E. D. 1990 Heat transport in high-Rayleigh-number convection. Phys. Rev. A 42 (6), 3650–3653.Google Scholar
Siggia, E. D. 1994 High Rayleigh number convection. Annu. Rev. Fluid Mech. 26 (1), 137–168.Google Scholar
Smith, T. R., Moehlis, J. & Holmes, P. 2005 Low-dimensional modelling of turbulence using the proper orthogonal decomposition: a tutorial. Nonlinear Dyn. 41 (1–3), 275–307.Google Scholar
Stevens, R. J. A. M., van der Poel, E. P., Grossmann, S. & Lohse, D. 2013 The unifying theory of scaling in thermal convection: the updated prefactors. J. Fluid Mech. 730, 295–308.Google Scholar
Stretch, D. D.1990 Automated pattern eduction from turbulent flow diagnostics. Annual Research Briefs-1990, pp. 145–157. Center for Turbulence Research, Stanford University.Google Scholar
Stringano, G. & Verzicco, R. 2006 Mean flow structure in thermal convection in a cylindrical cell of aspect ratio one half. J. Fluid Mech. 548, 1–16.Google Scholar
Trefethen, L. N & Bau III, D. 1997 Numerical Linear Algebra. vol. 50. SIAM.Google Scholar
Verzicco, R. & Camussi, R. 1999 Prandtl number effects in convective turbulence. J. Fluid Mech. 383, 55–73.Google Scholar
Verzicco, R. & Camussi, R. 2003 Numerical experiments on strongly turbulent thermal convection in a slender cylindrical cell. J. Fluid Mech. 477, 19–49.Google Scholar
Verzicco, R. & Sreenivasan, K. R. 2008 A comparison of turbulent thermal convection between conditions of constant temperature and constant heat flux. J. Fluid Mech. 595, 203–219.Google Scholar
Viswanath, D. 2007 Recurrent motions within plane Couette turbulence. J. Fluid Mech. 580, 339–358.Google Scholar
Waleffe, F. 2009 Exact coherent structures in turbulent shear flows. In Turbulence and Interactions, pp. 139–158. Springer.Google Scholar
Waleffe, F., Boonkasame, A. & Smith, L. M. 2015 Heat transport by coherent Rayleigh–Bénard convection. Phys. Fluids 27 (5), 051702.Google Scholar
Wen, B., Chini, G. P., Kerswell, R. R. & Doering, C. R. 2015a Time-stepping approach for solving upper-bound problems: application to two-dimensional Rayleigh–Bénard convection. Phys. Rev. E 92 (4), 043012.Google Scholar
Wen, B., Corson, L. T. & Chini, G. P. 2015b Structure and stability of steady porous medium convection at large Rayleigh number. J. Fluid Mech. 772, 197–224.Google Scholar
Whitehead, J. P. & Doering, C. R. 2011 Ultimate state of two-dimensional Rayleigh–Bénard convection between free-slip fixed-temperature boundaries. Phys. Rev. Lett. 106 (24), 244501.Google Scholar
Whitehead, J. P. & Wittenberg, R. W. 2014 A rigorous bound on the vertical transport of heat in Rayleigh–Bénard convection at infinite Prandtl number with mixed thermal boundary conditions. J. Math. Phys. 55 (9), 093104.Google Scholar