Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-19T03:54:03.422Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Reynolds number dependence of velocity-gradient structure and dynamics

Published online by Cambridge University Press:  19 December 2018

Rishita Das Open the ORCID record for Rishita Das [Opens in a new window]  and
Sharath S. Girimaji
Rishita Das*
Affiliation:
Department of Aerospace Engineering, Texas A&M University, College Station, TX 77843, USA
Sharath S. Girimaji
Affiliation:
Department of Aerospace Engineering, Texas A&M University, College Station, TX 77843, USA Department of Ocean Engineering, Texas A&M University, College Station, TX 77843, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

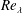We seek to examine the changes in velocity-gradient structure (local streamline topology) and related dynamics as a function of Reynolds number ($Re_{\unicode[STIX]{x1D706}}$). The analysis factorizes the velocity gradient (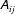$\unicode[STIX]{x1D608}_{ij}$) into the magnitude (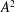$A^{2}$) and normalized-gradient tensor (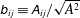$\unicode[STIX]{x1D623}_{ij}\equiv \unicode[STIX]{x1D608}_{ij}/\sqrt{A^{2}}$). The focus is on bounded 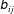$\unicode[STIX]{x1D623}_{ij}$ as (i) it describes small-scale structure and local streamline topology, and (ii) its dynamics is shown to determine magnitude evolution. Using direct numerical simulation (DNS) data, the moments and probability distributions of 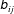$\unicode[STIX]{x1D623}_{ij}$ and its scalar invariants are shown to attain 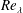$Re_{\unicode[STIX]{x1D706}}$ independence. The critical values beyond which each feature attains 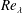$Re_{\unicode[STIX]{x1D706}}$ independence are established. We proceed to characterize the 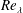$Re_{\unicode[STIX]{x1D706}}$ dependence of 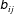$\unicode[STIX]{x1D623}_{ij}$-conditioned statistics of key non-local pressure and viscous processes. Overall, the analysis provides further insight into velocity-gradient dynamics and offers an alternative framework for investigating intermittency, multifractal behaviour and for developing closure models.

JFM classification

Turbulent Flows: Intermittency Turbulent Flows: Isotropic turbulence Turbulent Flows: Turbulent Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 861 , 25 February 2019 , pp. 163 - 179
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ashurst, W. T., Kerstein, A. R., Kerr, R. M. & Gibson, C. H. 1987 Alignment of vorticity and scalar gradient with strain rate in simulated Navier–Stokes turbulence. Phys. Fluids 30 (8), 2343–2353.Google Scholar
Bechlars, P. & Sandberg, R. D. 2017 Variation of enstrophy production and strain rotation relation in a turbulent boundary layer. J. Fluid Mech. 812, 321–348.Google Scholar
Bikkani, R. K. & Girimaji, S. S. 2007 Role of pressure in nonlinear velocity gradient dynamics in turbulence. Phys. Rev. E 75 (3), 036307.Google Scholar
Blackburn, H. M., Mansour, N. N. & Cantwell, B. J. 1996 Topology of fine-scale motions in turbulent channel flow. J. Fluid Mech. 310, 269–292.Google Scholar
Cantwell, B. J. 1992 Exact solution of a restricted Euler equation for the velocity gradient tensor. Phys. Fluids A 4 (4), 782–793.Google Scholar
Chevillard, L. & Meneveau, C. 2006 Lagrangian dynamics and statistical geometric structure of turbulence. Phys. Rev. Lett. 97 (17), 174501.Google Scholar
Chevillard, L., Meneveau, C., Biferale, L. & Toschi, F. 2008 Modeling the pressure Hessian and viscous Laplacian in turbulence: comparisons with direct numerical simulation and implications on velocity gradient dynamics. Phys. Fluids 20 (10), 101504.Google Scholar
Chong, M. S., Perry, A. E. & Cantwell, B. J. 1990 A general classification of three-dimensional flow fields. Phys. Fluids A 2 (5), 765–777.Google Scholar
Danish, M., Suman, S. & Girimaji, S. S. 2016 Influence of flow topology and dilatation on scalar mixing in compressible turbulence. J. Fluid Mech. 793, 633–655.Google Scholar
Donzis, D. A., Sreenivasan, K. R. & Yeung, P. K. 2005 Scalar dissipation rate and dissipative anomaly in isotropic turbulence. J. Fluid Mech. 532, 199–216.Google Scholar
Donzis, D. A., Yeung, P. K. & Sreenivasan, K. R. 2008 Dissipation and enstrophy in isotropic turbulence: resolution effects and scaling in direct numerical simulations. Phys. Fluids 20 (4), 045108.Google Scholar
Donzis, D. A. & Sreenivasan, K. R. 2010 Short-term forecasts and scaling of intense events in turbulence. J. Fluid Mech. 647, 13–26.Google Scholar
Girimaji, S. S. & Pope, S. B. 1990 A diffusion model for velocity gradients in turbulence. Phys. Fluids A 2 (2), 242–256.Google Scholar
Girimaji, S. S. & Speziale, C. G. 1995 A modified restricted Euler equation for turbulent flows with mean velocity gradients. Phys. Fluids 7 (6), 1438–1446.Google Scholar
Jeong, E. & Girimaji, S. S. 2003 Velocity-gradient dynamics in turbulence: effect of viscosity and forcing. Theor. Comput. Fluid Dyn. 16 (6), 421–432.Google Scholar
Johnson, P. L. & Meneveau, C. 2016 A closure for Lagrangian velocity gradient evolution in turbulence using recent-deformation mapping of initially Gaussian fields. J. Fluid Mech. 804, 387–419.Google Scholar
Kailasnath, P., Sreenivasan, K. R. & Stolovitzky, G. 1992 Probability density of velocity increments in turbulent flows. Phys. Rev. Lett. 68 (18), 2766–2769.Google Scholar
Kaneda, Y., Ishihara, T., Yokokawa, M., Itakura, K. & Uno, A. 2003 Energy dissipation rate and energy spectrum in high resolution direct numerical simulations of turbulence in a periodic box. Phys. Fluids 15 (2), L21–L24.Google Scholar
Li, Y., Perlman, E., Wan, M., Yang, Y., Meneveau, C., Burns, R., Chen, S., Szalay, A. & Eyink, G. 2008 A public turbulence database cluster and applications to study Lagrangian evolution of velocity increments in turbulence. J. Turbul. 9, N31.Google Scholar
Martín, J., Dopazo, C. & Valiño, L. 1998a Dynamics of velocity gradient invariants in turbulence: restricted Euler and linear diffusion models. Phys. Fluids 10 (8), 2012–2025.Google Scholar
Martín, J., Ooi, A., Chong, M. S. & Soria, J. 1998b Dynamics of the velocity gradient tensor invariants in isotropic turbulence. Phys. Fluids 10 (9), 2336–2346.Google Scholar
Meneveau, C. 2011 Lagrangian dynamics and models of the velocity gradient tensor in turbulent flows. Annu. Rev. Fluid Mech. 43, 219–245.Google Scholar
Pereira, R. M., Garban, C. & Chevillard, L. 2016 A dissipative random velocity field for fully developed fluid turbulence. J. Fluid Mech. 794, 369–408.Google Scholar
Pereira, R. M., Moriconi, L. & Chevillard, L. 2018 A multifractal model for the velocity gradient dynamics in turbulent flows. J. Fluid Mech. 839, 430–467.Google Scholar
Schumacher, J., Scheel, J. D., Krasnov, D., Donzis, D. A., Yakhot, V. & Sreenivasan, K. R. 2014 Small-scale universality in fluid turbulence. Proc. Natl Acad. Sci. USA 111 (30), 10961–10965.Google Scholar
Soria, J., Sondergaard, R., Cantwell, B. J., Chong, M. S. & Perry, A. E. 1994 A study of the fine-scale motions of incompressible time-developing mixing layers. Phys. Fluids 6 (2), 871–884.Google Scholar
Sreenivasan, K. R. 1998 An update on the energy dissipation rate in isotropic turbulence. Phys. Fluids 10 (2), 528–529.Google Scholar
Suman, S. & Girimaji, S. S. 2010 Velocity gradient invariants and local flow-field topology in compressible turbulence. J. Turbul. 11 (2), N2.Google Scholar
Vieillefosse, P. 1982 Local interaction between vorticity and shear in a perfect incompressible fluid. J. Phys. 43 (6), 837–842.Google Scholar
Wang, X., Szalay, A., Aragón-Calvo, M. A., Neyrinck, M. C. & Eyink, G. L. 2014 Kinematic morphology of large-scale structure: evolution from potential to rotational flow. Astrophys. J. 793 (1), 58.Google Scholar
Yakhot, V. & Donzis, D. 2017 Emergence of multiscaling in a random-force stirred fluid. Phys. Rev. Lett. 119 (4), 044501.Google Scholar
Yakhot, V. & Sreenivasan, K. R. 2005 Anomalous scaling of structure functions and dynamic constraints on turbulence simulations. J. Stat. Phys. 121 (5–6), 823–841.Google Scholar
Yeung, P. K., Zhai, X. M. & Sreenivasan, K. R. 2015 Extreme events in computational turbulence. Proc. Natl Acad. Sci. USA 112 (41), 12633–12638.Google Scholar