Published online by Cambridge University Press: 19 May 2014
A model is proposed for predicting the presence of cumulative nonlinear distortions in the acoustic waveforms produced by high-speed jet flows. The model relies on the conventional definition of the acoustic shock formation distance and employs an effective Gol’dberg number 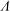 $\Lambda $ for diverging acoustic waves. The latter properly accounts for spherical spreading, whereas the classical Gol’dberg number
$\Lambda $ for diverging acoustic waves. The latter properly accounts for spherical spreading, whereas the classical Gol’dberg number 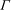 $\Gamma $ is restricted to plane wave applications. Scaling laws are then derived to account for the effects imposed by jet exit conditions of practical interest and includes Mach number, temperature ratio, Strouhal number and an absolute observer distance relative to a broadband Gaussian source. Surveys of the acoustic pressure produced by a laboratory-scale, shock-free and unheated Mach 3 jet are used to support findings of the model. Acoustic waveforms are acquired on a two-dimensional grid extending out to 145 nozzle diameters from the jet exit plane. Various statistical metrics are employed to examine the degree of local and cumulative nonlinearity in the measured waveforms and their temporal derivatives. This includes a wave steepening factor (WSF), skewness, kurtosis and the normalized quadrature spectral density. The analysed data are shown to collapse reasonably well along rays emanating from the post-potential-core region of the jet. An application of the generalized Burgers equation is used to demonstrate the effect of cumulative nonlinear distortion on an arbitrary acoustic waveform produced by a high-convective-Mach-number supersonic jet. It is advocated that cumulative nonlinear distortion effects during far-field sound propagation are too subtle in this range-restricted environment and over the region covered, which may be true for other laboratory-scale jet noise facilities.
$\Gamma $ is restricted to plane wave applications. Scaling laws are then derived to account for the effects imposed by jet exit conditions of practical interest and includes Mach number, temperature ratio, Strouhal number and an absolute observer distance relative to a broadband Gaussian source. Surveys of the acoustic pressure produced by a laboratory-scale, shock-free and unheated Mach 3 jet are used to support findings of the model. Acoustic waveforms are acquired on a two-dimensional grid extending out to 145 nozzle diameters from the jet exit plane. Various statistical metrics are employed to examine the degree of local and cumulative nonlinearity in the measured waveforms and their temporal derivatives. This includes a wave steepening factor (WSF), skewness, kurtosis and the normalized quadrature spectral density. The analysed data are shown to collapse reasonably well along rays emanating from the post-potential-core region of the jet. An application of the generalized Burgers equation is used to demonstrate the effect of cumulative nonlinear distortion on an arbitrary acoustic waveform produced by a high-convective-Mach-number supersonic jet. It is advocated that cumulative nonlinear distortion effects during far-field sound propagation are too subtle in this range-restricted environment and over the region covered, which may be true for other laboratory-scale jet noise facilities.