Article contents
A numerical study of a weakly stratified turbulent wake
Published online by Cambridge University Press: 13 July 2015
Abstract
Direct numerical simulation (DNS) of a time-developing turbulent wake evolving in a stably stratified background is presented. A large initial Froude number is chosen to allow the wake to become fully turbulent and axisymmetric before stratification affects the spreading rate of the mean defect. Turbulence statistics are formed by averaging over the homogeneous streamwise direction of a domain that is larger than earlier stratified-wake simulations in order to reduce the statistical uncertainty. The DNS results are used to cast light on the mechanisms that lead to the various states of this flow – namely the three-dimensional (essentially unstratified), non-equilibrium (or ‘wake-collapse’) and quasi-two-dimensional (or ‘two-component’) regimes, previously observed for wakes embedded in both weakly and strongly stratified backgrounds. For this relatively high-initial-Reynolds- and Froude-number simulation, we find that the signature reduction in the rate of decay of the maximum mean defect velocity during the wake-collapse regime is due to buoyancy-induced alterations of the turbulence structure, which weaken and redistribute the Reynolds shear stresses whose gradients appear in the streamwise mean momentum equation. The change in the rate of decay of the turbulence kinetic energy (TKE) observed during the wake-collapse regime (which occurs well after the mean velocity decay reduction begins) is not caused by transfer of turbulent gravitational potential energy to TKE, as has been previously suggested. The results instead reveal that the reduction in TKE decay – which for this flow, with its relatively weak internal waves, eventually leads to TKE growth, heralding the arrival of the two-component regime – is caused by an increase in the rate of TKE production associated with the wake structure becoming increasingly two-dimensional, such that the lateral Reynolds shear stress, 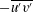 $-\overline{u^{\prime }v^{\prime }}$, becomes dominant. The present results are also compared with those of previous simulations at different Froude and Reynolds numbers, and whose initial conditions contain different turbulence structures. This comparison confirms a strong degree of commonality in the late-wake behaviour, which lends support to the hypothesis that all wakes in stably stratified environments achieve a universal state in the final stages of decay.
$-\overline{u^{\prime }v^{\prime }}$, becomes dominant. The present results are also compared with those of previous simulations at different Froude and Reynolds numbers, and whose initial conditions contain different turbulence structures. This comparison confirms a strong degree of commonality in the late-wake behaviour, which lends support to the hypothesis that all wakes in stably stratified environments achieve a universal state in the final stages of decay.
- Type
- Papers
- Information
- Copyright
- © 2015 Cambridge University Press
Footnotes
Present address: CMLA, ENS de Cachan, 61 avenue du président Wilson, 94235 Cachan, France.
References
- 37
- Cited by



