Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-22T14:32:14.063Z Has data issue: false hasContentIssue false
  • English
  • Français

Numerical stability analysis of a vortex ring with swirl

Published online by Cambridge University Press:  04 September 2019

Yuji Hattori Open the ORCID record for Yuji Hattori [Opens in a new window] ,
Francisco J. Blanco-Rodríguez  and
Stéphane Le Dizès Open the ORCID record for Stéphane Le Dizès [Opens in a new window]
Yuji Hattori*
Affiliation:
Institute of Fluid Science, Tohoku University, Sendai 980-8577, Japan
Francisco J. Blanco-Rodríguez
Affiliation:
Aix Marseille Université, CNRS, Centrale Marseille, IRPHE, UMR 7342, 13384 Marseille, France Área de Mecánica de fluidos, Departamento de Ingeniería Aeroespacial y Mecánica de fluidos, Universidad de Sevilla, Avda de los Descubrimientos, s/n, 41092 Sevilla, Spain
Stéphane Le Dizès
Affiliation:
Aix Marseille Université, CNRS, Centrale Marseille, IRPHE, UMR 7342, 13384 Marseille, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The linear instability of a vortex ring with swirl with Gaussian distributions of azimuthal vorticity and velocity in its core is studied by direct numerical simulation. The numerical study is carried out in two steps: first, an axisymmetric simulation of the Navier–Stokes equations is performed to obtain the quasi-steady state that forms a base flow; then, the equations are linearized around this base flow and integrated for a sufficiently long time to obtain the characteristics of the most unstable mode. It is shown that the vortex rings are subjected to curvature instability as predicted analytically by Blanco-Rodríguez & Le Dizès (J. Fluid Mech., vol. 814, 2017, pp. 397–415). Both the structure and the growth rate of the unstable modes obtained numerically are in good agreement with the analytical results. However, a small overestimation (e.g. 22 % for a curvature instability mode) by the theory of the numerical growth rate is found for some instability modes. This is most likely due to evaluation of the critical layer damping which is performed for the waves on axisymmetric line vortices in the analysis. The actual position of the critical layer is affected by deformation of the core due to the curvature effect; as a result, the damping rate changes since it is sensitive to the position of the critical layer. Competition between the curvature and elliptic instabilities is also investigated. Without swirl, only the elliptic instability is observed in agreement with previous numerical and experimental results. In the presence of swirl, sharp bands of both curvature and elliptic instabilities are obtained for $\unicode[STIX]{x1D700}=a/R=0.1$, where $a$ is the vortex core radius and 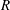$R$ the ring radius, while the elliptic instability dominates for 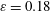$\unicode[STIX]{x1D700}=0.18$. New types of instability mode are also obtained: a special curvature mode composed of three waves is observed and spiral modes that do not seem to be related to any wave resonance. The curvature instability is also confirmed by direct numerical simulation of the full Navier–Stokes equations. Weakly nonlinear saturation and subsequent decay of the curvature instability are also observed.

JFM classification

Instability: Parametric instability Vortex Flows: Vortex instability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 878 , 10 November 2019 , pp. 5 - 36
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Blanco-Rodríguez, F.  J. & Le Dizès, S. 2016 Elliptic instability of a curved Batchelor vortex. J. Fluid Mech. 804, 224–247.Google Scholar
Blanco-Rodríguez, F.  J. & Le Dizès, S. 2017 Curvature instability of a curved Batchelor vortex. J. Fluid Mech. 814, 397–415.Google Scholar
Dazin, A., Dupont, P. & Stanislas, M. 2006a Experimental characterization of the instability of the vortex ring. Part I: Linear phase. Exp. Fluids 40, 383–399.Google Scholar
Dazin, A., Dupont, P. & Stanislas, M. 2006b Experimental characterization of the instability of the vortex rings. Part II: Non-linear phase. Exp. Fluids 41, 401–413.Google Scholar
Delbende, I., Rossi, M. & Daube, O. 2012 DNS of flows with helical symmetry. Theor. Comput. Fluid Dyn. 26, 141–160.Google Scholar
Eloy, C., Le Gal, P. & Le Dizès, S. 2000 Experimental study of the multipolar vortex instability. Phys. Rev. Lett. 85, 3000–3003.Google Scholar
Eloy, C., Le Gal, P. & Le Dizès, S. 2003 Elliptic and triangular instabilities in rotating cylinders. J. Fluid Mech. 476, 357–388.Google Scholar
Fabre, D., Sipp, D. & Jacquin, L. 2006 Kelvin waves and the singular modes of the Lamb-Oseen vortex. J. Fluid Mech. 551, 235–274.Google Scholar
Fukumoto, Y. & Hattori, Y. 2005 Curvature instability of a vortex ring. J. Fluid Mech. 526, 77–115.Google Scholar
Fukumoto, Y. & Moffatt, H. K. 2000 Motion and expansion of a viscous vortex ring. Part 1. A higher-order asymptotic formula for the velocity. J. Fluid Mech. 417, 1–45.Google Scholar
Gan, L., Nickels, T. B. & Dawson, J. R. 2011 An experimental study of a turbulent vortex ring: a three-dimensional representation. Exp. Fluids 51, 1493–1507.Google Scholar
Gargan-Shingles, C., Rudman, M. & Ryan, K. 2016 The linear stability of swirling vortex rings. Phys. Fluids 28, 114106.Google Scholar
Hattori, Y. & Fukumoto, Y. 2003 Short-wavelength stability analysis of thin vortex rings. Phys. Fluids 15, 3151–3163.Google Scholar
Hattori, Y. & Fukumoto, Y. 2009 Short-wavelength stability analysis of a helical vortex tube. Phys. Fluids 21, 014104.Google Scholar
Hattori, Y. & Fukumoto, Y. 2010 Short-wave stability of a helical vortex tube: the effect of torsion on the curvature instability. Theor. Comput. Fluid Dyn. 24, 363–368.Google Scholar
Hattori, Y. & Fukumoto, Y. 2011 Erratum: short-wavelength stability analysis of a helical vortex tube. Phys. Fluids 23, 049901.Google Scholar
Hattori, Y. & Fukumoto, Y. 2012 Effects of axial flow on the stability of a helical vortex tube. Phys. Fluids 24, 054102.Google Scholar
Hattori, Y. & Fukumoto, Y. 2014 Modal stability analysis of a helical vortex tube with axial flow. J. Fluid Mech. 738, 222–249.Google Scholar
Hattori, Y. & Hijiya, K. 2010 Short-wavelength stability analysis of Hill’s vortex with/without swirl. Phys. Fluids 22, 074104.Google Scholar
Kerswell, R. 1993 The instability of precessing flow. Geophys. Astrophys. Fluid Dyn. 72, 107–144.Google Scholar
Knobloch, E., Mahalov, A. & Marsden, J. E. 1994 Normal forms for three-dimensional parametric instabilities in ideal hydrodynamics. Physica D 73, 49–81.Google Scholar
Lacaze, L., Ryan, K. & Le Dizès, S. 2007 Elliptic instability in a strained Batchelor vortex. J. Fluid Mech. 577, 341–361.Google Scholar
Lagrange, R., Eloy, C., Nadal, F. & Meunier, P. 2008 Instability of a fluid inside a precessing cylinder. Phys. Fluids 20, 081701.Google Scholar
Le Dizès, S. 2004 Viscous critical-layer analysis of vortex normal modes. Stud. Appl. Maths 112, 315–332.Google Scholar
Lele, S. K. 1992 Compact finite-difference schemes with spectral-like resolution. J. Comput. Phys. 103, 16–42.Google Scholar
Mahalov, A. 1993 The instability of rotating fluid columns subjected to a weak external Coriolis force. Phys. Fluids A 5, 891–900.Google Scholar
Mao, X. & Hussain, F. 2017 Optimal transient growth on a vortex ring and its transition via cascade of ringlets. J. Fluid Mech. 832, 269–286.Google Scholar
Maxworthy, T. 1972 The structure and stability of vortex rings. J. Fluid Mech. 51, 15–32.Google Scholar
Maxworthy, T. 1977 Some experimental studies of vortex rings. J. Fluid Mech. 81, 465–495.Google Scholar
Moore, D. W. & Saffman, P. G. 1975 The instability of a straight vortex filament in a strain field. Proc. R. Soc. Lond. A 346, 413–425.Google Scholar
Roy, C., Schaeffer, N., Le Dizès, S. & Thompson, M. 2008 Phys. Fluids 20, 094101.Google Scholar
Saffman, P. G. 1992 Vortex Dynamics, chap. 2, 10, Cambridge University Press.Google Scholar
Selçuk, C., Delbende, I. & Rossi, M. 2018 Helical vortices: linear stability analysis and nonlinear dynamics. Fluid Dyn. Res. 50, 011411.Google Scholar
Shariff, K., Verzicco, R. & Orlandi, P. 1994 A numerical study of three-dimensional vortex ring instabilities: viscous corrections and early nonlinear stage. J. Fluid Mech. 279, 351–375.Google Scholar
Sipp, D. 2000 Weakly nonlinear saturation of short-wave instabilities in a strained Lamb-Oseen vortex. Phys. Fluids 12, 1715–1729.Google Scholar
Thompson, M. C., Leweke, T. & Hourigan, K. 2007 Spherewall collisions: vortex dynamics and stability. J. Fluid Mech. 575, 121–148.Google Scholar
Widnall, S. E. & Tsai, C.-Y. 1976 The stability of short waves on a straight vortex filament in a weak externally imposed strain field. J. Fluid Mech. 73, 721–733.Google Scholar
Widnall, S. E., Bliss, D. B. & Zalay, Y. 1971 Theoretical and experimental study of the stability of a vortex pair. In Aircraft Wake Turbulence and Its Detection (ed. Olsen, J. H., Goldburg, A. & Rogers, M.), pp. 305–338. Springer.Google Scholar
Widnall, S. E. & Sullivan, J. P. 1973 On the stability of vortex rings. Proc. R. Soc. Lond. A 332, 335–353.Google Scholar
Widnall, S. E. & Tsai, C.-Y. 1977 The instability of the thin vortex ring of constant vorticity. Phil. Trans. R. Soc. Lond. A 287, 273–305.Google Scholar