Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Krajnovic, Prof., Sinisa
Barros, D.
Ruiz, T.
Borée, J.
and
Noack, B.
2014.
Control of a three-dimensional blunt body wake using low and high frequency pulsed jets.
International Journal of Flow Control,
Vol. 6,
Issue. 1,
p.
61.
Parkin, D.
Sheridan, J.
and
Thompson, M.C.
2015.
Numerical analysis of periodic open-loop flow control on bluff bodies in ground proximity.
Journal of Wind Engineering and Industrial Aerodynamics,
Vol. 145,
Issue. ,
p.
339.
Gao, Nan
Li, Yaqing
and
Bai, Honglei
2015.
Measuring Large Scale Flow Structures Behind a Bluff Body Using a Hot-wire Rake.
Procedia Engineering,
Vol. 126,
Issue. ,
p.
532.
Gao, N.
Li, Y. Q.
Bai, H. L.
and
Wu, C. J.
2016.
Effects of Synthetic jets on a D-Shaped Cylinder wake at a Subcritical Reynolds Number.
Flow, Turbulence and Combustion,
Vol. 97,
Issue. 3,
p.
729.
Wang, Chenglei
Tang, Hui
Duan, Fei
and
Yu, Simon C.M.
2016.
Control of wakes and vortex-induced vibrations of a single circular cylinder using synthetic jets.
Journal of Fluids and Structures,
Vol. 60,
Issue. ,
p.
160.
McArthur, Damien
Burton, David
Thompson, Mark
and
Sheridan, John
2016.
On the near wake of a simplified heavy vehicle.
Journal of Fluids and Structures,
Vol. 66,
Issue. ,
p.
293.
Minelli, G.
Krajnović, S.
Basara, B.
and
Noack, B. R.
2016.
Numerical Investigation of Active Flow Control Around a Generic Truck A-Pillar.
Flow, Turbulence and Combustion,
Vol. 97,
Issue. 4,
p.
1235.
Minelli, Guglielmo
and
Krajnović, Siniša
2016.
Proceedings of the 5th International Conference on Jets, Wakes and Separated Flows (ICJWSF2015).
Vol. 185,
Issue. ,
p.
295.
Lorite-Díez, M.
Jiménez-González, J.I.
Gutiérrez-Montes, C.
and
Martínez-Bazán, C.
2017.
Drag reduction of slender blunt-based bodies using optimized rear cavities.
Journal of Fluids and Structures,
Vol. 74,
Issue. ,
p.
158.
Dellar, O. J.
and
Jones, B. Ll
2017.
Dynamically correct formulations of the linearised Navier–Stokes equations.
International Journal for Numerical Methods in Fluids,
Vol. 85,
Issue. 1,
p.
3.
Yao, W.
and
Jaiman, R. K.
2017.
Feedback control of unstable flow and vortex-induced vibration using the eigensystem realization algorithm.
Journal of Fluid Mechanics,
Vol. 827,
Issue. ,
p.
394.
Hemmati, Arman
Wood, David H.
and
Martinuzzi, Robert J.
2018.
On simulating the flow past a normal thin flat plate.
Journal of Wind Engineering and Industrial Aerodynamics,
Vol. 174,
Issue. ,
p.
170.
Edwige, Stephie
Eulalie, Yoann
Gilotte, Philippe
and
Mortazavi, Iraj
2018.
Wake flow analysis and control on a 47° slant angle Ahmed body.
International Journal of Numerical Methods for Heat & Fluid Flow,
Vol. 28,
Issue. 5,
p.
1061.
Brandner, Paul A.
Venning, James A.
and
Pearce, Bryce W.
2018.
Wavelet analysis techniques in cavitating flows.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 376,
Issue. 2126,
p.
20170242.
Rao, Anirudh N.
Zhang, Jie
Minelli, Guglielmo
Basara, Branislav
and
Krajnović, Siniša
2019.
An LES Investigation of the Near-Wake Flow Topology of a Simplified Heavy Vehicle.
Flow, Turbulence and Combustion,
Vol. 102,
Issue. 2,
p.
389.
Minelli, G.
Dong, T.
Noack, B. R.
and
Krajnović, S.
2020.
Upstream actuation for bluff-body wake control driven by a genetically inspired optimization.
Journal of Fluid Mechanics,
Vol. 893,
Issue. ,
Easanesan, Gershom
Burton, David
and
Thompson, Mark C.
2020.
The effects of nose-shape and upstream flow separation on the wake of a cylindrical square-backed body.
Experimental Thermal and Fluid Science,
Vol. 118,
Issue. ,
p.
110142.
Zhu, Taihang
and
Morrison, Jonathan F.
2021.
Simulation of the turbulent axisymmetric bluff body wake with pulsed jet forcing.
Physical Review Fluids,
Vol. 6,
Issue. 12,
Cheng, Yuwei
and
Chen, Qian
2021.
Large Eddy Simulation and Dynamic Mode Decomposition of Turbulent Mixing Layers.
Applied Sciences,
Vol. 11,
Issue. 24,
p.
12127.
Bao, Di
Borée, Jacques
Haffner, Yann
and
Sicot, Christophe
2022.
Near wake interactions and drag increase regimes for a square-back bluff body.
Journal of Fluid Mechanics,
Vol. 936,
Issue. ,
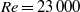 $Re= 23\hspace{0.167em} 000$ are used to investigate the drag on a two-dimensional elongated cylinder caused by rear-edge periodic actuation, with particular focus on an optimum open-loop configuration. The 3.64 (length/thickness) aspect-ratio cylinder has a rectangular cross-section with rounded leading corners, representing the two-dimensional cross-section of the now generic Ahmed-body geometry. The simulations show that the optimum drag reduction occurs in the forcing Strouhal number range of
$Re= 23\hspace{0.167em} 000$ are used to investigate the drag on a two-dimensional elongated cylinder caused by rear-edge periodic actuation, with particular focus on an optimum open-loop configuration. The 3.64 (length/thickness) aspect-ratio cylinder has a rectangular cross-section with rounded leading corners, representing the two-dimensional cross-section of the now generic Ahmed-body geometry. The simulations show that the optimum drag reduction occurs in the forcing Strouhal number range of 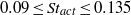 $0. 09\leq S{t}_{act} \leq 0. 135$, which is approximately half of the Strouhal number corresponding to shedding of von Kármán vortices into the wake for the natural case. This result agrees well with recent experiments of Henning et al. (Active Flow Control, vol. 95, 2007, pp. 369–390). A thorough transient wake analysis employing dynamic mode decomposition is conducted for all cases, with special attention paid to the Koopman modes of the wake flow and vortex progression downstream. Two modes are found to coexist in all cases, the superimposition of which recovers the majority of features observed in the flow. Symmetric vortex shedding in the near wake, which effectively extends the mean recirculation bubble, is shown to be the major mechanism in lowering the drag. This is associated with opposite-signed vortices reducing the influence of natural vortex shedding, resulting in an increase in the pressure in the near wake, while the characteristic wake antisymmetry returns further downstream. Lower-frequency actuation is shown to create larger near-wake symmetric vortices, which improves the effectiveness of this process.
$0. 09\leq S{t}_{act} \leq 0. 135$, which is approximately half of the Strouhal number corresponding to shedding of von Kármán vortices into the wake for the natural case. This result agrees well with recent experiments of Henning et al. (Active Flow Control, vol. 95, 2007, pp. 369–390). A thorough transient wake analysis employing dynamic mode decomposition is conducted for all cases, with special attention paid to the Koopman modes of the wake flow and vortex progression downstream. Two modes are found to coexist in all cases, the superimposition of which recovers the majority of features observed in the flow. Symmetric vortex shedding in the near wake, which effectively extends the mean recirculation bubble, is shown to be the major mechanism in lowering the drag. This is associated with opposite-signed vortices reducing the influence of natural vortex shedding, resulting in an increase in the pressure in the near wake, while the characteristic wake antisymmetry returns further downstream. Lower-frequency actuation is shown to create larger near-wake symmetric vortices, which improves the effectiveness of this process.

