Published online by Cambridge University Press: 05 September 2011
We study both theoretically and numerically surface quasi-geostrophic turbulence regularized by the usual molecular viscosity, with an emphasis on a number of classical predictions. It is found that the system’s number of degrees of freedom  , which is defined in terms of local Lyapunov exponents, scales as
, which is defined in terms of local Lyapunov exponents, scales as 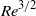 , where
, where 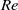 is the Reynolds number expressible in terms of the viscosity, energy dissipation rate and system’s integral scale. For general power-law energy spectra
is the Reynolds number expressible in terms of the viscosity, energy dissipation rate and system’s integral scale. For general power-law energy spectra 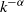 , a comparison of
, a comparison of 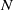 with the number of dynamically active Fourier modes, i.e. the modes within the energy inertial range, yields
with the number of dynamically active Fourier modes, i.e. the modes within the energy inertial range, yields 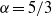 . This comparison further renders the scaling
. This comparison further renders the scaling 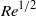 for the exponential dissipation rate at the dissipation wavenumber. These results have been predicted on the basis of Kolmogorov’s theory. Our approach thus recovers these classical predictions and is an analytic alternative to the traditional phenomenological method. The implications of the present findings are discussed in conjunction with related results in the literature. Support for the analytic results is provided through a series of direct numerical simulations.
for the exponential dissipation rate at the dissipation wavenumber. These results have been predicted on the basis of Kolmogorov’s theory. Our approach thus recovers these classical predictions and is an analytic alternative to the traditional phenomenological method. The implications of the present findings are discussed in conjunction with related results in the literature. Support for the analytic results is provided through a series of direct numerical simulations.