Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Fernandez-Feria, R.
2017.
Note on optimum propulsion of heaving and pitching airfoils from linear potential theory.
Journal of Fluid Mechanics,
Vol. 826,
Issue. ,
p.
781.
Strangfeld, Christoph
Nayeri, Christian N.
Paschereit, Christian O.
and
Greenblatt, David
2018.
Mechanism of Vortex Perturbation via Unsteady Pitching.
Journal of Aircraft,
Vol. 55,
Issue. 5,
p.
1831.
Li, Mingshui
Yang, Yang
Li, Ming
and
Liao, Haili
2018.
Direct measurement of the Sears function in turbulent flow.
Journal of Fluid Mechanics,
Vol. 847,
Issue. ,
p.
768.
Zhong, Yingzi
Li, Mingshui
and
Ma, Cunming
2018.
Aerodynamic admittance of truss girder in homogenous turbulence.
Journal of Wind Engineering and Industrial Aerodynamics,
Vol. 172,
Issue. ,
p.
152.
Fernandez-Feria, R.
and
Alaminos-Quesada, J.
2018.
Unsteady thrust, lift and moment of a two-dimensional flapping thin airfoil in the presence of leading-edge vortices: a first approximation from linear potential theory.
Journal of Fluid Mechanics,
Vol. 851,
Issue. ,
p.
344.
Wei, Nathaniel J.
Kissing, Johannes
Wester, Tom T. B.
Wegt, Sebastian
Schiffmann, Klaus
Jakirlic, Suad
Hölling, Michael
Peinke, Joachim
and
Tropea, Cameron
2019.
Insights into the periodic gust response of airfoils.
Journal of Fluid Mechanics,
Vol. 876,
Issue. ,
p.
237.
Wei, Nathaniel J.
Kissing, Johannes
and
Tropea, Cameron
2019.
Generation of periodic gusts with a pitching and plunging airfoil.
Experiments in Fluids,
Vol. 60,
Issue. 11,
Baddoo, Peter J.
2020.
Lightning Solvers for Potential Flows.
Fluids,
Vol. 5,
Issue. 4,
p.
227.
Young, Anna M.
and
Smyth, Amanda S.
2020.
The interaction of a Sears-type sinusoidal gust with a cambered aerofoil in the presence of non-uniform streamwise flow.
Wu, Zhenlong
Bangga, Galih
Lutz, Thorsten
Kampers, Gerrit
and
Hölling, Michael
2020.
Insights into airfoil response to sinusoidal gusty inflow by oscillating vanes.
Physics of Fluids,
Vol. 32,
Issue. 12,
Ma, Li
Yao, Minghui
Zhang, Wei
Lou, Kai
Cao, Dongxing
and
Niu, Yan
2020.
A Novel Aerodynamic Force and Flutter of the High-Aspect-Ratio Cantilever Plate in Subsonic Flow.
Shock and Vibration,
Vol. 2020,
Issue. ,
p.
1.
Zheng, Xiaobo
Pröbsting, Stefan
Wang, Hongliang
and
Li, Ye
2021.
Characteristics of vortex shedding from a sinusoidally pitching hydrofoil at high Reynolds number.
Physical Review Fluids,
Vol. 6,
Issue. 8,
Ōtomo, Shūji
Henne, Sabrina
Mulleners, Karen
Ramesh, Kiran
and
Viola, Ignazio Maria
2021.
Unsteady lift on a high-amplitude pitching aerofoil.
Experiments in Fluids,
Vol. 62,
Issue. 1,
Li, Ming
Li, Qiusheng
and
Shi, Haoyun
2021.
Effect of sinusoidal vertical gust on the pressure distributions on and flow structures around a rectangular cylinder.
Experiments in Fluids,
Vol. 62,
Issue. 7,
Li, Weilin
Patruno, Luca
Niu, Huawei
de Miranda, Stefano
and
Hua, Xugang
2021.
Identification of complex admittance functions using 2D-URANS models: Inflow generation and validation on rectangular cylinders.
Journal of Wind Engineering and Industrial Aerodynamics,
Vol. 208,
Issue. ,
p.
104435.
Natale, Nunzio
Russo, Serena
Dequand, Sylvie
Lepage, Arnaud
and
Paletta, Nicola
2021.
Aerodynamic Design of Airfoil Shape for Gust Generation in a Transonic Wind Tunnel.
Aerotecnica Missili & Spazio,
Vol. 100,
Issue. 4,
p.
345.
Benjamin, Tan Kin Jon
Hesse, Henrik
and
Wang, Peng Cheng
2021.
Wing-Tail Interaction Under Forced Harmonic Pitch.
Baddoo, Peter J.
Hajian, Rozhin
and
Jaworski, Justin W.
2021.
Unsteady aerodynamics of porous aerofoils.
Journal of Fluid Mechanics,
Vol. 913,
Issue. ,
Young, Anna M.
and
Smyth, Amanda S. M.
2021.
Gust–Airfoil Coupling with a Loaded Airfoil.
AIAA Journal,
Vol. 59,
Issue. 3,
p.
773.
Turhan, Burak
Wang, Zhijin
and
Gursul, Ismet
2022.
Coherence of unsteady wake of periodically plunging airfoil.
Journal of Fluid Mechanics,
Vol. 938,
Issue. ,
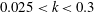 $0.025<k<0.3$ and the Reynolds number of the undisturbed flow is in the range of
$0.025<k<0.3$ and the Reynolds number of the undisturbed flow is in the range of  $120\,000<\mathit{Re}<300\,000$. While the Theodorsen function is found to be a good estimator for the unsteady lift at moderate mean angles of attack, the Sears function does not capture the experimental transfer functions in frequency dependence or in limiting values. A second-order model provided by Atassi (J. Fluid Mech., vol. 141, 1984, pp. 109–122) agrees well with the experimental transfer function.
$120\,000<\mathit{Re}<300\,000$. While the Theodorsen function is found to be a good estimator for the unsteady lift at moderate mean angles of attack, the Sears function does not capture the experimental transfer functions in frequency dependence or in limiting values. A second-order model provided by Atassi (J. Fluid Mech., vol. 141, 1984, pp. 109–122) agrees well with the experimental transfer function.


