Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-20T00:11:53.279Z Has data issue: false hasContentIssue false
  • English
  • Français

Nonlinear dynamics of forced baroclinic critical layers

Published online by Cambridge University Press:  25 November 2019

Chen Wang Open the ORCID record for Chen Wang [Opens in a new window]  and
Neil J. Balmforth Open the ORCID record for Neil J. Balmforth [Opens in a new window]
Chen Wang*
Affiliation:
Department of Mathematics, University of British Columbia, Vancouver, BC V6T 1Z2, Canada
Neil J. Balmforth
Affiliation:
Department of Mathematics, University of British Columbia, Vancouver, BC V6T 1Z2, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

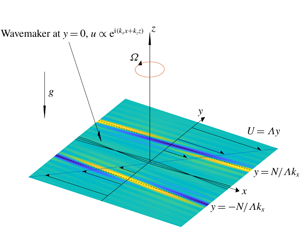
In this paper, we study the forcing of baroclinic critical levels, which arise in stratified fluids with horizontal shear flow along the surfaces where the phase speed of a wave relative to the mean flow matches a natural internal wave speed. Linear theory predicts the baroclinic critical-layer dynamics is similar to that of a classical critical layer, characterized by the secular growth of flow perturbations over a region of decreasing width. By using matched asymptotic expansions, we construct a nonlinear baroclinic critical layer theory to study how the flow perturbations evolve once they enter the nonlinear regime. A key feature of the theory is that, because the location of the baroclinic critical layer is determined by the streamwise wavenumber, the nonlinear dynamics filters out harmonics and the modification to the mean flow controls the evolution. At late times, we show that the vorticity begins to focus into yet smaller regions whose width decreases exponentially with time, and that the addition of dissipative effects can arrest this focussing to create a drifting coherent structure. Jet-like defects in the mean horizontal velocity are the main outcome of the critical-layer dynamics.

JFM classification

Waves/Free-surface Flows: Critical layers Geophysical and Geological Flows: Stratified flows Geophysical and Geological Flows: Internal waves
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 883 , 25 January 2020 , A12
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Badulin, S. I., Shrira, V. I. & Tsimring, L. S. 1985 The trapping and vertical focusing of internal waves in a pycnocline due to the horizontal inhomogeneities of density and currents. J. Fluid Mech. 158, 199–218.CrossRefGoogle Scholar
Balmforth, N. J. & Korycansky, D. G. 2001 Non-linear dynamics of the corotation torque. Mon. Not. R. Astron. Soc. 326, 833–851.CrossRefGoogle Scholar
Barranco, J. A., Pei, S. & Marcus, P. S. 2018 Zombie vortex instability. III. Persistence with nonuniform stratification and radiative damping. Astrophys. J. 869, 127.CrossRefGoogle Scholar
Basovich, A. Y. & Tsimring, L. S. 1984 Internal waves in a horizontally inhomogeneous flow. J. Fluid Mech. 142, 233–249.CrossRefGoogle Scholar
Béland, M. 1976 Numerical study of the nonlinear Rossby wave critical level development in a barotropic zonal flow. J. Atmos. Sci. 33, 2066–2078.2.0.CO;2>CrossRefGoogle Scholar
Booker, J. R. & Bretherton, F. P. 1967 The critical layer for internal gravity waves in a shear flow. J. Fluid Mech. 27, 513–539.CrossRefGoogle Scholar
Boulanger, N., Meunier, P. & Le Dizès, S. 2007 Structure of a stratified tilted vortex. J. Fluid Mech. 583, 443–458.CrossRefGoogle Scholar
Bretherton, F. P. 1966 The propagation of groups of internal gravity waves in a shear flow. Q. J. R. Meteorol. Soc. 92, 466–480.CrossRefGoogle Scholar
Brown, S. N. & Stewartson, K. 1978 The evolution of the critical layer of a Rossby wave. Part II. Geophys. Astrophys. Fluid Dyn. 10, 1–24.CrossRefGoogle Scholar
Brown, S. N. & Stewartson, K. 1980 On the nonlinear reflexion of a gravity wave at a critical level. Part 1. J. Fluid Mech. 100, 577–595.CrossRefGoogle Scholar
Brown, S. N. & Stewartson, K. 1982a On the nonlinear reflection of a gravity wave at a critical level. Part 2. J. Fluid Mech. 115, 217–230.CrossRefGoogle Scholar
Brown, S. N. & Stewartson, K. 1982b On the nonlinear reflection of a gravity wave at a critical level. Part 3. J. Fluid Mech. 115, 231–250.CrossRefGoogle Scholar
Bühler, O. 2014 Waves and Mean Flows. Cambridge University Press.CrossRefGoogle Scholar
Edwards, N. R. & Staquet, C. 2005 Focusing of an inertia-gravity wave packet by a baroclinic shear flow. Dyn. Atmos. Oceans 40, 91–113.CrossRefGoogle Scholar
Emanuel, K. A. 1994 Atmospheric Convection. Cambridge University Press.Google Scholar
Haynes, P. H. 1989 The effect of barotropic instability on the nonlinear evolution of a Rossby-wave critical layer. J. Fluid Mech. 207, 231–266.CrossRefGoogle Scholar
Killworth, P. D. & McIntyre, M. E. 1985 Do Rossby-wave critical layers absorb, reflect, or over-reflect? J. Fluid Mech. 161, 449–492.CrossRefGoogle Scholar
Marcus, P. S., Pei, S., Jiang, C.-H. & Hassanzadeh, P. 2013 Three-dimensional vortices generated by self-replication in stably stratified rotating shear flows. Phys. Rev. Lett. 111, 084501.CrossRefGoogle ScholarPubMed
Marcus, P. S., Pei, S., Jiang, C.-H. & Barranco, J. A. 2015 Zombie vortex instability. I. A purely hydrodynamic instability to resurrect the dead zones of protoplanetary disks. Astrophys. J. 808, 87.CrossRefGoogle Scholar
Marcus, P. S., Pei, S., Jiang, C.-H. & Barranco, J. A. 2016 Zombie vortex instability. II. Thresholds to trigger instability and the properties of zombie turbulence in the dead zones of protoplanetary disks. Astrophys. J. 883, 2.Google Scholar
Maslowe, S. A. 1986 Crtical layers in shear flows. Ann. Rev. Fluid Mech. 18, 405–432.CrossRefGoogle Scholar
Olbers, D. J. 1981 The propagation of internal waves in a geostrophic current. J. Phys. Oceanogr. 11, 1224–1233.2.0.CO;2>CrossRefGoogle Scholar
Staquet, C. & Huerre, G. 2002 On transport across a barotropic shear flow by breaking inertia-gravity waves. Phys. Fluids 14, 1993–2006.CrossRefGoogle Scholar
Stewartson, K. 1978 The evolution of the critical layer of a Rossby wave. Geophys. Astrophys. Fluid Dyn. 9, 185–200.CrossRefGoogle Scholar
Vanneste, J. & Yavneh, I. 2007 Unbalanced instabilities of rapidly rotating stratified shear flows. J. Fluid Mech. 584, 373–396.CrossRefGoogle Scholar
Wang, C. & Balmforth, N. J. 2018 Strato-rotational instability without resonance. J. Fluid Mech. 846, 815–833.CrossRefGoogle Scholar
Wang, M.2016 Baroclinic critical layers and zombie vortex instability in stratified rotational shear flow. PhD thesis, University of California, Berkeley.Google Scholar
Warn, T. & Warn, H. 1978 The evolution of a nonlinear critical level. Stud. Appl. Maths 59, 37–71.CrossRefGoogle Scholar
Warn, T. & Warn, H. 1976 On the development of a Rossby wave critical level. J. Atmos. Sci. 33, 2021–2024.2.0.CO;2>CrossRefGoogle Scholar
Yavneh, I., McWilliams, J. C. & Molemaker, M. J. 2001 Non-axisymmetric instability of centrifugally stable stratified Taylor–Couette flow. J. Fluid Mech. 448, 1–21.CrossRefGoogle Scholar