Article contents
Natural convection in vertical enclosures with conjugate boundary conditions
Published online by Cambridge University Press: 03 August 2022
Abstract
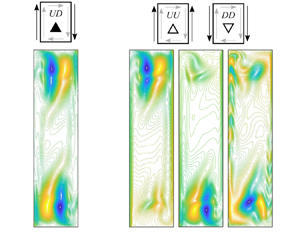
A numerical investigation and reduced-order modelling of natural convection in a cavity with differentially heated sidewalls is discussed. The effect of conjugate boundary conditions on the cavity's heat transfer and natural flow circulation with varying aspect ratios and Rayleigh numbers is examined. Validation of the canonical differentially heated cavity reveals that a modification to the definition of the Rayleigh number (Ra) is required to reconcile the  $Nu\sim Ra^{1/4}$ scaling (where Nu is the Nusselt number) and validity of previously proposed correlations for the heat transfer in vertical enclosures. Dynamic mode decomposition is used to uncover the underlying time-dependent flow structures and the results are compared with stability bifurcation studies in the literature. A flow mode previously associated with smaller aspect ratio cavities is identified as the unstable mode for a larger aspect ratio of 4. The effect of conjugate boundary conditions is scaled based on the ratio of the internal and external boundary layers, wherein higher external Reynolds numbers aid in the heat transfer as the Nusselt number approaches the isothermal limit. A reduced-order theoretical model is proposed to predict the Nusselt number for the conjugate boundary conditions. The performance of conjugate boundary conditions is connected to the flow stability, with the parallel-flow configuration acting in a destabilizing manner while the counterflow configuration has a stabilizing effect and results in the highest heat transfer. We also found that relatively large heat transfer can be achieved with substantially lower external actuation with intermediate aspect ratios, translating to less power in practice.
$Nu\sim Ra^{1/4}$ scaling (where Nu is the Nusselt number) and validity of previously proposed correlations for the heat transfer in vertical enclosures. Dynamic mode decomposition is used to uncover the underlying time-dependent flow structures and the results are compared with stability bifurcation studies in the literature. A flow mode previously associated with smaller aspect ratio cavities is identified as the unstable mode for a larger aspect ratio of 4. The effect of conjugate boundary conditions is scaled based on the ratio of the internal and external boundary layers, wherein higher external Reynolds numbers aid in the heat transfer as the Nusselt number approaches the isothermal limit. A reduced-order theoretical model is proposed to predict the Nusselt number for the conjugate boundary conditions. The performance of conjugate boundary conditions is connected to the flow stability, with the parallel-flow configuration acting in a destabilizing manner while the counterflow configuration has a stabilizing effect and results in the highest heat transfer. We also found that relatively large heat transfer can be achieved with substantially lower external actuation with intermediate aspect ratios, translating to less power in practice.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
References
REFERENCES
- 4
- Cited by



