Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-17T16:54:36.623Z Has data issue: false hasContentIssue false
  • English
  • Français

Morphologies and dynamics of micro-droplet impact onto an idealised scratch

Published online by Cambridge University Press:  26 August 2021

Khaled H.A. Al-Ghaithi Open the ORCID record for Khaled H.A. Al-Ghaithi [Opens in a new window] ,
Oliver G. Harlen Open the ORCID record for Oliver G. Harlen [Opens in a new window] ,
Nikil Kapur  and
Mark C.T. Wilson Open the ORCID record for Mark C.T. Wilson [Opens in a new window]
Khaled H.A. Al-Ghaithi
Affiliation:
EPSRC Centre for Doctoral Training in Fluid Dynamics, University of Leeds, Leeds LS2 9JT, UK
Oliver G. Harlen
Affiliation:
School of Mathematics, University of Leeds, Leeds LS2 9JT, UK
Nikil Kapur
Affiliation:
School of Mechanical Engineering, University of Leeds, Leeds LS2 9JT, UK
Mark C.T. Wilson*
Affiliation:
School of Mechanical Engineering, University of Leeds, Leeds LS2 9JT, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

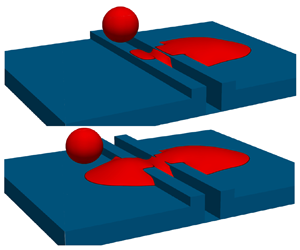
As inkjet technology develops to produce smaller droplets, substrate features such as accidental scratches or manufacturing defects can potentially affect the outcome of printing, particularly for printed electronics where continuous tracks are required. Here, the deposition of micro-droplets onto a scratch of commensurate size is studied. The scratch is considered as a groove of rectangular cross-section, with rectangular side ridges representing material displaced from the substrate, and seven equilibrium morphologies are identified as a result of inertial spreading, contact-line pinning, imbibition into the scratch and capillary flow. A regime map is constructed in terms of scratch depth and width, and theoretical estimates of the regime boundaries are developed by adapting droplet spreading laws for flat surfaces to account for liquid entering the scratches. Good agreement is seen with numerical results obtained using a graphical processing unit-accelerated three-dimensional multiphase lattice Boltzmann model validated against published experiments, and the influences of Reynolds number, Weber number and advancing and receding contact angles are explored. Negative and positive implications of the results for printing applications are discussed and illustrated via multiple-droplet simulations of printing across and along scratches.

JFM classification

Drops and Bubbles: Breakup/coalescence Drops and Bubbles: Drops Interfacial Flows (free surface): Capillary flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 925 , 25 October 2021 , A23
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ashoke Raman, K., Jaiman, R.K., Lee, T.S. & Low, H.T. 2017 Dynamics of simultaneously impinging drops on a dry surface: role of impact velocity and air inertia. J. Colloid Interface Sci. 516, 232–247.CrossRefGoogle Scholar
Bell, J.M. & Cameron, F.K. 1906 The flow of liquids through capillary spaces. J. Phys. Chem. 10 (8), 658–674.CrossRefGoogle Scholar
Bennett, T. & Poulikakos, D. 1993 Splat-quench solidification: estimating the maximum spreading of a droplet impacting a solid surface. J. Mater. Sci. 28, 963–970.CrossRefGoogle Scholar
Brostow, W., Deborde, J.-L., Jaklewicz, M. & Olszynski, P. 2003 Tribology with emphasis on polymers: friction, scratch and wear resistance. J. Mater. Educ. 25 (4–6), 119–132.Google Scholar
Bussmann, M., Mostaghimi, J. & Chandra, S. 1999 On a three-dimensional volume tracking model of droplet impact. Phys. Fluids 11 (6), 1406–1417.CrossRefGoogle Scholar
Castrejón-Pita, J.R., Kubiak, K.J., Castrejón-Pita, A.A., Wilson, M.C.T. & Hutchings, I.M. 2013 Mixing and internal dynamics of droplets impacting and coalescing on a solid surface. Phys. Rev. E 88 (2), 023023.CrossRefGoogle ScholarPubMed
Chandra, S. & Avedisian, C.T. 1991 On the collision of a droplet with a solid surface. Proc. R. Soc. Lond. A 432, 13–41.Google Scholar
Chen, Z., Wu, L.Y.L., Chwa, E. & Tham, O. 2008 Scratch resistance of brittle thin films on compliant substrates. Mater. Sci. Engng 493 (1–2), 292–298.CrossRefGoogle Scholar
Chilton, N. 2012 Printed circuit board fabrication. In Inkjet Technology for Digital Fabrication (ed. I.M. Hutchings & G.D. Martin), chap. 8, pp. 183–206. John Wiley & Sons, Ltd.Google Scholar
Cimpeanu, R. & Papageorgiou, D.T. 2018 Three-dimensional high speed drop impact onto solid surfaces at arbitrary angles. Intl J. Multiphase Flow 107, 192–207.CrossRefGoogle Scholar
Clanet, C., Béguin, C., Richard, D. & Quéré, D. 2004 Maximal deformation of an impacting drop. J. Fluid Mech. 517, 199–208.CrossRefGoogle Scholar
Connington, K. & Lee, T. 2013 Lattice Boltzmann simulations of forced wetting transitions of drops on superhydrophobic surfaces. J. Comput. Phys. 250, 601–615.CrossRefGoogle Scholar
Dasari, A., Yu, Z.Z. & Mai, Y.W. 2009 Fundamental aspects and recent progress on wear/scratch damage in polymer nanocomposites. Mater. Sci. Engng 63 (2), 31–80.CrossRefGoogle Scholar
Davis, S.H. 1980 Moving contact lines and rivulet instabilities. Part 1. The static rivulet. J. Fluid Mech. 98 (2), 225–242.CrossRefGoogle Scholar
De Jong, R., Enríquez, O.R. & Van der Meer, D. 2015 Exploring droplet impact near a millimetre-sized hole: comparing a closed pit with an open-ended pore. J. Fluid Mech. 772, 427–444.CrossRefGoogle Scholar
D'Humières, D., Ginzburg, I., Krafczyk, M., Lallemand, P. & Luo, L.S. 2002 Multiple-relaxation-time lattice Boltzmann models in three dimensions. Phil. Trans. R. Soc. Lond. A 360, 437–451.Google Scholar
Ding, H. & Spelt, P.D.M. 2007 Wetting condition in diffuse interface simulations of contact line motion. Phys. Rev. E 75 (4), 046708.CrossRefGoogle ScholarPubMed
Ding, S., Liu, X., Wu, X. & Zhang, X. 2020 Droplet breakup and rebound during impact on small cylindrical superhydrophobic targets. Phys. Fluids 32 (10), 102106.CrossRefGoogle Scholar
Dong, H., Carr, W.W., Bucknall, D.G. & Morris, J.F. 2007 Temporally-resolved inkjet drop impaction on surfaces. AIChE J. 53 (10), 2606–2617.CrossRefGoogle Scholar
Duineveld, P.C. 2003 The stability of ink-jet printed lines of liquid with zero receding contact angle on a homogeneous substrate. J. Fluid Mech. 477, 175–200.CrossRefGoogle Scholar
Eggers, J., Fontelos, M.A., Josserand, C. & Zaleski, S. 2010 Drop dynamics after impact on a solid wall: theory and simulations. Phys. Fluids 22 (6), 62101.CrossRefGoogle Scholar
Feng, J.Q. 2015 Sessile drop deformations under an impinging jet. Theor. Comput. Fluid Dyn. 29 (4), 277–290.CrossRefGoogle Scholar
Furlani, E.P. 2015 Fluid mechanics for inkjet printing. In Fundamentals of Inkjet Printing (ed. S.D. Hoath), chap. 2, pp. 13–56. Wiley-VCH.CrossRefGoogle Scholar
Gunstensen, A.K., Rothman, D.H., Zaleski, S. & Zanetti, G. 1991 Lattice Boltzmann model of immiscible fuids. Phys. Rev. A 43 (8), 4320–4327.CrossRefGoogle Scholar
He, X., Chen, S. & Zhang, R. 1999 A lattice Boltzmann scheme for incompressible multiphase flow and its application in simulation of Rayleigh–Taylor instability. J. Comput. Phys. 152 (2), 642–663.CrossRefGoogle Scholar
Huang, H., Sukop, M. & Lu, X. 2015 Multiphase Lattice Boltzmann Methods: Theory and Application. John Wiley & Sons.CrossRefGoogle Scholar
Jackson, F.F., Kubiak, K.J., Wilson, M.C.T., Molinari, M. & Stetsyuk, V. 2019 Droplet misalignment limit for inkjet printing into cavities on textured surfaces. Langmuir 35 (29), 9564–9571.CrossRefGoogle ScholarPubMed
Josserand, C., Lemoyne, L., Troeger, R. & Zaleski, S. 2005 Droplet impact on a dry surface: triggering the splash with a small obstacle. J. Fluid Mech. 524, 47–56.CrossRefGoogle Scholar
Josserand, C. & Thoroddsen, S.T. 2016 Drop impact on a solid surface. Annu. Rev. Fluid Mech. 48 (1), 365–391.CrossRefGoogle Scholar
Kant, P., Hazel, A.L., Dowling, M., Thompson, A.B. & Juel, A. 2017 Controlling droplet spreading with topography. Phys. Rev. Fluids 2 (9), 094002.CrossRefGoogle Scholar
Kant, P., Hazel, A.L., Dowling, M., Thompson, A.B. & Juel, A. 2018 Sequential deposition of microdroplets on patterned surfaces. Soft Matt. 14, 8709–8716.CrossRefGoogle ScholarPubMed
Khojasteh, D., Kazerooni, M., Salarian, S. & Kamali, R. 2016 Droplet impact on superhydrophobic surfaces: a review of recent developments. J. Ind. Engng Chem. 42, 1–14.CrossRefGoogle Scholar
Krüger, T., Kuzmin, A., Kusumaatmaja, H., Shardt, O., Silva, G. & Viggen, E.M. 2016 The Lattice Boltzmann Method: Principles and Practice. Springer.Google Scholar
Kwon, J.-S., Lee, D.J. & Oh, J.H. 2018 Formation and characterization of inkjet-printed nanosilver lines on plasma-treated glass substrates. Appl. Sci. 8 (2), 280.CrossRefGoogle Scholar
Laan, N., De Bruin, K.G., Bartolo, D., Josserand, C. & Bonn, D. 2014 Maximum diameter of impacting liquid droplets. Phys. Rev. Appl. 2 (4), 044018.CrossRefGoogle Scholar
Lee, J.B., Laan, N., De Bruin, K.G., Skantzaris, G., Shahidzadeh, N., Derome, D., Carmeliet, J. & Bonn, D. 2015 Universal rescaling of drop impact on smooth and rough surfaces. J. Fluid Mech. 786, R4.CrossRefGoogle Scholar
Li, Q., Du, D.H., Fei, L.L. & Luo, K.H. 2019 Three-dimensional non-orthogonal MRT pseudopotential lattice Boltzmann model for multiphase flows. Comput. Fluids 186, 128–140.CrossRefGoogle Scholar
Li, Q., Luo, K.H., Kang, Q.J. & Chen, Q. 2014 Contact angles in the pseudopotential lattice Boltzmann modeling of wetting. Phys. Rev. E 90 (5), 53301.CrossRefGoogle ScholarPubMed
Li, Q., Luo, K.H. & Li, X.J. 2013 Lattice Boltzmann modeling of multiphase flows at large density ratio with an improved pseudopotential model. Phys. Rev. E 87 (5), 053301.CrossRefGoogle ScholarPubMed
Lim, T., Han, S., Chung, J., Chung, J.T., Ko, S. & Grigoropoulos, C.P. 2009 Experimental study on spreading and evaporation of inkjet printed pico-liter droplet on a heated substrate. Intl J. Heat Mass Transfer 52 (1–2), 431–441.CrossRefGoogle Scholar
Lucas, R. 1918 Ueber das Zeitgesetz des kapillaren Aufstiegs von Flüssigkeiten. Colloid Polym. Sci. 23 (1), 15–22.Google Scholar
Madejski, J. 1976 Solidification of droplets on a cold surface. Intl J. Heat Mass Transfer 19 (9), 1009– 1013.CrossRefGoogle Scholar
Mirjalili, S., Jain, S.S. & Dodd, M.S. 2017 Interface-capturing methods for two-phase flows: an overview and recent developments. Center Turbul. Res.: Annu. Res. Briefs 2017 (1), 117–135.Google Scholar
Nie, Z. & Kumacheva, E. 2008 Patterning surfaces with functional polymers. Nat. Mater. 7 (4), 277–290.CrossRefGoogle ScholarPubMed
Pasandideh-Fard, M., Qiao, Y.M., Chandra, S. & Mostaghimi, J. 1996 Capillary effects during droplet impact on a solid surface. Phys. Fluids 8 (3), 650–659.CrossRefGoogle Scholar
Range, K. & Feuillebois, F. 1998 Influence of surface roughness on liquid drop impact. J. Colloid Interface Sci. 203 (1), 16–30.CrossRefGoogle Scholar
Rashidian, H., Sellier, M. & Mandin, P. 2019 Dynamic wetting of an occlusion after droplet impact. Intl J. Multiphase Flow 111, 264–271.CrossRefGoogle Scholar
Rioboo, R., Marengo, M. & Tropea, C. 2002 Time evolution of liquid drop impact onto solid, dry surfaces. Exp. Fluids 33 (1), 112–124.CrossRefGoogle Scholar
Roisman, I.V. 2009 Inertia dominated drop collisions. II. An analytical solution of the Navier–Stokes equations for a spreading viscous film. Phys. Fluids 21 (5), 052104.CrossRefGoogle Scholar
Roisman, I.V., Prunet-Foch, B., Tropea, C. & Vignes-Adler, M. 2002 Multiple drop impact onto a dry solid substrate. J. Colloid Interface Sci. 256 (2), 396–410.CrossRefGoogle Scholar
Scheller, B.L. & Bousfield, D.W. 1995 Newtonian drop impact with a solid surface. AIChE J. 41 (6), 1357–1367.CrossRefGoogle Scholar
Seemann, R., Brinkmann, M., Kramer, E.J., Lange, F.F. & Lipowsky, R. 2005 Wetting morphologies at microstructured surfaces. Proc. Natl Acad. Sci. USA 102 (6), 1848–1852.CrossRefGoogle ScholarPubMed
Sekimot, K., Oguma, R. & Kawasaki, K. 1987 Morphological stability analysis of partial wetting. Ann. Phys. 176 (2), 359–392.CrossRefGoogle Scholar
Shan, X. & Chen, H. 1993 Lattice Boltzmann model for simulating flows with multi phases and components. Phys. Rev. E 47 (3), 1815–1819.CrossRefGoogle Scholar
Soltman, D.B. 2011 Understanding inkjet printed pattern generation. PhD thesis, University of California at Berkeley, CA.Google Scholar
Stringer, J. & Derby, B. 2010 Formation and stability of lines produced by inkjet printing. Langmuir 26 (12), 10365–10372.CrossRefGoogle ScholarPubMed
Swift, M.R., Osborn, W.R. & Yeomans, J.M. 1995 Lattice Boltzmann simulation of nonideal fluids. Phys. Rev. Lett. 75 (5), 830–833.CrossRefGoogle ScholarPubMed
Sykes, T.C., Castrejón-Pita, A.A., Castrejón-Pita, J.R., Harbottle, D., Khatir, Z., Thompson, H.M. & Wilson, M.C.T. 2020 a Surface jets and internal mixing during the coalescence of impacting and sessile droplets. Phys. Rev. Fluids 5 (2), 023602.CrossRefGoogle Scholar
Sykes, T.C., Harbottle, D., Khatir, Z., Thompson, H.M. & Wilson, M.C.T. 2020 b Substrate wettability influences internal jet formation and mixing during droplet coalescence. Langmuir 36 (32), 9596–9607.CrossRefGoogle ScholarPubMed
Thompson, A.B., Tipton, C.R., Juel, A., Hazel, A.L. & Dowling, M. 2014 Sequential deposition of overlapping droplets to form a liquid line. J. Fluid Mech. 761, 261–281.CrossRefGoogle Scholar
Ukiwe, C. & Kwok, D.Y. 2005 On the maximum spreading diameter of impacting droplets on well-prepared solid surfaces. Langmuir 21 (2), 666–673.CrossRefGoogle ScholarPubMed
Van Dam, D.B. & Le Clerc, C. 2004 Experimental study of the impact of an ink-jet printed droplet on a solid substrate. Phys. Fluids 16 (9), 3403–3414.CrossRefGoogle Scholar
Visser, C.W., Frommhold, P.E., Wildeman, S., Mettin, R., Lohse, D. & Sun, C. 2015 Dynamics of high-speed micro-drop impact: numerical simulations and experiments at frame-to-frame times below 100 ns. Soft Matt. 11 (9), 1708–1722.CrossRefGoogle ScholarPubMed
Visser, C.W., Tagawa, Y., Sun, C. & Lohse, D. 2012 Microdroplet impact at very high velocity. Soft Matt. 8 (41), 10732–10737.CrossRefGoogle Scholar
Vrancken, R.J., Kusumaatmaja, H., Hermans, K., Prenen, A.M., Pierre-Louis, O., Bastiaansen, C.W. & Broer, D.J. 2010 Fully reversible transition from Wenzel to Cassie-Baxter states on corrugated superhydrophobic surfaces. Langmuir 26 (5), 3335–3341.CrossRefGoogle ScholarPubMed
Wang, Y. & Bourouiba, L. 2018 Non-isolated drop impact on surfaces. J. Fluid Mech. 835, 24–44.CrossRefGoogle Scholar
Washburn, E.W. 1921 The dynamics of capillary flow. Phys. Rev. 17 (3), 273–283.CrossRefGoogle Scholar
Wildeman, S., Visser, C.W., Sun, C. & Lohse, D. 2016 On the spreading of impacting drops. J. Fluid Mech. 805, 636–655.CrossRefGoogle Scholar
Wilson, M.C.T. & Kubiak, K.J. 2016 Simulation of drops on surfaces. In Fundamentals of Inkjet Printing (ed. S.D. Hoath), chap. 11, pp. 281–312. Wiley-VCH.CrossRefGoogle Scholar
Yang, D., Krasowska, M., Priest, C., Popescu, M.N. & Ralston, J. 2011 Dynamics of capillary-driven flow in open microchannels. J. Phys. Chem. 115 (38), 18761–18769.Google Scholar
Yarin, A.L. 2006 Drop impact dynamics: splashing, spreading, receding, bouncing$\ldots$. Annu. Rev. Fluid Mech. 38 (1), 159–192.CrossRefGoogle Scholar
Yarin, A.L. & Weiss, D.A. 1995 Impact of drops on solid surfaces: self-similar capillary waves, and splashing as a new type of kinematic discontinuity. J. Fluid Mech. 283, 141–173.CrossRefGoogle Scholar
Yokoi, K., Vadillo, D., Hinch, J. & Hutchings, I.M. 2009 Numerical studies of the influence of the dynamic contact angle on a droplet impacting on a dry surface. Phys. Fluids 21 (7), 072102.CrossRefGoogle Scholar
Yuan, P. & Schaefer, L. 2006 Equations of state in a lattice Boltzmann model. Phys. Fluids 18 (4), 042101.CrossRefGoogle Scholar
Zhang, L., Cheng, X., Ku, T., Song, Y. & Zhang, D. 2018 Lattice Boltzmann study of successive droplets impingement on the non-ideal recessed microchannel for high-resolution features. Intl J. Heat Mass Transfer 120, 1085–1100.CrossRefGoogle Scholar

Al-Ghaithi et al. supplementary movie 1

Quasi-spherical cap morphology example.

Download Al-Ghaithi et al. supplementary movie 1(Video)
Video 5.2 MB

Al-Ghaithi et al. supplementary movie 2

Inertial morphology example.

Download Al-Ghaithi et al. supplementary movie 2(Video)
Video 4 MB

Al-Ghaithi et al. supplementary movie 3

Edge-pinned morphology example.

Download Al-Ghaithi et al. supplementary movie 3(Video)
Video 3.9 MB

Al-Ghaithi et al. supplementary movie 4

Semi-Imbibed morphology example.

Download Al-Ghaithi et al. supplementary movie 4(Video)
Video 4.3 MB

Al-Ghaithi et al. supplementary movie 5

Fully-Imbibed morphology example

Download Al-Ghaithi et al. supplementary movie 5(Video)
Video 2.8 MB

Al-Ghaithi et al. supplementary movie 6

Capillary morphology example. Capillary flow occurs after droplet deposition. The frame rate changes in the video because of the difference in frame rate between impact spreading and capillary flow.

Download Al-Ghaithi et al. supplementary movie 6(Video)
Video 5.7 MB

Al-Ghaithi et al. supplementary movie 7

Simulations of printing of consecutive droplets across scratches of two depths. The width and depths are chosen within the split semi-imbibed morphology range. Splitting occurs for the first depth as expected but consecutive droplets push back and cause re-coalescence. Splitting occurs and persists for the second depth.

Download Al-Ghaithi et al. supplementary movie 7(Video)
Video 14.5 MB

Al-Ghaithi et al. supplementary movie 8

Simulations of printing consecutive droplets along scratches in the edge-pinned range. The first droplet experiences the expected morphology but consecutive droplets cause overspill. Making the scratch deeper solves the over-spilling issue.

Download Al-Ghaithi et al. supplementary movie 8(Video)
Video 6.8 MB