Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-17T11:22:58.822Z Has data issue: false hasContentIssue false
  • English
  • Français

Monodisperse particle-laden exchange flows in a vertical duct

Published online by Cambridge University Press:  21 May 2018

N. Mirzaeian  and
K. Alba Open the ORCID record for K. Alba [Opens in a new window]
N. Mirzaeian
Affiliation:
Department of Engineering Technology, University of Houston, Houston, TX 77204, USA
K. Alba*
Affiliation:
Department of Engineering Technology, University of Houston, Houston, TX 77204, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study buoyancy-driven exchange flow of two mixtures in a vertical narrow duct (two-dimensional channel as well as pipe) theoretically. While the light mixture is assumed always to be a pure fluid, the heavy mixture can be selected as either a pure or a particle-laden fluid. A small width-to-length ratio considered for the duct (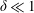$\unicode[STIX]{x1D6FF}\ll 1$) has been used as the perturbation parameter in developing a lubrication model (negligible inertia). In particular, we have adopted the methodology of Zhou et al. (Phys. Rev. Lett., vol. 94, 2005, 117803) for free-surface particle-laden film flows and extended it to a lock exchange system in confined geometry under the Boussinesq approximation. The resulting model is in the form of the classical Riemann problem and has been solved numerically using a robust total variation diminishing finite difference scheme. Both pure and particle-laden cases are investigated in detail. It is observed that the interface between the two fluids takes a self-similar shape at long times. In the case that both heavy and light fluids are pure, the dynamics of the flow is governed by two dimensionless quantities, namely the Reynolds number, $Re$, and the viscosity ratio, $\unicode[STIX]{x1D705}$, of the light and heavy fluids. The interpenetration of the heavy and light layers increases with 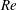$Re$ but decreases with $\unicode[STIX]{x1D705}$. Also, the heights of the heavy and light fronts change with $\unicode[STIX]{x1D705}$ but remain unchanged with $Re$. In the case of the particle-laden flow, however, four additional dimensionless parameters emerge, namely the initial volume fraction of particles, 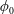$\unicode[STIX]{x1D719}_{0}$, the ratio of particle diameter to duct width, 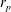$r_{p}$, and the density ratios of particles to carrying fluid, $\unicode[STIX]{x1D709}$, and of light fluid to carrying fluid, 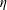$\unicode[STIX]{x1D702}$. The effect of these parameters on the dynamics of the flow has been quantified through a systematic approach. In the presence of solid particles, the interface between the heavy and light layers becomes more curved compared to the case of pure fluids. This modification occurs due to the change of heavy mixture viscosity alongside the duct. Novel particle-rich zones are further discovered in the vicinity of the advancing heavy and light fronts. These zones are associated with different transport rates of the fluid and solid particles. 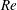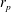The degree of particle enrichment remains the same with $Re$, is enhanced by $\unicode[STIX]{x1D705}$, $r_{p}$ and 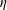$\unicode[STIX]{x1D702}$, and is slightly diminished with 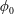$\unicode[STIX]{x1D719}_{0}$ and 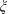$\unicode[STIX]{x1D709}$. 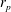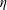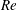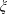On the other hand, the stretched exchange zone between the heavy and light fronts grows with $r_{p}$, $\unicode[STIX]{x1D702}$ and $Re$, but decays with $\unicode[STIX]{x1D719}_{0}$, $\unicode[STIX]{x1D705}$ and $\unicode[STIX]{x1D709}$.

JFM classification

Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 847 , 25 July 2018 , pp. 134 - 160
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Acrivos, A., Fan, X. & Mauri, R. 1994 On the measurement of the relative viscosity of suspensions. J. Rheol. 38 (5), 1285–1296.Google Scholar
Alba, K., Laure, P. & Khayat, R. E. 2011 Transient two-layer thin-film flow inside a channel. Phys. Rev. E 84, 026320.CrossRefGoogle ScholarPubMed
Alba, K., Taghavi, S. M. & Frigaard, I. A. 2012 Miscible density-stable displacement flows in inclined tube. Phys. Fluids 24, 123102.Google Scholar
Alba, K., Taghavi, S. M. & Frigaard, I. A. 2013a Miscible density-unstable displacement flows in inclined tube. Phys. Fluids 25, 067101.Google Scholar
Alba, K., Taghavi, S. M. & Frigaard, I. A. 2013b A weighted residual method for two-layer non-Newtonian channel flows: steady-state results and their stability. J. Fluid Mech. 731, 509–544.Google Scholar
Alba, K., Taghavi, S. M. & Frigaard, I. A. 2014 Miscible heavy-light displacement flows in an inclined two-dimensional channel: a numerical approach. Phys. Fluids 26 (12), 122104.CrossRefGoogle Scholar
Asomah, A. K. & Napier-Munn, T. J. 1997 An empirical model of hydrocyclones incorporating angle of cyclone inclination. Miner. Eng. 10 (3), 339–347.CrossRefGoogle Scholar
Auzerais, F. M., Jackson, R. & Russel, W. B. 1988 The resolution of shocks and the effects of compressible sediments in transient settling. J. Fluid Mech. 195, 437–462.Google Scholar
Baird, M. H. I., Aravamudan, K., Rao, N. V. R., Chadam, J. & Peirce, A. P. 1992 Unsteady axial mixing by natural convection in vertical column. AIChE J. 38, 1825–1834.CrossRefGoogle Scholar
Benjamin, T. B. 1968 Gravity currents and related phenomena. J. Fluid Mech. 31, 209–248.CrossRefGoogle Scholar
Birman, V. K., Battandier, B. A., Meiburg, E. & Linden, P. F. 2007 Lock-exchange flows in sloping channels. J. Fluid Mech. 577, 53–77.Google Scholar
Boateng, A. A. 2015 Rotary Kilns: Transport Phenomena and Transport Processes. Butterworth–Heinemann.Google Scholar
Bonnecaze, R. T., Huppert, H. E. & Lister, J. R. 1993 Particle-driven gravity currents. J. Fluid Mech. 250, 339–369.Google Scholar
Borden, Z. & Meiburg, E. 2013 Circulation-based models for Boussinesq internal bores. J. Fluid Mech. 726, R1.Google Scholar
Boycott, A. E. 1920 Sedimentation of blood corpuscles. Nature 104, 532.Google Scholar
Cook, B. P. 2008 Theory for particle settling and shear-induced migration in thin-film liquid flow. Phys. Rev. E 78 (4), 045303.Google Scholar
Cook, B. P., Alexandrov, O. & Bertozzi, A. L. 2009 Linear stability of particle-laden thin films. Eur. Phys. J. Spec. Top. 166 (1), 77–81.CrossRefGoogle Scholar
Cook, B. P., Bertozzi, A. L. & Hosoi, A. E. 2008 Shock solutions for particle-laden thin films. SIAM J. Appl. Maths 68 (3), 760–783.CrossRefGoogle Scholar
Davies, R. M. & Taylor, G. I. 1950 The mechanics of large bubbles rising through extended liquids and through liquids in a tube. Proc. R. Soc. Lond. A 200, 375.Google Scholar
Davis, R. H., Herbolzheimer, E. & Acrivos, A. 1983 Wave formation and growth during sedimentation in narrow tilted channels. Phys. Fluids 26 (8), 2055–2064.CrossRefGoogle Scholar
Debacq, M., Fanguet, V., Hulin, J. P., Salin, D. & Perrin, B. 2001 Self-similar concentration profiles in buoyant mixing of miscible fluids in a vertical tube. Phys. Fluids 13, 3097–3100.CrossRefGoogle Scholar
Espín, L. & Kumar, S. 2014 Forced spreading of films and droplets of colloidal suspensions. J. Fluid Mech. 742, 495–519.CrossRefGoogle Scholar
Greenspan, H. P. 1978 On the motion of a small viscous droplet that wets a surface. J. Fluid Mech. 84 (1), 125–143.CrossRefGoogle Scholar
Grunewald, N., Levy, R., Mata, M., Ward, T. & Bertozzi, A. L. 2010 Self-similarity in particle-laden flows at constant volume. J. Engng Maths 66 (1–3), 53–63.CrossRefGoogle Scholar
Hallez, Y. & Magnaudet, J. 2015 Buoyancy-induced turbulence in a tilted pipe. J. Fluid Mech. 762, 435–477.Google Scholar
Hamed, A. M. 2005 Experimental investigation on the adsorption/desorption processes using solid desiccant in an inclined-fluidized bed. Renew. Energy 30 (12), 1913–1921.Google Scholar
Hasnain, A. & Alba, K. 2017 Buoyant displacement flow of immiscible fluids in inclined ducts: a theoretical approach. Phys. Fluids 29, 052102.CrossRefGoogle Scholar
Kerswell, R. R. 2011 Exchange flow of two immiscible fluids and the principle of maximum flux. J. Fluid Mech. 682, 132–159.Google Scholar
Kurganov, A. & Tadmor, E. 2000 New high-resolution central schemes for nonlinear conservation laws and convection–diffusion equations. J. Comput. Phys. 160 (1), 241–282.CrossRefGoogle Scholar
Lyon, M. K. & Leal, L. G. 1998 An experimental study of the motion of concentrated suspensions in two-dimensional channel flow. Part 1. Monodisperse systems. J. Fluid Mech. 363, 25–56.Google Scholar
Martin, J., Rakotomalala, N., Talon, L. & Salin, D. 2011 Viscous lock-exchange in rectangular channels. J. Fluid Mech. 673, 132–146.Google Scholar
Mata, M. R. & Bertozzi, A. L. 2011 A numerical scheme for particle-laden thin film flow in two dimensions. J. Comput. Phys. 230 (16), 6334–6353.Google Scholar
Matson, G. P. & Hogg, A. J. 2012 Viscous exchange flows. Phys. Fluids 24 (2), 023102.CrossRefGoogle Scholar
Mavromoustaki, A. & Bertozzi, A. L. 2014 Hyperbolic systems of conservation laws in gravity-driven, particle-laden thin-film flows. J. Engng Maths 88 (1), 29–48.Google Scholar
Meiburg, E. & Kneller, B. 2010 Turbidity currents and their deposits. Annu. Rev. Fluid Mech. 42, 135–156.Google Scholar
Metzger, B., Guazzelli, É. & Butler, J. E. 2005 Large-scale streamers in the sedimentation of a dilute fiber suspension. Phys. Rev. Lett. 95 (16), 164506.Google Scholar
Murisic, N., Ho, J., Hu, V., Latterman, P., Koch, T., Lin, K., Mata, M. & Bertozzi, A. L. 2011 Particle-laden viscous thin-film flows on an incline: experiments compared with a theory based on shear-induced migration and particle settling. Physica D 240 (20), 1661–1673.Google Scholar
Murisic, N., Pausader, B., Peschka, D. & Bertozzi, A. L. 2013 Dynamics of particle settling and resuspension in viscous liquid films. J. Fluid Mech. 717, 203–231.Google Scholar
Nelson, E. B. & Guillot, D. 2006 Well Cementing, 2nd edn. Schlumberger Educational Services.Google Scholar
Petitjeans, P. & Maxworthy, T. 1996 Miscible displacements in capillary tubes. Part 1. Experiments. J. Fluid Mech. 326, 37–56.Google Scholar
Phillips, R. J., Armstrong, R. C., Brown, R. A., Graham, A. L. & Abbott, J. R. 1992 A constitutive equation for concentrated suspensions that accounts for shear-induced particle migration. Phys. Fluids 4 (1), 30–40.Google Scholar
Pratt, H. R. C. & Baird, M. H. I. 1983 Axial dispersion. In Handbook of Solvent Extraction (ed. Lo, T. C., Baird, M. H. I. & Hanson, C.). Wiley.Google Scholar
Richardson, J. F. & Zaki, W. N. 1954 The sedimentation of a suspension of uniform spheres under conditions of viscous flow. Chem. Engng Sci. 3 (2), 65–73.CrossRefGoogle Scholar
Saha, S., Salin, D. & Talon, L. 2013 Low Reynolds number suspension gravity currents. Eur. Phys. J. E 36, 85.Google ScholarPubMed
Sahu, K. C. & Vanka, S. P. 2011 A multiphase lattice Boltzmann study of buoyancy-induced mixing in a tilted channel. Comput. Fluids 50 (1), 199–215.Google Scholar
Schaflinger, U., Acrivos, A. & Zhang, K. 1990 Viscous resuspension of a sediment within a laminar and stratified flow. Intl J. Multiphase Flow 16 (4), 567–578.CrossRefGoogle Scholar
Sebilleau, F., Issa, R. I. & Walker, S. P. 2016 Analysis of turbulence modelling approaches to simulate single-phase buoyancy driven counter-current flow in a tilted tube. Flow Turbul. Combust. 96 (1), 95–132.CrossRefGoogle Scholar
Segre, G. & Silberberg, A. 1961 Radial particle displacements in Poiseuille flow of suspensions. Nature 189, 209–210.Google Scholar
Seon, T., Hulin, J.-P., Salin, D., Perrin, B. & Hinch, E. J. 2005 Buoyancy driven miscible front dynamics in tilted tubes. Phys. Fluids 17, 031702.CrossRefGoogle Scholar
Seon, T., Hulin, J.-P., Salin, D., Perrin, B. & Hinch, E. J. 2006 Laser-induced fluorescence measurements of buoyancy driven mixing in tilted tubes. Phys. Fluids 18, 041701.CrossRefGoogle Scholar
Seon, T., Znaien, J., Salin, D., Hulin, J.-P., Hinch, E. J. & Perrin, B. 2007a Front dynamics and macroscopic diffusion in buoyant mixing in a tilted tube. Phys. Fluids 19, 125105.Google Scholar
Seon, T., Znaien, J., Salin, D., Hulin, J.-P., Hinch, E. J. & Perrin, B. 2007b Transient buoyancy-driven front dynamics in nearly horizontal tubes. Phys. Fluids 19, 123603.CrossRefGoogle Scholar
Shin, J. O., Dalziel, S. B. & Linden, P. F. 2004 Gravity currents produced by lock exchange. J. Fluid Mech. 521, 1–34.CrossRefGoogle Scholar
Spaid, M. A. & Homsy, G. M. 1996 Stability of Newtonian and viscoelastic dynamic contact lines. Phys. Fluids 8 (2), 460–478.Google Scholar
Taghavi, S. M., Alba, K. & Frigaard, I. A. 2012a Buoyant miscible displacement flows at moderate viscosity ratios and low Atwood numbers in near-horizontal ducts. Chem. Engng Sci. 69, 404–418.Google Scholar
Taghavi, S. M., Alba, K., Seon, T., Wielage-Burchard, K., Martinez, D. M. & Frigaard, I. A. 2012b Miscible displacement flows in near-horizontal ducts at low Atwood number. J. Fluid Mech. 696, 175–214.CrossRefGoogle Scholar
Taghavi, S. M., Seon, T., Martinez, D. M. & Frigaard, I. A. 2009 Buoyancy-dominated displacement flows in near-horizontal channels: the viscous limit. J. Fluid Mech. 639, 1–35.CrossRefGoogle Scholar
Taghavi, S. M., Seon, T., Wielage-Burchard, K., Martinez, D. M. & Frigaard, I. A. 2011 Stationary residual layers in buoyant Newtonian displacement flows. Phys. Fluids 23, 044105.Google Scholar
Wang, L. & Bertozzi, A. L. 2014 Shock solutions for high concentration particle-laden thin films. SIAM J. Appl. Maths 74 (2), 322–344.Google Scholar
Wang, L., Mavromoustaki, A., Bertozzi, A. L., Urdaneta, G. & Huang, K. 2015 Rarefaction-singular shock dynamics for conserved volume gravity driven particle-laden thin film. Phys. Fluids 27 (3), 033301.CrossRefGoogle Scholar
Wiklund, J. A., Stading, M., Pettersson, A. J. & Rasmuson, A. 2006 A comparative study of UVP and LDA techniques for pulp suspensions in pipe flow. AIChE J. 52 (2), 484–495.CrossRefGoogle Scholar
Wong, J. T. & Bertozzi, A. L. 2016 A conservation law model for bidensity suspensions on an incline. Physica D 330, 47–57.Google Scholar
Zheng, Z., Rongy, L. & Stone, H. A. 2015 Viscous fluid injection into a confined channel. Phys. Fluids 27 (6), 062105.CrossRefGoogle Scholar
Zhou, J., Dupuy, B., Bertozzi, A. L. & Hosoi, A. E. 2005 Theory for shock dynamics in particle-laden thin films. Phys. Rev. Lett. 94 (11), 117803.CrossRefGoogle ScholarPubMed
Znaien, J., Moisy, F. & Hulin, J.-P. 2011 Flow structure and momentum transport for buoyancy driven mixing flows in long tubes at different tilt angles. Phys. Fluids 23, 035105.CrossRefGoogle Scholar