Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Crawford, Alice M.
Stunder, Barbara J. B.
Ngan, Fong
and
Pavolonis, Michael J.
2016.
Initializing HYSPLIT with satellite observations of volcanic ash: A case study of the 2008 Kasatochi eruption.
Journal of Geophysical Research: Atmospheres,
Vol. 121,
Issue. 18,
Pouget, Solène
Bursik, Marcus
Johnson, Christopher G.
Hogg, Andrew J.
Phillips, Jeremy C.
and
Sparks, R. Stephen J.
2016.
Interpretation of umbrella cloud growth and morphology: implications for flow regimes of short-lived and long-lived eruptions.
Bulletin of Volcanology,
Vol. 78,
Issue. 1,
Costa, A.
Suzuki, Y.J.
Cerminara, M.
Devenish, B.J.
Ongaro, T. Esposti
Herzog, M.
Van Eaton, A.R.
Denby, L.C.
Bursik, M.
de' Michieli Vitturi, M.
Engwell, S.
Neri, A.
Barsotti, S.
Folch, A.
Macedonio, G.
Girault, F.
Carazzo, G.
Tait, S.
Kaminski, E.
Mastin, L.G.
Woodhouse, M.J.
Phillips, J.C.
Hogg, A.J.
Degruyter, W.
and
Bonadonna, C.
2016.
Results of the eruptive column model inter-comparison study.
Journal of Volcanology and Geothermal Research,
Vol. 326,
Issue. ,
p.
2.
Sutherland, Bruce R.
and
Hong, Youn Sub (Dominic)
2016.
Sedimentation from particle-bearing plumes in a stratified ambient.
Physical Review Fluids,
Vol. 1,
Issue. 7,
Ungarish, M.
Johnson, C.G.
and
Hogg, A.J.
2016.
Sustained axisymmetric intrusions in a rotating system.
European Journal of Mechanics - B/Fluids,
Vol. 56,
Issue. ,
p.
110.
Prata, Fred
Woodhouse, Mark
Huppert, Herbert E.
Prata, Andrew
Thordarson, Thor
and
Carn, Simon
2017.
Atmospheric processes affecting the separation of volcanic ash and SO2 in volcanic eruptions: inferences from the May 2011 Grímsvötn eruption.
Atmospheric Chemistry and Physics,
Vol. 17,
Issue. 17,
p.
10709.
Kavanagh, Janine L.
Engwell, Samantha L.
and
Martin, Simon A.
2018.
A review of laboratory and numerical modelling in volcanology.
Solid Earth,
Vol. 9,
Issue. 2,
p.
531.
Ungarish, Marius
and
Hogg, Andrew J.
2018.
Models of internal jumps and the fronts of gravity currents: unifying two-layer theories and deriving new results.
Journal of Fluid Mechanics,
Vol. 846,
Issue. ,
p.
654.
Rossi, Eduardo
Bonadonna, Costanza
and
Degruyter, Wim
2019.
A new strategy for the estimation of plume height from clast dispersal in various atmospheric and eruptive conditions.
Earth and Planetary Science Letters,
Vol. 505,
Issue. ,
p.
1.
Ball, Thomasina V.
and
Huppert, Herbert E.
2019.
Similarity solutions and viscous gravity current adjustment times.
Journal of Fluid Mechanics,
Vol. 874,
Issue. ,
p.
285.
Webster, Helen N.
Devenish, Benjamin J.
Mastin, Larry G.
Thomson, David J.
and
Van Eaton, Alexa R.
2020.
Operational Modelling of Umbrella Cloud Growth in a Lagrangian Volcanic Ash Transport and Dispersion Model.
Atmosphere,
Vol. 11,
Issue. 2,
p.
200.
Bear-Crozier, Adele
Pouget, Solène
Bursik, Marcus
Jansons, Emile
Denman, Jarrad
Tupper, Andrew
and
Rustowicz, Rose
2020.
Automated detection and measurement of volcanic cloud growth: towards a robust estimate of mass flux, mass loading and eruption duration.
Natural Hazards,
Vol. 101,
Issue. 1,
p.
1.
Allen, P. A.
Dorrell, R. M.
Harlen, O. G.
Thomas, R. E.
and
McCaffrey, W. D.
2020.
Pulse propagation in gravity currents.
Physics of Fluids,
Vol. 32,
Issue. 1,
Wu, Ching-Sen
and
Dai, Albert
2020.
Experiments on two-layer stratified gravity currents in the slumping phase.
Journal of Hydraulic Research,
Vol. 58,
Issue. 5,
p.
831.
Mingotti, Nicola
and
Woods, Andrew W.
2020.
Stokes settling and particle-laden plumes: implications for deep-sea mining and volcanic eruption plumes.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 378,
Issue. 2179,
p.
20190532.
Skevington, Edward W. G.
Hogg, Andrew J.
and
Ungarish, Marius
2021.
Development of supercritical motion and internal jumps within lock-release radial currents and draining flows.
Physical Review Fluids,
Vol. 6,
Issue. 6,
de' Michieli Vitturi, Mattia
and
Pardini, Federica
2021.
PLUME-MoM-TSM 1.0.0: a volcanic column and umbrella cloud spreading model.
Geoscientific Model Development,
Vol. 14,
Issue. 3,
p.
1345.
Constantinescu, Robert
Hopulele-Gligor, Aurelian
Connor, Charles B.
Bonadonna, Costanza
Connor, Laura J.
Lindsay, Jan M.
Charbonnier, Sylvain
and
Volentik, Alain C. M.
2021.
The radius of the umbrella cloud helps characterize large explosive volcanic eruptions.
Communications Earth & Environment,
Vol. 2,
Issue. 1,
Pegler, Samuel S.
and
Ferguson, David J.
2021.
Rapid heat discharge during deep-sea eruptions generates megaplumes and disperses tephra.
Nature Communications,
Vol. 12,
Issue. 1,
Ishii, Kensuke
Nishijo, Akira
Koyaguchi, Takehiro
and
Suzuki, Yujiro J.
2022.
A physics-based source model for real-time tephra-dispersal forecasting for weak eruption plumes.
Journal of Applied Volcanology,
Vol. 11,
Issue. 1,
 $t$ as
$t$ as  $t^{3/4}$, rather than
$t^{3/4}$, rather than 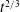 $t^{2/3}$ as suggested previously. We also identify a transition to drag-dominated flow, which is described by a similarity solution with radial growth now proportional to
$t^{2/3}$ as suggested previously. We also identify a transition to drag-dominated flow, which is described by a similarity solution with radial growth now proportional to 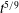 $t^{5/9}$. In the presence of an ambient wind, intrusions are not axisymmetric. Instead, they are predominantly advected downstream, while at the same time spreading laterally and thinning vertically due to persistent buoyancy forces. We show that close to the source, this lateral spreading is in a buoyancy-inertial regime, whereas far downwind, the horizontal buoyancy forces that drive the spreading are balanced by drag. Our results emphasise the important role of buoyancy-driven spreading, even at large distances from the source, in the formation of the flowing thin horizontally extensive layers of ash that form in the atmosphere as a result of volcanic eruptions.
$t^{5/9}$. In the presence of an ambient wind, intrusions are not axisymmetric. Instead, they are predominantly advected downstream, while at the same time spreading laterally and thinning vertically due to persistent buoyancy forces. We show that close to the source, this lateral spreading is in a buoyancy-inertial regime, whereas far downwind, the horizontal buoyancy forces that drive the spreading are balanced by drag. Our results emphasise the important role of buoyancy-driven spreading, even at large distances from the source, in the formation of the flowing thin horizontally extensive layers of ash that form in the atmosphere as a result of volcanic eruptions.


