Published online by Cambridge University Press: 20 January 2015
Transition to turbulence in shear flows is often subcritical, thus the dynamics of the flow strongly depends on the shape and amplitude of the perturbation of the laminar state. In the state space, initial perturbations which directly relaminarize are separated from those that go through a chaotic trajectory by a hypersurface having a very small number of unstable dimensions, known as the edge of chaos. Even for the simple case of plane Couette flow in a small domain, the edge of chaos is characterized by a fractal, folded structure. Thus, the problem of determining the threshold energy to trigger subcritical transition consists in finding the states on this complex hypersurface with minimal distance (in the energy norm) from the laminar state. In this work we have investigated the minimal-energy regions of the edge of chaos, by developing a minimization method looking for the minimal-energy perturbations capable of approaching the edge state (within a prescribed tolerance) in a finite target time 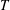 $T$. For sufficiently small target times, the value of the minimal energy has been found to vary with
$T$. For sufficiently small target times, the value of the minimal energy has been found to vary with 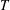 $T$ following a power law, whose best fit is given by
$T$ following a power law, whose best fit is given by 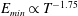 $E_{min}\propto T^{-1.75}$. For large values of
$E_{min}\propto T^{-1.75}$. For large values of  $T$, the minimal energy achieves a constant value which corresponds to the energy of the minimal seed, namely the perturbation of minimal energy asymptotically approaching the edge state (Rabin et al., J. Fluid Mech., vol. 738, 2012, R1). For
$T$, the minimal energy achieves a constant value which corresponds to the energy of the minimal seed, namely the perturbation of minimal energy asymptotically approaching the edge state (Rabin et al., J. Fluid Mech., vol. 738, 2012, R1). For 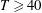 $T\geqslant 40$, all of the symmetries of the edge state are broken and the minimal perturbation appears to be localized in space with a basic structure composed of scattered patches of streamwise velocity with inclined streamwise vortices on their flanks. Finally, we have found that minimal perturbations originate in a small low-energy zone of the state space and follow very fast similar trajectories towards the edge state. Such trajectories are very different from those of linear optimal disturbances, which need much higher initial amplitudes to approach the edge state. The time evolution of these minimal perturbations represents the most efficient path to subcritical transition for Couette flow.
$T\geqslant 40$, all of the symmetries of the edge state are broken and the minimal perturbation appears to be localized in space with a basic structure composed of scattered patches of streamwise velocity with inclined streamwise vortices on their flanks. Finally, we have found that minimal perturbations originate in a small low-energy zone of the state space and follow very fast similar trajectories towards the edge state. Such trajectories are very different from those of linear optimal disturbances, which need much higher initial amplitudes to approach the edge state. The time evolution of these minimal perturbations represents the most efficient path to subcritical transition for Couette flow.