Hostname: page-component-745bb68f8f-l4dxg Total loading time: 0 Render date: 2025-01-10T03:41:41.777Z Has data issue: false hasContentIssue false
  • English
  • Français

Linear stability of compressible flow in a streamwise corner

Published online by Cambridge University Press:  04 November 2011

Oliver T. Schmidt  and
Ulrich Rist
Oliver T. Schmidt*
Affiliation:
Institut für Aerodynamik und Gasdynamik, Universität Stuttgart, Pfaffenwaldring 21, 70569 Stuttgart, Germany
Ulrich Rist
Affiliation:
Institut für Aerodynamik und Gasdynamik, Universität Stuttgart, Pfaffenwaldring 21, 70569 Stuttgart, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The linear stability of the compressible flow in a streamwise corner is studied. The steady laminar mean flow is obtained as a solution to the parabolized Navier–Stokes equations with the asymptotic cross-flow velocity enforced on the far-field boundaries via a sponge region and was found to be in good agreement with the corresponding self-similar solution. 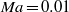The eigenvalue problem of linear stability theory is solved for base flows at three different Mach numbers representing the incompressible limit (), the subsonic (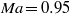) and the supersonic (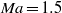) velocity regime. Particular attention is given to the subject of wave obliqueness. Owing to the break of periodicity by the opposing wall the exact spanwise wavenumbers and associated phase angles resolved in the computational domain are not known a priori. We address this problem by imposing different phase angles on the far-field boundaries to obtain the critical values of the Reynolds number, streamwise wavenumber and spanwise wavenumber from a three-dimensional solution space. The stability characteristics of the different types of modes present in the spectrum are discussed. An inviscid corner mode with odd-symmetry not present in the subsonic regime and with a higher amplification rate than its symmetric counterpart is identified in the supersonic case. Acoustic modes are found in an enlarged computational domain and categorized with respect to speed, symmetry and wall-boundedness, among them an acoustic corner mode.

JFM classification

Boundary Layers: Boundary layer stability Compressible Flows: Compressible boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 688 , 10 December 2011 , pp. 569 - 590
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Alizard, F., Robinet, J.-C. & Rist, U. 2010 Sensitivity analysis of a streamwise corner flow. Phys. Fluids 22 (1).CrossRefGoogle Scholar
2. Balachandar, S. & Malik, M. R. 1993 Inviscid instability of streamwise corner flow. NASA STI/Recon Technical Report Number 94.Google Scholar
3. Barclay, W. H. 1973 Experimental investigation of the laminar flow along a straight 135-deg corner. Aeronaut. Q. 24, 147–154.CrossRefGoogle Scholar
4. Barclay, W. H. & El-Gamal, H. A. 1983 Streamwise corner flow with wall suction. AIAA J. 21, 31–37.CrossRefGoogle Scholar
5. Barclay, W. H. & El-Gamal, H. A. 1984 Further solutions in streamwise corner flow with wall suction. AIAA J. 22, 1169–1171.CrossRefGoogle Scholar
6. Barclay, W. H. & Ridha, A. H. 1980 Flow in streamwise corners of arbitrary angle. AIAA J. 18, 1413–1420.CrossRefGoogle Scholar
7. Bodony, D. J. 2006 Analysis of sponge zones for computational fluid mechanics. J. Comput. Phys. 212, 681–702.CrossRefGoogle Scholar
8. Boyd, J. P. & Boyd, J. P. 2001 Chebyshev and Fourier Spectral Methods. Dover.Google Scholar
9. Canuto, C., Hussaini, M. Y., Quarteroni, A. & Zang, T. A. 1988 Spectral Methods in Fluid Dynamics. Springer.CrossRefGoogle Scholar
10. Carrier, G. 1947 The boundary layer in a corner. Q. Appl. Maths 4, 367–370.CrossRefGoogle Scholar
11. Davis, T. A. 2006 Direct Methods for Sparse Linear Systems. Society for Industrial Mathematics.CrossRefGoogle Scholar
12. Dhanak, M. R. 1992 Instability of flow in a streamwise corner. ICASE Report No. 92-70.Google Scholar
13. Dhanak, M. R. 1993 On the instability of flow in a streamwise corner. Proc. R. Soc. Lond. A 441, 201–210.Google Scholar
14. Duck, P. W. & Dhanak, M. R. 1996 The effects of free stream pressure gradient on a corner boundary layer. APS Meeting Abstracts.Google Scholar
15. El-Gamal, H. A. & Barclay, W. H. 1978 Experiments on the laminar flow in a rectangular streamwise corner. Aeronaut. Q. 29, 75–97.CrossRefGoogle Scholar
16. Fedorov, A. V. 2003 Receptivity of a high-speed boundary layer to acoustic disturbances. J. Fluid Mech. 491, 101–129.CrossRefGoogle Scholar
17. Galionis, I. & Hall, P. 2005 Spatial stability of the incompressible corner flow. Theor. Comput. Fluid Dyn. 19, 77–113.CrossRefGoogle Scholar
18. Ghia, K. N. 1975 Incompressible streamwise flow along an unbounded corner. AIAA J. 13, 902–907.CrossRefGoogle Scholar
19. Ghia, K. N. & Davis, R. T. 1974a A study of compressible potential and asymptotic viscous flows for corner region. AIAA J. 12, 355–359.CrossRefGoogle Scholar
20. Ghia, K. N. & Davis, R. T. 1974b Corner layer flow: optimization of numerical method of solution. Comput. Fluids 2, 17–34.CrossRefGoogle Scholar
21. Groskopf, G., Kloker, M. J. & Marxen, O. 2010 Bi-global crossplane stability analysis of high-speed boundary-layer flows with discrete roughness. In Seventh IUTAM Symposium on Laminar–Turbulent Transition (ed. P. Schlatter & D. S. Henningson), pp. 171.Google Scholar
22. Hanifi, A., Schmid, P. J. & Henningson, D. S. 1996 Transient growth in compressible boundary layer flow. Phys. Fluids 8, 826–837.CrossRefGoogle Scholar
23. Kreiss, H. O. & Oliger, J. 1972 Comparison of accurate methods for the integration of hyperbolic equations. Tellus 24 (3), 199–215.CrossRefGoogle Scholar
24. Lakin, W. D. & Hussaini, M. Y. 1984 Stability of the laminar boundary layer in a streamwise corner. Proc. R. Soc. Lond. A 393, 101–116.Google Scholar
25. Lehoucq, R. B., Sorensen, D. C. & Yang, C. 1998 ARPACK Users’ Guide: Solution of Large-Scale Eigenvalue Problems with Implicitly Restarted Arnoldi Methods. SIAM.CrossRefGoogle Scholar
26. Mack, L. M. 1984 Boundary-layer linear stability theory. In AGARD Special Course on the Stability and Transition of Laminar Flow.Google Scholar
27. Mikhail, A. G. & Ghia, K. N. 1978 Viscous compressible flow in the boundary region of an axial corner. AIAA J. 16, 931–939.CrossRefGoogle Scholar
28. Nomura, Y. 1962 Theoretical and experimental investigations on the incompressible viscous flow around the corner. Mem. Defence Acad. Japan 2, 115–145.Google Scholar
29. Nomura, Y. 1982 Analysis of a characteristic of laminar corner flow. AIAA J. 20, 1020–1022.CrossRefGoogle Scholar
30. Orszag, S. & Patterson, G. 1972 Numerical simulation of turbulence. Statist. Model Turbul. 127–147.CrossRefGoogle Scholar
31. Pal, A. & Rubin, S. G. 1971 Asymptotic features of viscous flow along a corner. Q. Appl. Maths 29, 91–108.CrossRefGoogle Scholar
32. Parker, S. J. & Balachandar, S. 1999 Viscous and inviscid instabilities of flow along a streamwise corner. Theor. Comput. Fluid Dyn. 13, 231–270.CrossRefGoogle Scholar
33. Ridha, A. 1992 On the dual solutions associated with boundary-layer equations in a corner. J. Engng Maths 26, 525–537.CrossRefGoogle Scholar
34. Ridha, A. 2002 Combined free and forced convection in a corner. Intl J. Heat Mass Transfer 45 (10), 2191–2205.CrossRefGoogle Scholar
35. Rubin, S. G. 1966 Incompressible flow along a corner. J. Fluid Mech. Digital Archive 26 (01), 97–110.CrossRefGoogle Scholar
36. Rubin, S. G. & Grossman, B. 1971 Viscous flow along a corner: numerical solution of the corner layer equations. Q. Appl. Maths 29, 169–186.CrossRefGoogle Scholar
37. Rubin, S. G. & Tannehill, J. C. 1992 Parabolized/reduced Navier–Stokes computational techniques. Annu. Rev. Fluid Mech. 24, 117–144.CrossRefGoogle Scholar
38. Schmid, P. J. & Henningson, D. S. 2001 Stability and Transition in Shear Flows. Springer.CrossRefGoogle Scholar
39. Tannehill, J., Anderson, D. & Pletcher, R. 1997 Computational Fluid Mechanics and Heat Transfer. Taylor & Francis.Google Scholar
40. Theofilis, V. 2003 Advances in global linear instability analysis of nonparallel and three-dimensional flows. Prog. Aeronaut. Sci. 39, 249–315.CrossRefGoogle Scholar
41. Trefethen, Lloyd N. 2000 Spectral Methods in MATLAB. Society for Industrial Mathematics.CrossRefGoogle Scholar
42. Weinberg, B. C. & Rubin, S. G. 1972 Compressible corner flow. J. Fluid Mech. 56, 753–774.CrossRefGoogle Scholar
43. Zamir, M. 1970 Boundary-layer theory and the flow in a streamwise corner (Flow equations for curvilinear boundary layer based on laminar incompressible boundary layer in streamwise corner). Aeronaut. J. 74, 330–332.CrossRefGoogle Scholar
44. Zamir, M. 1981 Similarity and stability of the laminar boundary layer in a streamwise corner. Proc. R. Soc. Lond. A 377, 269–288.Google Scholar
45. Zamir, M. & Young, A. D. 1970 Experimental investigation of the boundary layer in a streamwise corner. Aeronaut. Q. 30, 471–484.CrossRefGoogle Scholar
46. Zamir, M. & Young, A. D. 1979 Pressure gradient and leading edge effects on the corner boundary layer. Aeronaut. Q. 30, 471–483.CrossRefGoogle Scholar