Published online by Cambridge University Press: 06 January 2022
We revisit the somewhat classical problem of the linear stability of a rigidly rotating liquid column in this article. Although the literature pertaining to this problem dates back to 1959, the relation between inviscid and viscous stability criteria has not yet been clarified. While the viscous criterion for stability, given by  $We < n^2 + k^2 -1$, is both necessary and sufficient, this relation has only been shown to be sufficient in the inviscid case. Here,
$We < n^2 + k^2 -1$, is both necessary and sufficient, this relation has only been shown to be sufficient in the inviscid case. Here,  $We = \rho \varOmega ^2 a^3 / \gamma$ is the Weber number and measures the relative magnitudes of the centrifugal and surface tension forces, with
$We = \rho \varOmega ^2 a^3 / \gamma$ is the Weber number and measures the relative magnitudes of the centrifugal and surface tension forces, with  $\varOmega$ being the angular velocity of the rigidly rotating column,
$\varOmega$ being the angular velocity of the rigidly rotating column,  $a$ the column radius,
$a$ the column radius,  $\rho$ the density of the fluid and
$\rho$ the density of the fluid and  $\gamma$ the surface tension coefficient;
$\gamma$ the surface tension coefficient;  $k$ and
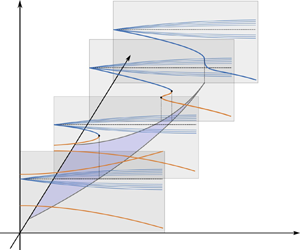
$k$ and  $n$ denote the axial and azimuthal wavenumbers of the imposed perturbation. We show that the subtle difference between the inviscid and viscous criteria arises from the surprisingly complicated picture of inviscid stability in the
$n$ denote the axial and azimuthal wavenumbers of the imposed perturbation. We show that the subtle difference between the inviscid and viscous criteria arises from the surprisingly complicated picture of inviscid stability in the  $We$–
$We$– $k$ plane. For all
$k$ plane. For all  $n > 1$, the viscously unstable region, corresponding to
$n > 1$, the viscously unstable region, corresponding to  $We > n^2 + k^2-1$, contains an infinite hierarchy of inviscidly stable islands ending in cusps, with a dominant leading island. Only the dominant island, now infinite in extent along the
$We > n^2 + k^2-1$, contains an infinite hierarchy of inviscidly stable islands ending in cusps, with a dominant leading island. Only the dominant island, now infinite in extent along the  $We$ axis, persists for
$We$ axis, persists for  $n=1$. This picture may be understood, based on the underlying eigenspectrum, as arising from the cascade of coalescences between a retrograde mode, that is the continuation of the cograde surface-tension-driven mode across the zero Doppler frequency point, and successive retrograde Coriolis modes constituting an infinite hierarchy.
$n=1$. This picture may be understood, based on the underlying eigenspectrum, as arising from the cascade of coalescences between a retrograde mode, that is the continuation of the cograde surface-tension-driven mode across the zero Doppler frequency point, and successive retrograde Coriolis modes constituting an infinite hierarchy.