Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-17T13:18:09.915Z Has data issue: false hasContentIssue false
  • English
  • Français

Large-eddy simulation of flow over a cylinder with 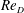$Re_{D}$ from 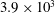$3.9\times 10^{3}$ to 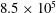$8.5\times 10^{5}$: a skin-friction perspective

Published online by Cambridge University Press:  05 May 2017

W. Cheng Open the ORCID record for W. Cheng [Opens in a new window] ,
D. I. Pullin ,
R. Samtaney ,
W. Zhang  and
W. Gao
W. Cheng*
Affiliation:
Mechanical Engineering, Physical Sciences and Engineering Division, King Abdullah University of Science and Technology, Thuwal, 23955-6900, Saudi Arabia Graduate Aerospace Laboratories, California Institute of Technology, CA 91125, USA
D. I. Pullin
Affiliation:
Graduate Aerospace Laboratories, California Institute of Technology, CA 91125, USA
R. Samtaney
Affiliation:
Mechanical Engineering, Physical Sciences and Engineering Division, King Abdullah University of Science and Technology, Thuwal, 23955-6900, Saudi Arabia
W. Zhang
Affiliation:
Mechanical Engineering, Physical Sciences and Engineering Division, King Abdullah University of Science and Technology, Thuwal, 23955-6900, Saudi Arabia
W. Gao
Affiliation:
Mechanical Engineering, Physical Sciences and Engineering Division, King Abdullah University of Science and Technology, Thuwal, 23955-6900, Saudi Arabia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present wall-resolved large-eddy simulations (LES) of flow over a smooth-wall circular cylinder up to 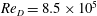$Re_{D}=8.5\times 10^{5}$, where $Re_{D}$ is Reynolds number based on the cylinder diameter 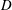$D$ and the free-stream speed 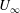$U_{\infty }$. The stretched-vortex subgrid-scale (SGS) model is used in the entire simulation domain. For the sub-critical regime, six cases are implemented with 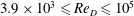$3.9\times 10^{3}\leqslant Re_{D}\leqslant 10^{5}$. Results are compared with experimental data for both the wall-pressure-coefficient distribution on the cylinder surface, which dominates the drag coefficient, and the skin-friction coefficient, which clearly correlates with the separation behaviour. In the super-critical regime, LES for three values of $Re_{D}$ are carried out at different resolutions. The drag-crisis phenomenon is well captured. For lower resolution, numerical discretization fluctuations are sufficient to stimulate transition, while for higher resolution, an applied boundary-layer perturbation is found to be necessary to stimulate transition. Large-eddy simulation results at 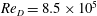$Re_{D}=8.5\times 10^{5}$, with a mesh of 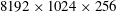$8192\times 1024\times 256$, agree well with the classic experimental measurements of Achenbach (J. Fluid Mech., vol. 34, 1968, pp. 625–639) especially for the skin-friction coefficient, where a spike is produced by the laminar–turbulent transition on the top of a prior separation bubble. We document the properties of the attached-flow boundary layer on the cylinder surface as these vary with 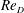$Re_{D}$. Within the separated portion of the flow, mean-flow separation–reattachment bubbles are observed at some values of $Re_{D}$, with separation characteristics that are consistent with experimental observations. Time sequences of instantaneous surface portraits of vector skin-friction trajectory fields indicate that the unsteady counterpart of a mean-flow separation–reattachment bubble corresponds to the formation of local flow-reattachment cells, visible as coherent bundles of diverging surface streamlines.

JFM classification

Boundary Layers: Boundary Layers Boundary Layers: Boundary layer separation Turbulent Flows: Turbulence simulation
Type
Papers
Information
Journal of Fluid Mechanics , Volume 820 , 10 June 2017 , pp. 121 - 158
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Achenbach, E. 1968 Distribuion of local pressure and skin friction around a circular cylinder in cross-flow up to Re = 5 × 106 . J. Fluid Mech. 34, 625–639.Google Scholar
Achenbach, E. & Heinecke, E. 1981 On vortex shedding from smooth and rough cylinders in the range of Reynolds numbers 6 × 103 to 5 × 106 . J. Fluid Mech. 109, 239–251.Google Scholar
Bearman, P. W. 1969 On vortex shedding from a circular cylinder in the critical Reynolds number regime. J. Fluid Mech. 37, 577–585.Google Scholar
Beaudan, P. & Moin, P.1994 Numerical experiments on the flow past a circular cylinder at sub-critical Reynolds number. Tech. Rep. TF-62. Stanford Univerisity.Google Scholar
Breuer, M. 2000 A challenging test case for large eddy simulation: high Reynolds number circular cylinder flow. Intl J. Heat Fluid Flow 21, 648–654.Google Scholar
Bursnall, W. J. & Loftin, L. K.1951 Experimental investigation of the pressure distribution about a yawed circular cylinder in the critical Reynolds number range. NACA Tech. Rep. TN-2463.Google Scholar
Cabot, W. & Moin, P. 1999 Approximate wall boundary conditions in the large-eddy simulation of high Reynolds number flow. Flow Turbul. Combust. 63, 269–291.Google Scholar
Cantwell, B. & Coles, D. 1983 An experimental study of entrainment and transport in the turbulent near wake of a circular cylinder. J. Fluid Mech. 136, 321–374.Google Scholar
Catalano, P., Wang, M., Iaccarino, G. & Moin, P. 2003 Numerical simulation of the flow around a circular cylinder at high Reynolds numbers. Intl J. Heat Fluid Flow 24, 463–469.Google Scholar
Chapman, D. R. 1979 Computational aerodynamics development and outlook. AIAA J. 17, 1293–1313.Google Scholar
Cheng, W., Pullin, D. I. & Samtaney, R. 2015 Large-eddy simulation of separation and reattachment of a flat plate turbulent boundary layer. J. Fluid Mech. 785, 78–108.Google Scholar
Choi, H. & Moin, P. 2012 Grid-point requirements for large eddy simulation: Chapman’s estimates revisited. Phys. Fluids 24, 011702.Google Scholar
Chong, M. S., Soria, J., Perry, A. E., Chacin, J., Cantwell, B. J. & Na, Y. 1998 Turbulence structures of wall-bounded shear flows found using DNS data. J. Fluid Mech. 357, 225–247.Google Scholar
Chung, D. & Pullin, D. I. 2009 Large-eddy simulation and wall modelling of turbulent channel flow. J. Fluid Mech. 631, 281–309.Google Scholar
Délery, J. M. 2001 Robert Legendre and Henri Werlé: toward the elucidation of three-dimensional separation. Annu. Rev. Fluid Mech. 33, 129–154.Google Scholar
Dong, S., Karniadakis, G. E., Ekmekci, A. & Rockwell, D. 2006 A combined direct numerical simulation–particle image velocimetry study of the turbulent near wake. J. Fluid Mech. 569, 185–207.Google Scholar
Fage, A. & Falkner, V. M.1931 The flow around a circular cylinder. Tech. Rep. 1369. Reports and Memoranda.Google Scholar
Fröhlich, J., Mellen, C. P., Rodi, W., Temmerman, L. & Leschziner, M. A. 2005 Highly resolved large-eddy simulation of separated flow in a channel with streamwise periodic constrictions. J. Fluid Mech. 526, 19–66.Google Scholar
Giedt, W. H. 1951 Effect of turbulence level of incident air stream on local heat transfer and skin friction on a cylinder. J. Aero. Sci. 18, 725–730.Google Scholar
Huai, X., Joslin, R. D. & Piomelli, U. 1997 Large-eddy simulation of transition to turbulence in boundary layers. Theor. Comput. Fluid Dyn. 9, 149–163.Google Scholar
Inoue, M. & Pullin, D. I. 2011 Large-eddy simulation of the zero-pressure-gradient turbulent boundary layer up to Re 𝜃 = O (1012). J. Fluid Mech. 686, 507–533.Google Scholar
Inoue, M., Pullin, D. I., Harun, Z. & Marusic, I. 2013 LES of the adverse-pressure gradient turbulent boundary layer. Intl J. Heat Fluid Flow 44, 293–300.Google Scholar
Kachanov, Y. S. & Levchenko, V. Y. 1984 The resonant interaction of disturbances at laminar–turbulent transition in a boundary layer. J. Fluid Mech. 138, 209–247.Google Scholar
Kravchenko, A. G. & Moin, P. 2000 Numerical studies of flow over a circular cylinder at Re D = 3900. Phys. Fluids 12, 403–417.Google Scholar
Lehmkuhl, O., Rodríguez, I., Borrell, R., Chiva, J. & Oliva, A. 2014 Unsteady forces on a circular cylinder at critical Reynolds numbers. Phys. Fluids 26, 125110.Google Scholar
Lourenco, L. M. & Shih, C.1994 Characteristics of the plane turbulent near wake of a circular cylinder, a particle image velocimetry study. In Numerical Experiments on the Flow Past a Circular Cylinder at Sub-Critical Reynolds Number by P. Beaudan & P. Moin. Tech. Rep. TF-62. Stanford Univerisity.Google Scholar
Lundgren, T. S. 1982 Strained spiral vortex model for turbulent fine structure. Phys. Fluids 25, 2193–2203.Google Scholar
Ma, X., Karamanos, G. S. & Karniadakis, G. E. 2000 Dynamics and low-dimensionality of a turbulent near wake. J. Fluid Mech. 410, 29–65.Google Scholar
Misra, A. & Pullin, D. I. 1997 A vortex-based subgrid stress model for large-eddy simulation. Phys. Fluids 9, 2443–2454.Google Scholar
Monty, J. P., Harun, Z. & Marusic, I. 2011 A parametric study of adverse pressure gradient turbulent boundary layers. Intl J. Heat Fluid Flow 32, 575–585.Google Scholar
Morinishi, Y., Lund, T. S., Vasilyev, O. V. & Moin, P. 1998 Fully conservative higher order finite difference schemes for incompressible flow. J. Comput. Phys. 143, 90–124.Google Scholar
Norberg, N. 1993 Pressure forces on a circular cylinder in cross flow. In IUTAM Symposium on Bluff-Body Wakes, Dynamics and Instabilities. Springer.Google Scholar
Parnaudeau, P., Carlier, J., Heitz, D. & Lamballais, H. 2008 Experimental and numerical studies of the flow over a circular cylinder at Reynolds number 3900. Phys. Fluids 20, 085101.Google Scholar
Perry, A. E. & Chong, M. S. 1987 A description of eddying motions and flow patterns using critical-point concepts. Annu. Rev. Fluid Mech. 19, 125–155.Google Scholar
Pfeil, H. & Orth, U. 1990 Boundary-layer transition on a cylinder with and without separation bubbles. Exp. Fluids 10, 23–32.Google Scholar
Prasad, A. & Williamson, C. H. K. 1997 The instability of the shear layer separating from a bluff body. J. Fluid Mech. 333, 375–402.Google Scholar
Roshko, A. 1961 Experiments on the flow past a circular cylinder at very high Reynolds number. J. Fluid Mech. 10, 345–356.Google Scholar
Roshko, A. 1993 Perspectives on bluff body aerodynamics. J. Wind Engng Ind. Aerodyn. 49, 79–100.Google Scholar
Saric, W. S. & Nayfeh, A. H. 1975 Nonparallel stability of boundary-layer flows. Phys. Fluids 18, 945–950.Google Scholar
Sayadi, T., Hamman, C. W. & Moin, P. 2013 Direct numerical simulation of complete H-type and K-type transitions with implications for the dynamics of turbulent boundary layers. J. Fluid Mech. 724, 480–509.Google Scholar
Schewe, G. 1983 On the force fluctuations acting on a circular cylinder in crossflow from subcritical up to transcritical Reynolds numbers. J. Fluid Mech. 133, 265–285.Google Scholar
Schewe, G. 1986 Sensitivity of transition phenomena to small perturbation in flow round a circular cylinder. J. Fluid Mech. 172, 33–46.Google Scholar
Shih, W. C. L., Wang, C., Coles, D. & Roshko, A. 1993 Experiments on flow past rough circular cylinders at large Reynolds numbers. J. Wind Engng Ind. Aerodyn. 49, 351–368.Google Scholar
Simens, M. P.2008 The study and control of wall bounded flows. PhD thesis, Universidad Politécnica de Madrid, Madrid.Google Scholar
Son, J. S. & Hanratty, T. J. 1969 Velocity gradients at the wall for flow around a cylinder at Reynolds numbers from 5 × 103 to 105 . J. Fluid Mech. 35, 353–368.Google Scholar
Szepessy, S. & Bearman, P. W. 1992 Aspect ratio and end plate effects on vortex shedding from a circular cylinder. J. Fluid Mech. 234, 191–217.Google Scholar
Travin, A., Shur, M., Strelets, M. & Spalart, P. 1999 Detached-eddy simlations past a circular cylinder. Flow Turbul. Combust. 63, 293–313.Google Scholar
Thom, A.1928 The boundary layer of the front portion of a cylinder. Tech. Rep. 1176. Reports and Memoranda.Google Scholar
Voelkl, T., Pullin, D. I. & Chan, D. C. 2000 A physical-space version of the stretched-vortex subgrid-stress model for large-eddy simulation. Phys. Fluids 12, 1810–1825.Google Scholar
Weidman, P.1968 Wave transition and blockage effects on cylinder base pressures. PhD thesis, California Institute of Technology.Google Scholar
Williamson, C. H. K. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid Mech. 28, 477–539.Google Scholar
Wu, X. & Moin, P. 2009 Direct numerical simulation of turbulence in a nominally zero-pressure-gradient flat-plate boundary layer. J. Fluid Mech. 630, 5–41.Google Scholar
You, D. & Moin, P. 2007 A dynamic global-coefficient subgrid-scale eddy-viscosity model for large-eddy simulation in complex geometries. Phys. Fluids 19, 065110.Google Scholar
Zang, Y., Street, R. L. & Koseff, J. R. 1994 A non-staggered grid, fractional step method for time-dependent incompressible Navier–Stokes equations in curvilinear coordinates. J. Comput. Phys. 114, 18–33.Google Scholar
Zhang, W., Cheng, W., Gao, W., Qamar, A. & Samtaney, R. 2015 Geometrical effects on the airfoil flow separation and transition. Comput. Fluids 15, 60–73.Google Scholar
Zhang, W. & Samtaney, R. 2016 Low-Re past an isolated cylinder with rounded corners. Comput. Fluids 136, 384–401.Google Scholar